Class 10 Maths Chapter 2 Practice Question Answers - Polynomials
Q1. Find the value of “p” from the polynomial x2 + 3x + p, if one of the zeroes of the polynomial is 2.
Sol:
As 2 is the zero of the polynomial.
We know that if α is a zero of the polynomial p(x), then p(α) = 0f
Substituting x = 2 in x2 + 3x + p,
⇒ 22 + 3(2) + p = 0
⇒ 4 + 6 + p = 0
⇒ 10 + p = 0
⇒ p = -10
Q2. Does the polynomial a4 + 4a2 + 5 have real zeroes?
Sol:
In the aforementioned polynomial, let a2 = x.
Now, the polynomial becomes,
x2 + 4x + 5
Comparing with ax2 + bx + c,
Here, b2 – 4ac = 42 – 4(1)(5) = 16 – 20 = -4
So, D = b2 – 4ac < 0
As the discriminant (D) is negative, the given polynomial does not have real roots or zeroes.
Q3. Compute the zeroes of the polynomial 4x2 – 4x – 8. Also, establish a relationship between the zeroes and coefficients.
Sol:
Let the given polynomial be p(x) = 4x2 – 4x – 8
To find the zeroes, take p(x) = 0
Now, factorise the equation 4x2 – 4x – 8 = 0
4x2 – 4x – 8 = 0
4(x2 – x – 2) = 0
x2 – x – 2 = 0
x2 – 2x + x – 2 = 0
x(x – 2) + 1(x – 2) = 0
(x – 2)(x + 1) = 0
x = 2, x = -1
So, the roots of 4x2 – 4x – 8 are -1 and 2.
Relation between the sum of zeroes and coefficients:
-1 + 2 = 1 = -(-4)/4 i.e. (- coefficient of x/ coefficient of x2)
Relation between the product of zeroes and coefficients:
(-1) × 2 = -2 = -8/4 i.e (constant/coefficient of x2)
Q4. Find the quadratic polynomial if its zeroes are 0, √5.
Sol:
A quadratic polynomial can be written using the sum and product of its zeroes as:
x2 – (α + β)x + αβ
Where α and β are the roots of the polynomial.
Here, α = 0 and β = √5
So, the polynomial will be:
x2 – (0 + √5)x + 0(√5)
= x2 – √5x
Q5. Find the value of “x” in the polynomial 2a2 + 2xa + 5a + 10 if (a + x) is one of its factors.
Sol:
Let f(a) = 2a2 + 2xa + 5a + 10
Since, (a + x) is a factor of 2a2 + 2xa + 5a + 10, f(-x) = 0
So, f(-x) = 2x2 – 2x2 – 5x + 10 = 0
-5x + 10 = 0
5x = 10
x = 10/5
Therefore, x = 2
Q6. How many zeros does the polynomial (x – 3)2 – 4 have? Also, find its zeroes.
Sol:
Given polynomial is (x – 3)2 – 4
Now, expand this expression.
=> x2 + 9 – 6x – 4
= x2 – 6x + 5
As the polynomial has a degree of 2, the number of zeroes will be 2.
Now, solve x2 – 6x + 5 = 0 to get the roots.
So, x2 – x – 5x + 5 = 0
=> x(x – 1) -5(x – 1) = 0
=> (x – 1)(x – 5) = 0
x = 1, x = 5
So, the roots are 1 and 5.
Q7. α and β are zeroes of the quadratic polynomial x2 – 6x + y. Find the value of ‘y’ if 3α + 2β = 20.
Sol:
Let, f(x) = x² – 6x + y
From the given,
3α + 2β = 20———————(i)
From f(x),
α + β = 6———————(ii)
And,
αβ = y———————(iii)
Multiply equation (ii) by 2. Then, subtract the whole equation from equation (i),
=> α = 20 – 12 = 8
Now, substitute this value in equation (ii),
=> β = 6 – 8 = -2
Substitute the values of α and β in equation (iii) to get the value of y, such as;
y = αβ = (8)(-2) = -16
Q8. If the zeroes of the polynomial x3 – 3x2 + x + 1 are a – b, a, a + b, then find the value of a and b.
Sol:
Let the given polynomial be:
p(x) = x3 – 3x2 + x + 1
Given,
The zeroes of the p(x) are a – b, a, and a + b.
Now, compare the given polynomial equation with general expression.
px3 + qx2 + rx + s = x3 – 3x2 + x + 1
Here, p = 1, q = -3, r = 1 and s = 1
For sum of zeroes:
Sum of zeroes will be = a – b + a + a + b
-q/p = 3a
Substitute the values q and p.
-(-3)/1 = 3a
a = 1
So, the zeroes are 1 – b, 1, 1 + b.
For the product of zeroes:
Product of zeroes = 1(1 – b)(1 + b)
-s/p = 1 – 𝑏2
=> -1/1 = 1 – 𝑏2
Or, 𝑏2 = 1 + 1 =2
So, b = √2
Thus, 1 – √2, 1, 1 + √2 are the zeroes of equation 𝑥3 − 3𝑥2 + 𝑥 + 1.
Q9. Find a quadratic polynomial each with the given numbers as the sum and product of its zeroes, respectively.
(i) 1/4, -1
(ii) 1, 1
(iii) 4, 1
Sol:
(i) From the formulas of sum and product of zeroes, we know,
Sum of zeroes = α + β
Product of zeroes = αβ
Given,
Sum of zeroes = 1/4
Product of zeroes = -1
Therefore, if α and β are zeroes of any quadratic polynomial, then the polynomial can be written as:-
x2 – (α + β)x + αβ
= x2 – (1/4)x + (-1)
= 4x2 – x – 4
Thus, 4x2 – x – 4 is the required quadratic polynomial.
(ii) Given,
Sum of zeroes = 1 = α + β
Product of zeroes = 1 = αβ
Therefore, if α and β are zeroes of any quadratic polynomial, then the polynomial can be written as:- x2 – (α + β)x + αβ
= x2 – x + 1
Thus, x2 – x + 1 is the quadratic polynomial.
(iii) Given,
Sum of zeroes, α + β = 4
Product of zeroes, αβ = 1
Therefore, if α and β are zeroes of any quadratic polynomial, then the polynomial can be written as:-
x. – (α + β)x + αβ
= x. – 4x + 1
Thus, x2 – 4x +1 is the quadratic polynomial.
Q10. Obtain all other zeroes of 3x4 + 6x3 – 2x2 – 10x – 5, if two of its zeroes are √(5/3) and-√(5/3).
Sol: Since this is a polynomial of degree 4, hence there will be a total of 4 roots.
√(5/3) and-√(5/3) are zeroes of polynomial f(x).
∴ [x-√(5/3)] [x+√(5/3)] = x2-(5/3)
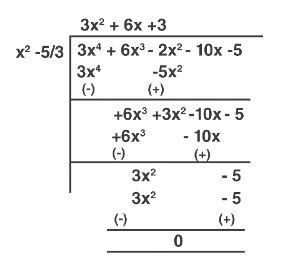
Therefore, 3x2 + 6x + 3 = 3x(x + 1) +3 (x + 1)
= (3x + 3)(x + 1)
= 3(x + 1)(x + 1)
= 3(x + 1)(x + 1)
Hence, x + 1 = 0 i.e. x = – 1 , – 1 is a zero of p(x).
So, its zeroes are given by: x = −1 and x = −1.
Therefore, all four zeroes of the given polynomial are:
√(5/3) and-√(5/3), −1 and −1.
Q11. Find a quadratic polynomial whose zeroes are reciprocals of the zeroes of the polynomial f(x) = ax2 + bx + c, a ≠ 0, c ≠ 0.
Sol:
Let α and β be the zeroes of the polynomial f(x) = ax2 + bx + c.
So, α + β = -b/a
αβ = c/a
According to the given, 1/α and 1/β are the zeroes of the required quadratic polynomial.
Now, the sum of zeroes = (1/α) + (1/β)
= (α + β)/αβ
= (-b/a)/ (c/a)
= -b/c
Product of two zeroes = (1/α) (1/β)
= 1/αβ
= 1/(c/a)
= a/c
The required quadratic polynomial = k[x2 – (sum of zeroes)x + (product of zeroes)]
= k[x2 – (-b/c)x + (a/c)]
= k[x2 + (b/c) + (a/c)]
Q12. Divide the polynomial f(x) = 3x2 – x3 – 3x + 5 by the polynomial g(x) = x – 1 – x2 and verify the division algorithm.
Sol:
Given,
f(x) = 3x2 – x3 – 3x + 5
g(x) = x – 1 – x2
Dividing f(x) = 3x2 – x3 – 3x + 5 by g(x) = x – 1 – x2
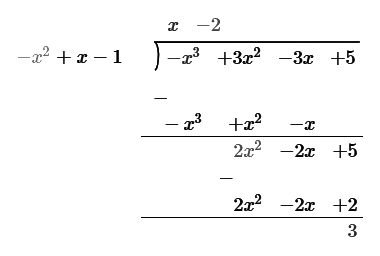
Here,
Quotient = q(x) = x – 2
Remainder = r(x) = 3
By division algorithm of polynomials,
Dividend = (Quotient × Divisor) + Remainder
So,
[q(x) × g(x)] + r(x) = (x – 2)(x – 1 – x2) + 3
= x2 – x – x3 – 2x + 2 + 2x2 + 3
= 3x2 – x3 – 3x + 5
= f(x)
Hence, the division algorithm is verified.
Q13. For what value of k, is the polynomial f(x) = 3x4 – 9x3 + x2 + 15x + k completely divisible by 3x2 – 5?
Sol:
Given,
f(x) = 3x4 – 9x3 + x2 + 15x + k
g(x) = 3x2 – 5
Dividing f(x) by g(x),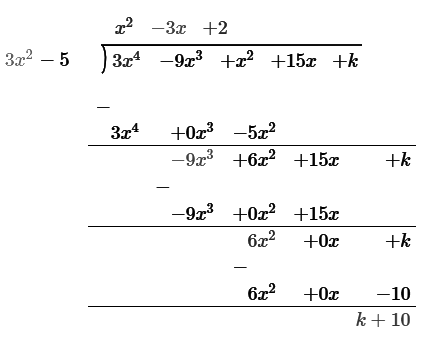
Given that f(x) is completely divisible by 3x2 – 5.
So, the remainder = 0
k + 10 = 0
k = -10
Q14. If 4 is a zero of the cubic polynomial x3 – 3x2 – 10x + 24, find its other two zeroes.
Sol:
Given cubic polynomial is p(x) = x3 – 3x2 – 10x + 24
4 is a zero of p(x).
So, (x – 4) is the factor of p(x).
Let us divide the given polynomial by (x – 4).
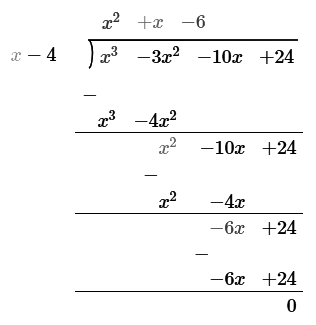
Here, the quotient = x2 + x – 6
= x2 + 3x – 2x – 6
= x(x + 3) – 2(x + 3)
= (x – 2)(x + 3)
Therefore, the other two zeroes of the given cubic polynomial are 2 and -3.
|
127 videos|584 docs|79 tests
|
















