Practice Questions: Coordinate Geometry

Q1. Find the distance of the point P (2, 3) from the x-axis.

Sol: 

We know that,

(x, y) = (2, 3) is a point on the Cartesian plane in the first quadrant.

x = Perpendicular distance from y-axis

y = Perpendicular distance from x-axis

Therefore, the perpendicular distance from x-axis = y coordinate = 3

Q 2. Find a relation between x and y such that the point (x, y) is equidistant from the points (7, 1) and (3, 5).

Sol: 

Let P(x, y) be equidistant from the points A(7, 1) and B(3, 5).

Then, AP = BP

AP² = BP²

Using distance formula,

(x - 7)² + (y - 1)² = (x - 3)² + (y - 5)²

x² - 14x + 49 + y² - 2y + 1 = x² - 6x + 9 + y² - 10y + 25

x - y = 2

Hence, the relation between x and y is x - y = 2.

Q.3. Find the coordinates of the points of trisection (i.e., points dividing into three equal parts) of the line segment joining the points A(2, - 2) and B(- 7, 4).

Sol:

Let P and Q be the points of trisection of AB, i.e., AP = PQ = QB.

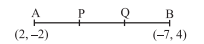
Therefore, P divides AB internally in the ratio 1: 2.

Let (x₁, y₁) = (2, -2)

(x₂, y₂) = (-7, 4)

m₁ : m₂ = 1 : 2

Therefore, the coordinates of P, by applying the section formula,

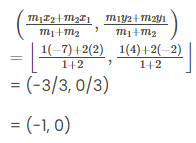
Similarly, Q also divides AB internally in the ratio 2 : 1. and the coordinates of Q by applying the section formula,

Hence, the coordinates of the points of trisection of the line segment joining A and B are (-1, 0) and (- 4, 2).

Q4. Find the ratio in which the line segment joining the points (- 3, 10) and (6, - 8) is divided by (- 1, 6).

Sol:

Let the ratio in which the line segment joining ( -3, 10) and (6, -8) is divided by point ( -1, 6) be k:1.

Therefore by section formula,

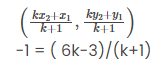
-k - 1 = 6k -3

7k = 2

k = 2/7

Hence, the required ratio is 2 : 7.

Q 5. Find the value of k if the points A(2, 3), B(4, k) and C(6, -3) are collinear.

Sol:

Given,

A(2, 3)= (x₁, y₁)

B(4, k) = (x₂, y₂)

C(6, -3) = (x₃, y₃)

If the given points are collinear, the area of the triangle formed by them will be 0.

½ [x₁(y₂ - y₃) + x₂(y₃ - y₁) + x₃(y₁ - y₂)] = 0

½ [2(k + 3) + 4(-3 -3) + 6(3 - k)] = 0

½ [2k + 6 - 24 + 18 - 6k] = 0

½ (-4k) = 0

4k = 0

k = 0

Q6. Find the area of the triangle formed by joining the mid-points of the sides of the triangle whose vertices are (0, -1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle.

Sol:

Let the vertices of the triangle be A(0, -1), B(2, 1) and C(0, 3).

Let D, E, F be the mid-points of the sides of this triangle.

Using the mid-point formula, coordinates of D, E, and F are:

D = [(0+2)/2, (-1+1)/2] = (1, 0)

E = [(0+0)/2, (-1+3)/2] = (0, 1)

F = [(0+2)/2, (3+1)/2] = (1, 2)

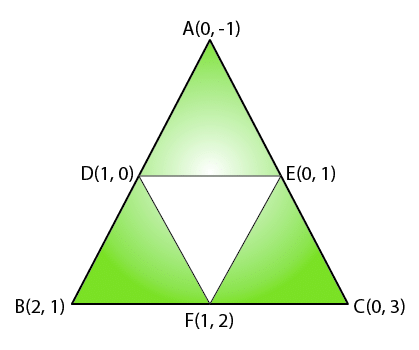
We know that,

Area of triangle = ½ [x₁(y₂ - y₃) + x₂(y₃ - y₁) + x₃(y₁ - y₂)]

Area of triangle DEF = ½ {(1(2 - 1) + 1(1 - 0) + 0(0 - 2)}

= ½ (1 + 1)

= 1

Area of triangle DEF = 1 sq.unit

Area of triangle ABC = ½ {0(1 - 3) + 2(3 - (-1)) + 0(-1 - 1)}

= ½ (8)

= 4

Area of triangle ABC = 4 sq.units

Hence, the ratio of the area of triangle DEF and ABC = 1 : 4.

Q7. Name the type of triangle formed by the points A (-5, 6), B (-4, -2) and C (7, 5).

Sol: 

The points are A (-5, 6), B (-4, -2) and C (7, 5).

Using distance formula,

d = √ ((x₂ - x₁)² + (y₂ - y₁)²)

AB = √((-4+5)² + (-2-6)²)

= √(1+64)

=√65

BC=√((7+4)² + (5+2)²)

=√(121 + 49)

=√170

AC=√((7+5)² + (5-6)²)

=√144 + 1

=√145

Since all sides are of different lengths, ABC is a scalene triangle.

Q8. Find the area of triangle PQR formed by the points P(-5, 7), Q(-4, -5) and R(4, 5).

Sol: 

Given,

P(-5, 7), Q(-4, -5) and R(4, 5)

Let P(-5, 7) = (x₁, y₁)

Q(-4, -5) = (x₂, y₂)

R(4, 5) = (x₃, y₃)

Area of the triangle PQR = (½)|x₁(y₂ - y₃) + x₂(y₃ - y₁) + x₃(y₁ - y₂)|

= (½) |-5(-5 - 5) + (-4)(5 - 7) + 4(7 + 5)|

= (½) |-5(-10) -4(-2) + 4(12)|

= (½) |50 + 8 + 48|

= (½) × 106

= 53

Therefore, the area of triangle PQR is 53 sq. units.

Q9. If the point C(-1, 2) divides internally the line segment joining A(2, 5) and B(x, y) in the ratio 3 : 4, find the coordinates of B.

Sol:

Given,

C(-1, 2) divides internally the line segment joining A(2, 5) and B(x, y) in the ratio 3 : 4.

Here,

A(2, 5) = (x₁, y₁) 

B(x, y) = (x₂, y₂)

m : n = 3 : 4

Using section formula,

C(-1, 2) = [(mx₂ + nx₁)/(m + n), (my₂ + ny₁)/(m + n)]

= [(3x + 8)/(3 + 4), (3y + 20)/(3 + 7)]

By equating the corresponding coordinates,

(3x + 8)/7 = -1

3x + 8 = -7

3x = -7 - 8

3x = -15

x = -5

And

(3y + 20)/7 = 2

3y + 20 = 14

3y = 14 - 20

3y = -6

y = -2

Therefore, the coordinates of B(x, y) = (-5, -2).

Q10. Find the ratio in which the line x - 3y = 0 divides the line segment joining the points (-2, -5) and (6, 3). Find the coordinates of the point of intersection.

Sol:

Let the given points be:

A(-2, -5) = (x₁, y₁)

B(6, 3) = (x₂, y₂)

The line x - 3y = 0 divides the line segment joining the points A and B in the ratio k : 1.

Using section formula,

Point of division P(x, y) = [(kx₂ + x₁)/(k + 1), (ky₂ + y₁)/(k + 1)]

x = (6k - 2)/(k + 1) and y = (3k - 5)/(k + 1)

Here, the point of division lies on the line x - 3y = 0.

Thus,

[(6k - 2)/(k + 1)] - 3[(3k - 5)/(k + 1)] = 0

6k - 2 - 3(3k - 5) = 0

6k - 2 - 9k + 15 = 0

-3k + 13 = 0

-3k = -13

k = 13/3

Thus, the ratio in which the line x - 3y = 0 divides the line segment AB is 13 : 3.

Therefore, x = [6(13/3) - 2]/ [(13/3) + 1]

= (78 - 6)/(13 + 3)

= 72/16

= 9/2

And

y = [3(13/3) - 5]/ [(13/3) + 1]

= (39 - 15)/(13 + 3)

= 24/16

= 3/2

Therefore, the coordinates of the point of intersection = (9/2, 3/2).

Q11. Write the coordinates of a point on the x-axis which is equidistant from points A(-2, 0) and B(6, 0).

Sol:

Let P(x, 0) be a point on the x-axis.

Given that point, P is equidistant from points A(-2, 0) and B(6, 0).

AP = BP

Squaring on both sides,

(AP)² = (BP)²

Using distance formula,

(x + 2)² + (0 - 0)² = (x - 6)² + (0 - 0)²

x² + 4x + 4 = x² - 12x + 36

4x + 12x = 36 - 4

16x = 32

x = 2

Therefore, the coordinates of a point on the x-axis = (2, 0).

Q12. If A(-2, 1), B(a, 0), C(4, b) and D(1, 2) are the vertices of a parallelogram ABCD, find the values of a and b. Hence, find the lengths of its sides.

Sol:

Given vertices of a parallelogram ABCD are:

A(-2, 1), B(a, 0), C(4, b) and D(1, 2)

We know that the diagonals of a parallelogram bisect each other.

So, midpoint of AC = midpoint of BD

[(-2 + 4)/2, (1 + b)/2] = [(a + 1)/2, (0 + 2)/2]

By equating the corresponding coordinates,

2/2 = (a + 1)/2 and (1 + b)/2 = 2/2

a + 1 = 2 and b + 1 = 2

a = 1 and b = 1

Therefore, a = 1 and b = 1.

Let us find the lengths of sides of a parallelogram, i.e. AB, BC, CD and DA

Using the distance formula,

AB = √[(1 + 2)² + (0 - 1)²] = √(9 + 1) = √10 units

BC = √[(4 - 1)² + (1 - 0)²] = √(9 + 1) = √10 units

And CD = √10 and DA = √10 {the opposite sides of a parallelogram are parallel and equal}

Hence, the length of each side of the parallelogram ABCD = √10 units.

Q.13: If A(-5, 7), B(-4, -5), C(-1, -6) and D(4, 5) are the vertices of a quadrilateral, find the area of the quadrilateral ABCD.

Sol: 

Given vertices of a quadrilateral are:

A(-5, 7), B(-4, -5), C(-1, -6) and D(4, 5)

The quadrilateral ABCD can be divided into two triangles ABD and BCD.

Area of the triangle with vertices (x₁, y₁), (x₂, y₂), and (x₃, y₃) = (½) |x₁(y₂ - y₃) + x₂(y₃ - y₁) + x₃(y₁ - y₂)|

Area of triangle ABD = (½) |-5(-5 - 5) + (-4)(5 - 7) + 4(7 + 5)|

= (½) |-5(-10) -4(-2) + 4(12)|

= (½) |50 + 8 + 48|

= (½) × 106

= 53

Area of triangle BCD = (½) |-4(-6 - 5) + (-1)(5 + 5) + 4(-5 + 6)|

= (½) |-4(-11) -1(10) + 4(1)|

= (½) |44 - 10 + 4|

= (½) × 38

= 19 

Therefore, the area of quadrilateral ABCD = Area of triangle ABD + Area of triangle BCD

= 53 + 19

= 72 sq.units

Q14. Find the ratio in which P(4, m) divides the line segment joining the points A(2, 3) and B(6, -3). Hence, find m.

Sol:

Let P(4, m) divides the line segment joining the points A(2, 3) and B(6, -3) in the ratio k : 1.

Here,

P(4, m) = (x, y)

A(2, 3) = (x₁, y₁)

B(6, -3) = (x₂, y₂)

Using section formula,

p(x, y) = [(kx₂ + x₁)/(k + 1), (ky₂ + y₁)/(k + 1)]

(4, m) = [(6k + 2)/(k + 1), (-3k + 3)/(k + 1)]

By equating the x-coodinate,

(6k + 2)/(k + 1) = 4

6k + 2 = 4k + 4

6k - 4k = 4 - 2

2k = 2

k = 1

Thus, the point P divides the line segment joining A and B in the ratio 1 : 1.

Now by equating the y-coodinate,

(-3k + 3)/(k + 1) = m

Substituting k = 1,

[-3(1) + 3]/(1 + 1) = m

m = (3 - 3)/2

m = 0

Q15. Find the distance of a point P(x, y) from the origin.

Sol:

Given,

P(x, y)

Coordinates of origin = O(0, 0)

Let P(x, y) = (x₁, y₁)

O(0, 0) = (x₂, y₂)

Using distance formula,

OP = √[(x2 - x1)² + (y2 - y1)²]

= √[(x - 0)² + (y - 0)²]

= √(x² + y²)

Hence, the distance of the point P(x, y) from the origin is √(x² + y²) units.