Class 10 Maths Chapter 11 HOTS Questions - Area Related to Circles
Q1: Find the area of the shaded region in given Fig. where arcs drawn with centres A, B, C and D intersect in pairs at mid-points P, Q, R and S of the sides AB, BC, CD and DA, respectively of a square ABCD (Use π = 3.14).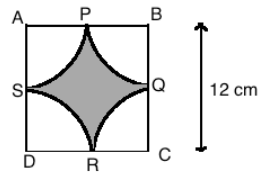 (a) 30.96 cm2
(a) 30.96 cm2
(b) 90.96 cm2
(c) 120.96 cm2
(d) 180.96 cm2
Ans: (a)
Area of all four sectors made by square and circle are equal.
And each sector have an angle of 90º
And radius of circle is 12/2 cm = 6 cm
Area of shaded region = Area of square ABCD − 4 × Area of one sector
⇒ Area of shaded region = Area of square ABCD − Area of circle with radius 6 cm
= 12 × 12 − π × 62 cm2
=144 − 113.04 cm2 = 30.96 cm2
Q2: PQRS is the diameter of a circle of radius 6 cm. The lengths PQ, QR and RS are equal. Semi-circles are drawn with PQ and QS as diameters as shown in figure, the ratio of the area of the shaded region to that of the unshaded region is 5:1. If true then enter 1 and if false then enter 0.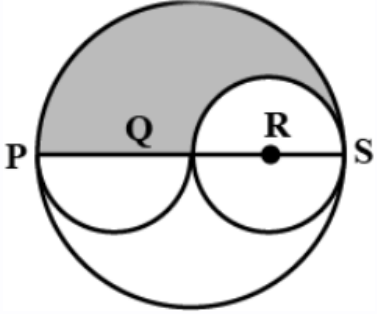 Ans: Area of circle = πr2 = 36π
Ans: Area of circle = πr2 = 36π
PQ = QR = RS = 2cm
Thus answer is false. 0
Q3: In figure ABC is a right-angled triangle right-angled at A. Semicircle are drawn on AB, AC and BC as diameters. Find the area of the shaded region.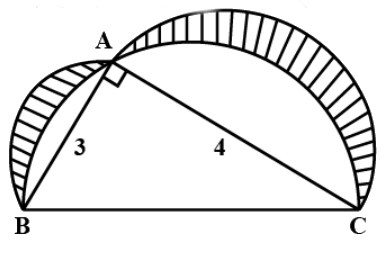
(a) 6sq. unit
(b) 9sq. unit
(c) 8sq. unit
(d) 5sq. unit
Ans: (a)
Area of shaded region = area of semicircle AB + area of semi-circle AC - (area of semicircle on side BC) + area of ΔABC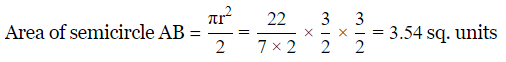
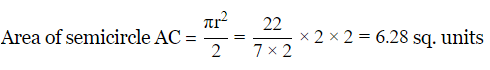
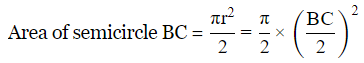
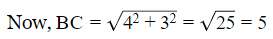

Area ΔABC= 1/2 × AB × AC = 1/2 × 3 × 4 = 6 sq. units
Therefore, area of shaded region = 3.54 + 6.28 − 9.82 + 6
= 6 sq. units
Q4: In a circular table corner of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as shown in Fig. Find the area of the design.
Ans: In △BOD By Trigonometry,
BD = 32sin 60o = 27.71cm
BC = 55.43cm
OD = 32 cos 60o
=16cm
∴ Area of △ BOC = 1/2 × 16 × 55.43
= 443.4cm2
∴ Area of Equilateral triangle = 3 × A△BOC = 3 × 443.4 =1330.2cm2
Area of circle = π × r2 =3.14 × 32 × 32 = 3215.36cm2
∴ Area of shaded region = 3215.36 − 1330.2 = 1885.16cm2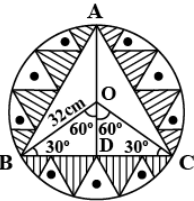
Q5: Two semi-circles are drawn on the diameter of a semi-circle with radius 18cm. A circle with centre C is drawn such that it touches the two semi-circles. Find area of shaded region.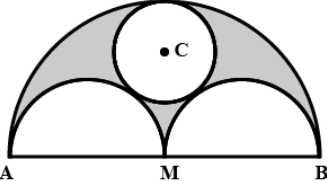
Ans: Draw RM ⊥ AB
AM = MB = AB/2 =18 cm
AP = PM = MQ = QB = 18/2 = 9 cm
MR = AM = 18㎝
CM = RM − CR = 18 − r
PC = PE + EC = 9 + r
In triangle CMP
PC2 = CM2 + PM2 ⇒ (9+r)2 = (18−r)2 + 92
⇒ 81 + 18r + r2 = 324 − 36r + r2 + 81 ⇒ 54r = 324 ⇒ r = 6 cm
∴ Area of shaded region=area of semi-circle AB−2 area of semi-circle-area of circle with C as centre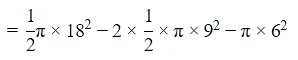
=162π − 81π − 36π = 45π cm2
|
126 videos|457 docs|75 tests
|

|
Explore Courses for Class 10 exam
|

|












