Class 8 Maths Chapter 5 Practice Question Answers - Squares and Square Roots
Q1: Write a Pythagorean triplet whose one member is:
(i) 6
(ii) 14
(iii) 16
(iv) 18
Sol:
We know, for any natural number m, 2m, m2 – 1, m2 + 1 is a Pythagorean triplet.
(i) 2m = 6
⇒ m = 6/2 = 3
m2 – 1= 32 – 1 = 9 – 1 = 8
m2 + 1= 32 + 1 = 9 + 1 = 10
Therefore, (6, 8, 10) is a Pythagorean triplet.
(ii) 2m = 14
⇒ m = 14/2 = 7
m2 – 1= 72 – 1 = 49 – 1 = 48
m2 + 1 = 72 + 1 = 49 + 1 = 50
Therefore, (14, 48, 50) is not a Pythagorean triplet.
(iii) 2m = 16
⇒ m = 16/2 = 8
m2 – 1 = 82 – 1 = 64 – 1 = 63
m2 + 1 = 82 + 1 = 64 + 1 = 65
Therefore, (16, 63, 65) is a Pythagorean triplet.
(iv) 2m = 18
⇒ m = 18/2 = 9
m2–1 = 92 – 1 = 81 – 1 = 80
m2 + 1 = 92 + 1 = 81 + 1 = 82
Therefore, (18, 80, 82) is a Pythagorean triplet.
Q2: Show that 121 is the sum of 11 odd natural numbers.
Sol: As 121 = 112
We know that the sum of first n odd natural numbers is n2.
Therefore, 121 = sum of first 11 odd natural numbers
= 1 + 3 + 5+ 7 + 9 + 11 +13 + 15 + 17 + 19 + 21
Q3: Use the identity and find the square of 189.
(a – b)2 = a2 – 2ab + b2
Sol: 189 = (200 – 11)2
= 40000 – 2 x 200 x 11 + 112
= 40000 – 4400 + 121
= 35721
Q4: Find the square roots of 100 and 169 by the method of repeated subtraction.
Sol:
Let us find the square root of 100 first.
100 – 1 = 99
99 – 3 = 96
96 – 5 = 91
91 – 7 = 84
84 – 9 = 75
75 – 11 = 64
64 – 13 = 51
51 – 15 = 36
36 – 17 = 19
19 – 19 = 0
Here, we have performed a subtraction ten times.
Therefore, √100 = 10
Now, the square root of 169:
169 – 1 = 168
168 – 3 = 165
165 – 5 = 160
160 – 7 = 153
153 – 9 = 144
144 – 11 = 133
133 – 13 = 120
120 – 15 = 105
105 – 17 = 88
88 – 19 = 69
69 – 21 = 48
48 – 23 = 25
25 – 25 = 0
Here, we have performed subtraction thirteen times.
Therefore, √169 = 13
Q5: Find the smallest whole number by which 1008 should be multiplied so as to get a perfect square number. Also, find the square root of the square number so obtained.
Sol:
Let us factorise the number 1008.
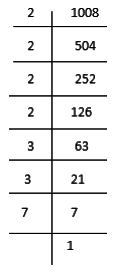
1008 = 2 × 2 × 2 × 2 × 3 × 3 × 7
= (2 × 2) × (2 × 2) × (3 × 3) × 7
Here, 7 cannot be paired.
Therefore, we will multiply 1008 by 7 to get a perfect square.
New number so obtained = 1008 × 7 = 7056
Now, let us find the square root of 7056
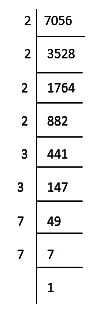
7056 = 2 × 2 × 2× 2 × 3 × 3 × 7 × 7
⇒ 7056 = (2 × 2) × (2 × 2) × (3 × 3) × (7 × 7)
⇒ 7056 = 22 × 22 × 32 × 72
⇒ 7056 = (2 × 2 × 3 × 7)2
Therefore;
⇒ √7056 = 2 × 2 × 3 × 7 = 84
Q6: 2025 plants are to be planted in a garden in such a way that each row contains as many plants as the number of rows. Find the number of rows and the number of plants in each row.
Sol:
Let the number of rows be, x.
Therefore, the number of plants in each row = x.
Total many contributed by all the students = x×x = x2
Given, x2 = Rs.2025
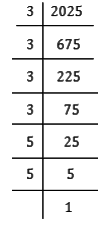
x2 = 3 × 3 × 3 × 3 × 5 × 5
⇒ x2 = (3 × 3) × (3 × 3) × (5 × 5)
⇒ x2 = (3 × 3 × 5) × (3 × 3 × 5)
⇒ x2 = 45 × 45
⇒ x = √(45 × 45)
⇒ x = 45
Therefore,
Number of rows = 45
Number of plants in each rows = 45
Q7: Find the square root of 7921 using long division method.
Sol:

∴ √7921 = 89
Q8: Find the least number which must be added to 1750 so as to get a perfect square. Also, find the square root of the obtained number.
Sol:
Using long division method:
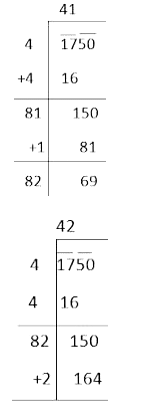
Here, (41)2 < 1750 > (42)2
We can say 1750 is ( 164 – 150 ) = 14 less than (42)2.
Therefore, if we add 14 to 1750, it will be a perfect square.
New number = 1750 + 14 = 1764
Therefore, the square root of 1764 is as follows:
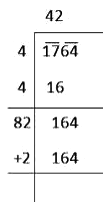 ∴√1764 = 42
∴√1764 = 42
Q9: Find the square root of 42.25 using long division method.
Sol:
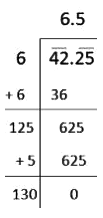 ∴ √42.25 = 6.5
∴ √42.25 = 6.5
Q10: Find the smallest square number that is divisible by each of the numbers 8, 15 and 20.
Sol:
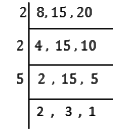
L.C.M of 8, 15 and 20 is (2 × 2 × 5 × 2 × 3) = 120.
120 = 2 × 2 × 3 × 5 × 2 = (2 × 2) × 3 × 5 × 2
Here, 3, 5 and 2 cannot be paired.
Therefore, we need to multiply 120 by (3 × 5 × 2) i.e. 30 to get a perfect square.
Hence, the smallest squared number which is divisible by numbers 8, 15 and 20 = 120 × 30 = 3600
Q11: Find the smallest whole number by which 2800 should be divided so as to get a perfect square.
Sol:
Let us first factorise the number 2800.
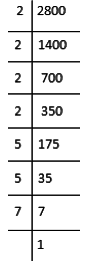
2800 = 2 × 2 × 2 × 2 × 5 × 5 × 7
= (2 × 2) × (2 × 2) × (5 × 5) × 7
Here, 7 cannot be paired.
Therefore, we will divide 2800 by 7 to get a perfect square.
New number = 2800 ÷ 7 = 400
Q12: Find the square root of 729 using factorisation method.
Sol:
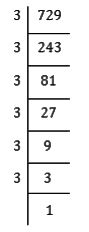
729 = 3 × 3 × 3 × 3 × 3 × 3 × 1
⇒ 729 = (3 × 3) × (3 × 3) × (3 × 3)
⇒ 729 = (3 × 3 × 3) × (3 × 3 × 3)
⇒ 729 = (3 × 3 × 3)2
Therefore,
⇒ √729 = 3 × 3 × 3 = 27
Q13: What would be the square root of 625 using the identity (a + b)2 = a2 + b2 + 2ab?
Sol: (625)2
= (600 + 25)2
= 6002 + 2 x 600 x 25 +252
= 360000 + 30000 + 625
= 390625
Q14: Show that the sum of two consecutive natural numbers is 132.
Sol:
Let 2n + 1 = 13
So, n = 6
So, ( 2n + 1)2 = 4n2 + 4n + 1
= (2n2 + 2n) + (2n2 + 2n + 1)
Substitute n = 6,
(13)2 = ( 2 x 62 + 2 x 6) + (2 x 62 + 2 x 6 + 1)
= (72 + 12) + (72 + 12 + 1)
= 84 + 85
Q15: (n + 1)2 - n2 = ?
Sol:
(n + 1)2 - n2
= (n2 + 2n + 1) – n2
= 2n + 1
Q16: How many numbers lie between squares of the following numbers?
(i) 12 and 13
(ii) 25 and 26
(iii) 99 and 100
Sol: As we know, between n2 and (n + 1)2, the number of non–perfect square numbers are 2n.
(i) Between 122 and 132 there are 2 × 12 = 24 natural numbers.
(ii) Between 252 and 262 there are 2 × 25 = 50 natural numbers.
(iii) Between 992 and 1002 there are 2 × 99 =198 natural numbers.
|
81 videos|423 docs|31 tests
|
















