Class 5 Exam > Class 5 Notes > Mathematics for Class 5: NCERT > Important Questions: How Big, How Heavy
Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy
Q1: Find the number of cubes in the base layer of the following figure: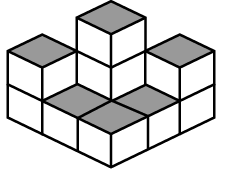
Ans: The number of cubes in the base layer of the given figure = 6
Q2: Count the number of cubes in the given shape
Ans: Total cubes in given figure are :11
Q3: Count the number of cubes in the given shape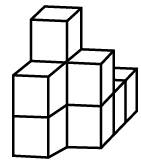
Ans: Total cubes in given figure are: 9
Q4: Count the number of cubes in the given shape.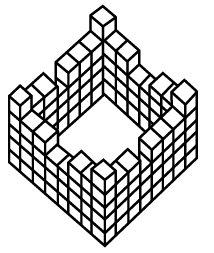
Ans: Total cubes in given figure are :110
Q5: Count the number of cubes in the given shape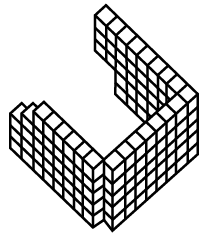
Ans: Total cubes in given figure are :113
Q6: Solid spheres of diameter 6 cm are dropped into a cylindrical beaker containing some water and are fully submerged. If the diameter of the beaker is 18cm and the water rises by 40cm, find the number of solid spheres dropped in the water.
Ans: By volume conservation we can say that the amount of water displaced by solid sphere is equal to the volume of spheres being dropped inside the cylindrical beaker.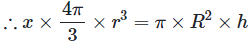
where x is the number of spheres
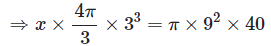where r = 3 and R = 9
On solving,
x = 90
Q7: How many lead cubes of side 2 cm could be made from a lead cube of side 8 cm?
Ans: Let x be the number of lead cubes of side 2 cm
Since all the smaller lead cubes are formed from the bigger lead cube of side 8 cm
⟹ Number of smaller lead cubes × volume of each lead cube = volume of bigger lead cube
⟹ x × (2)3 = (8)3 [∵ Volume of cube of edge length a is a3]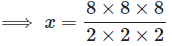
⟹x = 64
∴ 64 smaller lead cubes can be made form the bigger lead cube
Q8: Count the number of cubes and find the volume in cu. cm.
(Let the side of each cube = a)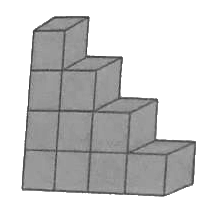
Ans: We observe that the number of cubes present = 10
Given: Side of each cube = a
Total volume = 10 × a3
=10a3
Q9: Count the number of cubes in the given shape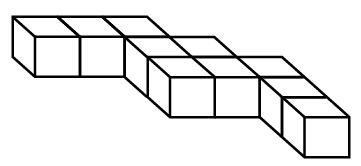
Ans: Total cubes in given figure are :10
Q10: Metal spheres, each of radius 2 cm, are packed into a rectangular box of internal dimensions 16cm × 8cm × 8cm. When 16 spheres are packed the box is filled with preservative liquid. Find the volume of this liquid (in cm3). Give your answer to the nearest integer. [Use π = 3.14]
Ans: Volume of rectangular box = lbh = 16 (64) = 1024 cm3
Volume of sphere = 4/3 πr3 = 33.5238 cm3
16 sphere = 16 (33.5238) = 536.3808
Volume of liquid = 1024 − 536.3808 = 488 cm3
The document Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy is a part of the Class 5 Course Mathematics for Class 5: NCERT.
All you need of Class 5 at this link: Class 5
|
28 videos|169 docs|41 tests
|
FAQs on Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy
| 1. How big is a Class 5 vehicle and what are its weight limitations? |  |
Ans. A Class 5 vehicle typically refers to a medium-duty truck with a Gross Vehicle Weight Rating (GVWR) ranging from 16,001 to 19,500 pounds. The weight limitations may vary depending on the specific regulations of different regions, but generally, Class 5 vehicles are designed to carry loads up to their designated GVWR.
| 2. What are the common uses for Class 5 trucks? | |
Ans. Class 5 trucks are commonly used for various purposes such as delivery trucks, utility trucks, box trucks, flatbed trucks, tow trucks, and small dump trucks. Their versatility makes them suitable for a wide range of industries including transportation, construction, and logistics.
| 3. Can a regular driver's license be used to operate a Class 5 vehicle? | |
Ans. In many regions, a regular driver's license is sufficient to operate a Class 5 vehicle. However, it is essential to check the specific requirements of your local jurisdiction as some places may require a commercial driver's license (CDL) or additional endorsements based on the vehicle's weight or nature of the cargo being transported.
| 4. What are the advantages of Class 5 trucks over other classes? | |
Ans. Class 5 trucks offer several advantages compared to other classes. They provide a balance between size and maneuverability, making them suitable for urban environments. Class 5 trucks also have a higher payload capacity compared to lighter classes, allowing for more substantial loads to be transported. Additionally, their cost of ownership and operation is generally lower compared to heavier commercial vehicles.
| 5. Are there any specific safety regulations or requirements for Class 5 vehicles? | |
Ans. Yes, there are specific safety regulations and requirements for Class 5 vehicles. These may include mandatory inspections, maintenance schedules, weight restrictions, and adherence to safety equipment standards. It is crucial for operators to familiarize themselves with the safety regulations in their region and ensure compliance to ensure the safe operation of Class 5 vehicles.
Related Exams
About this Document
|
Dec 18, 2024 Last updated |
Document Description: Important Questions: How Big, How Heavy for Class 5 2024 is part of Mathematics for Class 5: NCERT preparation.
The notes and questions for Important Questions: How Big, How Heavy have been prepared according to the Class 5 exam syllabus. Information about Important Questions: How Big, How Heavy covers topics
like and Important Questions: How Big, How Heavy Example, for Class 5 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Important Questions: How Big, How Heavy.
Introduction of Important Questions: How Big, How Heavy in English is available as part of our Mathematics for Class 5: NCERT
for Class 5 & Important Questions: How Big, How Heavy in Hindi for Mathematics for Class 5: NCERT course.
Download more important topics related with notes, lectures and mock test series for Class 5
Exam by signing up for free. Class 5: Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy
Description
Full syllabus notes, lecture & questions for Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy - Class 5 | Plus excerises question with solution to help you revise complete syllabus for Mathematics for Class 5: NCERT | Best notes, free PDF download
Information about Important Questions: How Big, How Heavy
In this doc you can find the meaning of Important Questions: How Big, How Heavy defined & explained in the simplest way possible. Besides explaining types of
Important Questions: How Big, How Heavy theory, EduRev gives you an ample number of questions to practice Important Questions: How Big, How Heavy tests, examples and also practice Class 5
tests
|
Explore Courses for Class 5 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy
,mock tests for examination
,Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy
,shortcuts and tricks
,ppt
,MCQs
,Important questions
,Semester Notes
,Exam
,Previous Year Questions with Solutions
,Objective type Questions
,video lectures
,Free
,Extra Questions
,Sample Paper
,past year papers
,Viva Questions
,Summary
,practice quizzes
,study material
,Class 5 Maths Chapter 14 Important Question Answers - Chapter 14 - How Big How Heavy
;
Additional Information about Important Questions: How Big, How Heavy for Class 5 Preparation
Important Questions: How Big, How Heavy Free PDF Download
The Important Questions: How Big, How Heavy is an invaluable resource that delves deep into the core of the Class 5 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Important Questions: How Big, How Heavy now and kickstart your journey towards success in the Class 5 exam.
Importance of Important Questions: How Big, How Heavy
The importance of Important Questions: How Big, How Heavy cannot be overstated, especially for Class 5 aspirants.
This document holds the key to success in the Class 5 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Important Questions: How Big, How Heavy Notes
Important Questions: How Big, How Heavy Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Important Questions: How Big, How Heavy.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Important Questions: How Big, How Heavy Notes on EduRev are your ultimate resource for success.
Important Questions: How Big, How Heavy Class 5
The "Important Questions: How Big, How Heavy Class 5 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 5 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Important Questions: How Big, How Heavy on the App
Students of Class 5 can study Important Questions: How Big, How Heavy alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Important Questions: How Big, How Heavy,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Important Questions: How Big, How Heavy is prepared as per the latest Class 5 syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup