Important Derivations: Wave Optics | Physics Class 12 - NEET PDF Download
Derivations related to Huygens’ Principle
(1) Using Huygens’ principle, verify the laws of reflection at a plane surface.
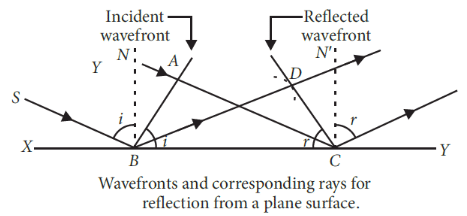
First the wavefront touches the reflecting surface at B and then at the successive points towards C. In accordance with Huygens’ principle, from each point on BC secondary wavelets start growing with the speed c. During the time the disturbance from A reaches the point C, the secondary wavelets from B must have spread over a hemisphere of radius DB = AC = ct, where ‘t’ is the time taken by the disturbance to travel from A to C. The tangent plane CD drawn from the point ‘C’ over this hemisphere of radius ‘ct’ will be the new reflected wavefront. Let angles of incidence and reflection be i and r respectively. In ΔABC and ΔDCB, we have
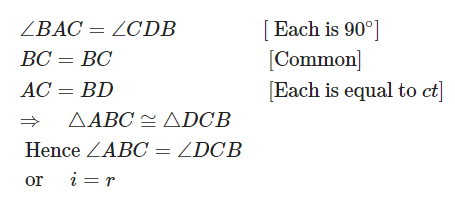
i.e., the angle of incidence is equal to the angle of reflection. This proves the first law of reflection.
(2) Use Huygens’ principle to verify the laws of refraction.
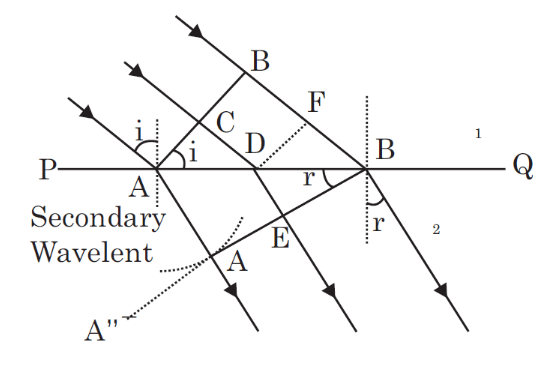
Laws of refraction: Suppose when distribution from point P on incident wave front reaches point P on the refracted wave front the disturbance from point Q reaches the point Q or the refracting surface XY. Since, A ‘Q’ P’ represents the refracted wave front the time takes by light to travel from a point on incident wave front to the corresponding point on refracted wave front would always be the same. Now, time taken by light to go from r to Q’ will be-
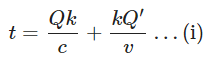
(Where c and v are the velocities of light in two medium)
In right angled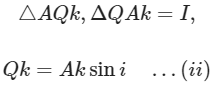
In right angle

Substituting eq. (ii) and (iii) in eq. (i), we get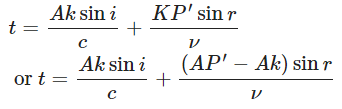
The rays from different points or the incident wave front will take the same time to reach the corresponding points on the refracted wave front i.e., given by equation (iv) is independent of Ak. will happens so, if

However,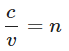
This is the shell’s law for refraction of light.
Introduction To Young’s Double Slits Experiment
During the year 1801, Thomas Young carried out an experiment where the wave and particle nature of light and matter were demonstrated. The schematic diagram of the experimental setup is shown below-
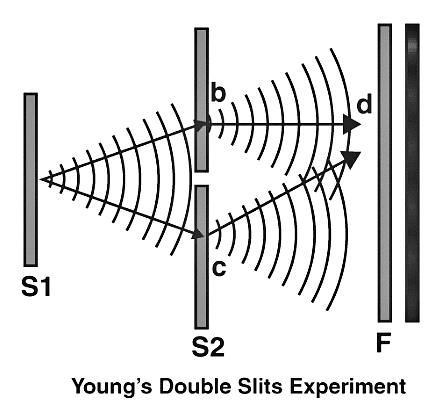
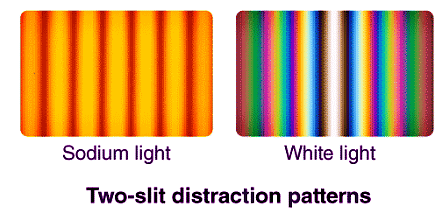
A beam of monochromatic light is made incident on the first screen, which contains the slit S0. The emerging light then incident on the second screen which consists of two slits namely, S1, S2. These two slits serve as a source of coherent light. The emerging light waves from these slits interfere to produce an interference pattern on the screen. The interference pattern consists of consecutive bright and dark fringes. The dark fringes are the result of destructive interference and bright fringes are the result of constructive interference.
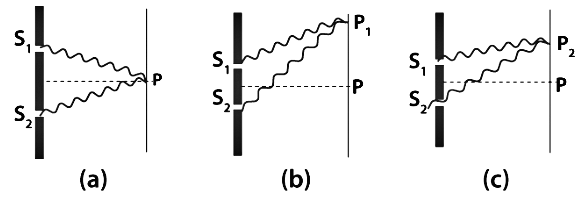
Figure(2): shows the interference pattern of two light waves to produce dark or bright fringes. Bright fringe(at P) is formed due to the overlap of two maxima or two minima. Figure 2a,2b . Dark fringe(at P) is formed due to the overlap of maxima with minima. Figure 2c.
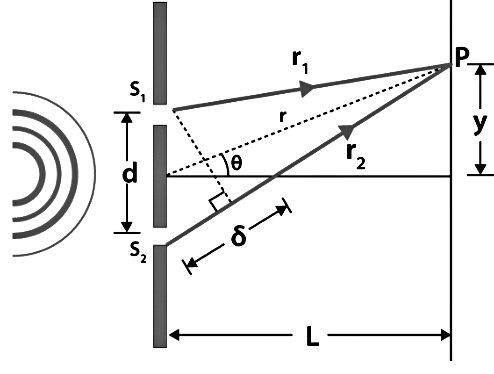
- The light falls on the screen at the point P. which is at a distance y from the centre O.
- The distance between the double-slit system and the screen is L
- The two slits are separated by the distance d
- Distance travelled by the light ray from slit 1 to point P on the screen is r1
- Distance travelled by the light ray from slit 2 to point P on the screen is r2
- Thus, the light ray from slit 2 travels an extra distance of ẟ = r2-r1 than light ray from slit 1.
- This extra distance is termed as Path difference.
Refer to Figure(3) Applying laws of cosines; we can write –
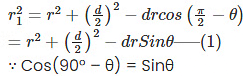
Similarly,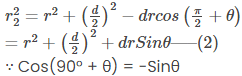
Subtracting equation (2) from (1) we get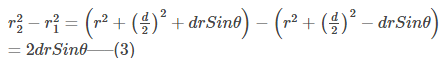
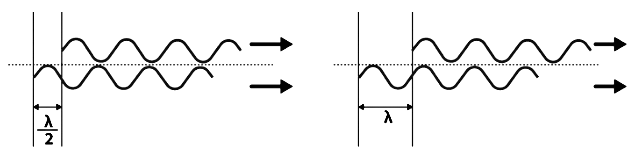
Let us impose the limit that the distance between the double slit system and the screen is much greater than the distance between the slits. That is L >> d. The sum of r1 and r2 can be approximated to r1 + r2 ≅2r. Thus, the path difference becomes – 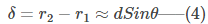
In this limit, the two rays r1 and r2 are essentially treated as parallel. (See Figure(4))
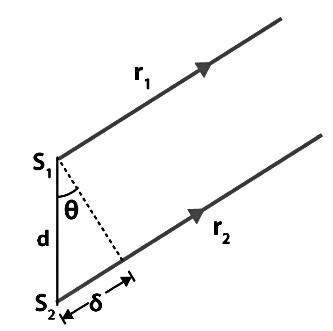
Figure(4): Assuming L >> d, The path difference between two rays.
To compare the phase of two waves, the value of path difference (ẟ) plays a crucial role.
Constructive interference is seen when path difference (𝛿) is zero or integral multiple of the wavelength (λ).
(Constructive interference) 𝛿 = dSin𝜃 = mλ —-(5), m = 0, ±1, ±2, ±3, ±4, ±5, ……
Where m is order number. The Zeroth order maximum (m=0)corresponds to the central bright fringe, here 𝜃=0. The first order maxima(m=±1)(bright fringe) are on either side the central fringe.
Similarly, when 𝛿 is an odd integral multiple of λ/2, the resultant fringes will be 1800 out of phase, thus, forming a dark fringe.
(Destructive interference) 𝛿 = dSin𝜃 = 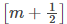
λ—(6), m = 0, ±1, ±2, ±3, ±4, ±5, ……
Assuming the distance between the slits are much greater than the wavelength of the incident light, we get-
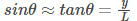
Substituting it in the constructive and destructive interference condition we can get the position of bright and dark fringes, respectively. The equation is as follows-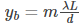
And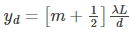
|
97 videos|336 docs|104 tests
|
FAQs on Important Derivations: Wave Optics - Physics Class 12 - NEET
| 1. What is Huygens' Principle? |  |
| 2. How is Huygens' Principle related to wave optics? |  |
| 3. What are some derivations related to Huygens' Principle? |  |
| 4. What is Young's double slit experiment? |  |
| 5. How does Young's double slit experiment relate to wave optics? |  |

|
Explore Courses for NEET exam
|

|

















