Important Formulas: Circles - GMAT PDF Download
| Table of contents |

|
| Area of a Circle |

|
| Circumference of a Circle |

|
| Segment of a Circle |

|
| Formulas List |

|
Area of a Circle

The area of a circle is πr2, where π=22/7 or ≈ 3.14 (can be used interchangeably for problem-solving purposes) and r is the radius of the circle.
π is the ratio of the circumference of a circle to its diameter.
Example: Find the area of a circle with radius = 7cm.
Solution: Given, radius of circle = 7cm
By the formula we know;
Area of circle = πr2
= π(7)2
= (22/7) (7)2
= 154 sq.cm.
Circumference of a Circle
The circumference of a circle is the distance covered by going around its boundary once.

The perimeter of a circle has a special name: Circumference, which is π times the diameter which is given by the formula;
Circumference of a circle = 2πr.
Example: The circumference of a circle whose radius is 21cm is given by;
C = 2πr
= 2 (22/7) (21)
= 132 cm
Segment of a Circle
A circular segment is a region of a circle that is “cut off” from the rest of the circle by a secant or a chord.
Sector of a Circle
A circle sector/ sector of a circle is defined as the region of a circle enclosed by an arc and two radii. The smaller area is called the minor sector, and the larger area is called the major sector.
Angle of a Sector
The angle of a sector is the angle that is enclosed between the two radii of the sector.
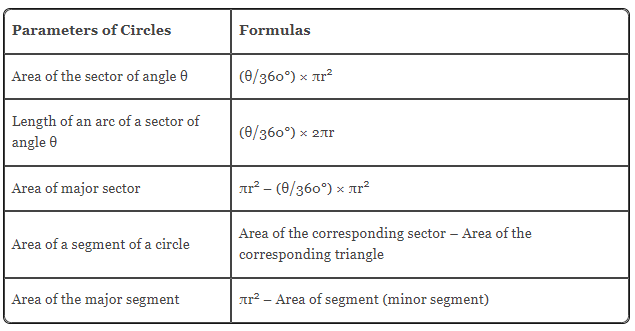
Area of a Sector of a Circle
The area of a sector is given by
(θ/360°)×πr2
where ∠θ is the angle of this sector(minor sector in the following case) and r is its radius
Example: Suppose the sector of a circle is 45° and radius is 4 cm, then the area of the sector will be:
Area = (θ/360°) × πr2
= (45°/360°) × (22/7) × 4 × 4
= 44/7 sq. cm
Length of an Arc of a Sector

The length of the arc of a sector can be found by using the expression for the circumference of a circle and the angle of the sector, using the following formula:
L= (θ/360°)×2πr
Where θ is the angle of sector and r is the radius of the circle.
Area of a Triangle
The area of a triangle is,
Area=(1/2)× base × height
If the triangle is an equilateral then,
Area=(√3/4)×a2 where “a” is the side length of the triangle.
Area of a Segment of a Circle
 Area of segment APB (highlighted in yellow) = (Area of sector OAPB) – (Area of triangle AOB)
Area of segment APB (highlighted in yellow) = (Area of sector OAPB) – (Area of triangle AOB)
=[(∅/360°)×πr2] – [(1/2)×AB×OM]
[To find the area of triangle AOB, use trigonometric ratios to find OM (height) and AB (base)]
Also, the area of segment APB can be calculated directly if the angle of the sector is known using the following formula.
=[(θ/360°)×πr2] – [r2×sin θ/2 × cosθ/2]
Where θ is the angle of the sector and r is the radius of the circle.
Formulas List