Overview: Logarithm | General Aptitude for GATE - Mechanical Engineering PDF Download
| Table of contents |

|
| History |

|
| What are Logarithms? |

|
| Logarithm Types |

|
| Logarithm Rules and Properties |

|
| Logarithmic Formulas |

|
In Mathematics, logarithms are the other way of writing the exponents. A logarithm of a number with a base is equal to another number. A logarithm is just the opposite function of exponentiation. For example, if 102 = 100 then log10 100 = 2.
Hence, we can conclude that,
Logb x = n or bn = x
Where b is the base of the logarithmic function.
This can be read as “Logarithm of x to the base b is equal to n”.
History
John Napier introduced the concept of Logarithms in the 17th century. Later it was used by many scientists, navigators, engineers, etc for performing various calculations which made it simple. In simple words, Logarithms are the inverse process of exponentiation.
What are Logarithms?
A logarithm is defined as the power to which a number must be raised to get some other values. It is the most convenient way to express large numbers. A logarithm has various important properties that prove multiplication and division of logarithms can also be written in the form of logarithm of addition and subtraction.
“The logarithm of a positive real number a with respect to base b, a positive real number not equal to 1[nb 1], is the exponent by which b must be raised to yield a”.
i.e. by = a ⇔ logba = y
Where,
- “a” and “b” are two positive real numbers
- y is a real number
- “a” is called argument, which is inside the log
- “b” is called the base, which is at the bottom of the log.
In other words, the logarithm gives the answer to the question “How many times a number is multiplied to get the other number?”.
For example, how many 3’s are multiplied to get the answer 27?
If we multiply 3 for 3 times, we get the answer 27.
Therefore, the logarithm is 3.
The logarithm form is written as follows:
Log3 (27) = 3 ….(1)
Therefore, the base 3 logarithm of 27 is 3.
The above logarithm form can also be written as:
3 x 3 x 3 = 27
33 = 27 …..(2)
Thus, the equations (1) and (2) both represent the same meaning.
Below are some of the examples of conversion from exponential forms to logarithms.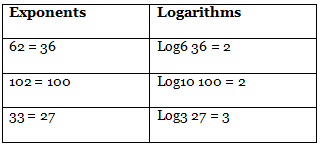
Logarithm Types
In most cases, we always deal with two different types of logarithms, namely
- Common Logarithm
- Natural Logarithm
Common Logarithm
The common logarithm is also called the base 10 logarithms. It is represented as log10 or simply log. For example, the common logarithm of 1000 is written as a log (1000). The common logarithm defines how many times we have to multiply the number 10, to get the required output.
For example, log (100) = 2
If we multiply the number 10 twice, we get the result 100.
Natural Logarithm
The natural logarithm is called the base e logarithm. The natural logarithm is represented as ln or loge. Here, “e” represents the Euler’s constant which is approximately equal to 2.71828. For example, the natural logarithm of 78 is written as ln 78. The natural logarithm defines how many we have to multiply “e” to get the required output.
For example, ln (78) = 4.357.
Thus, the base e logarithm of 78 is equal to 4.357.
Logarithm Rules and Properties
There are certain rules based on which logarithmic operations can be performed. The names of these rules are:
- Product rule
- Division rule
- Power rule/Exponential Rule
- Change of base rule
- Base switch rule
- Derivative of log
- Integral of log
Let us have a look at each of these properties one by one
Product Rule
In this rule, the multiplication of two logarithmic values is equal to the addition of their individual logarithms.
Logb (mn)= logb m + logb n
For example: log3 ( 2y ) = log3 (2) + log3 (y)
Division Rule
The division of two logarithmic values is equal to the difference of each logarithm.
Logb (m/n)= logb m – logb n
For example, log3 (2/ y) = log3 (2) -log3 (y)
Exponential Rule
In the exponential rule, the logarithm of m with a rational exponent is equal to the exponent times its logarithm.
Logb (mn) = n logb m
Example 1: logb(23) = 3 logb 2
Change of Base Rule
Logb m = loga m/ loga b
Example 2: logb 2 = loga 2/loga b
Base Switch Rule
logb (a) = 1 / loga (b)
Example3: logb 8 = 1/log8 b
Derivative of log
If f (x) = logb (x), then the derivative of f(x) is given by;
f'(x) = 1/(x ln(b))
Example 4: Given, f (x) = log10 (x)
Then, f'(x) = 1/(x ln(10))
Integral of Log
∫logb(x)dx = x(logb(x) – 1/ln(b)) + C
Example 5: ∫ log10(x) dx = x ∙ (log10(x) – 1 / ln(10)) + C
Other Properties
Some other properties of logarithmic functions are:
- Logb b = 1
- Logb 1 = 0
- Logb 0 = undefined
Logarithmic Formulas
logb(mn) = logb(m) + logb(n)
logb(m/n) = logb(m) – logb(n)
Logb (xy) = y logb(x)
Logbm√n = logb n/m
m logb(x) + n logb(y) = logb(xmyn)
logb(m+n) = logb m + logb(1+nm)
logb(m – n) = logb m + logb (1-n/m)
Example 1: Solve log2 (64) =?
Sol: since 26 = 2 × 2 × 2 × 2 × 2 × 2 = 64, 6 is the exponent value and log2 (64)= 6.
Example 2: What is the value of log10(100)?
Sol: In this case, 102 yields you 100. So, 2 is the exponent value, and the value of log10(100) = 2
Example 3: Use of the property of logarithms, solve for the value of x for log3 x = log3 4 + log3 7
Sol: By the addition rule, log3 4+ log3 7= log3 (4 * 7 )
Log3 (28). Thus, x = 28.
Example 4: Solve for x in log2 x = 5
Sol: This logarithmic function can be written In the exponential form as 25 = x
Therefore, 25 = 2 × 2 × 2 × 2 × 2 = 32, x = 32.
Example 5: Find the value of log5 (1/25).
Sol: Given: log5 (1/25)
By using the property,
Logb (m/n)= logb m – logb n
log5 (1/25) = log5 1 – log5 25
log5 (1/25) = 0 – log5 52
log5 (1/25) = -2log55
log5 (1/25) = -2 (1) [By using the property loga a = 1)
log5 (1/25) = -2.
Hence, the value of log5 (1/25) = -2
|
206 videos|190 docs|158 tests
|




















