NCERT Solutions Class 11 Maths Chapter 7 - Binomial Theorem
Question 1: If a and b are distinct integers, prove that a – b is a factor of an – bn, whenever n is a positive integer.
[Hint: write an = (a – b + b)n and expand]
ANSWER : - In order to prove that (a – b) is a factor of (an – bn), it has to be proved that
an – bn = k (a – b), where k is some natural number
It can be written that, a = a – b + b
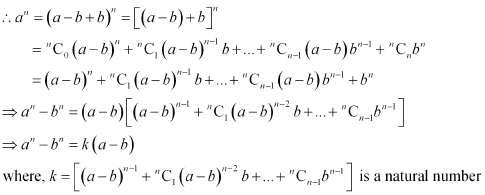
This shows that (a – b) is a factor of (an – bn), where n is a positive integer.
Question 2: Evaluate 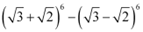 .
.
ANSWER : - Firstly, the expression (a + b)6 – (a – b)6 is simplified by using Binomial Theorem.
This can be done as
Question 3: Find the value of 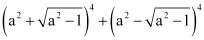 .
.
ANSWER : - Firstly, the expression (x + y)4 (x – y)4 is simplified by using Binomial Theorem.
This can be done as
Question 4: Find an approximation of (0.99)5 using the first three terms of its expansion.
ANSWER : - 0.99 = 1 – 0.01
Thus, the value of (0.99)5 is approximately 0.951.
Question 5: Find n, if the ratio of the fifth term from the beginning to the fifth term from the end in the expansion of 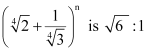
ANSWER : - In the expansion, 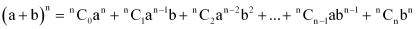 ,
,
Fifth term from the beginning 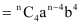
Fifth term from the end 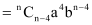
Therefore, it is evident that in the expansion of 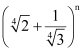 , the fifth term from the beginning is
, the fifth term from the beginning is 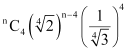 and the fifth term from the end is
and the fifth term from the end is 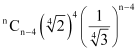 .
.
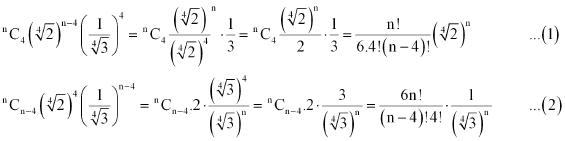
It is given that the ratio of the fifth term from the beginning to the fifth term from the end is 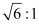 . Therefore, from (1) and (2), we obtain
. Therefore, from (1) and (2), we obtain
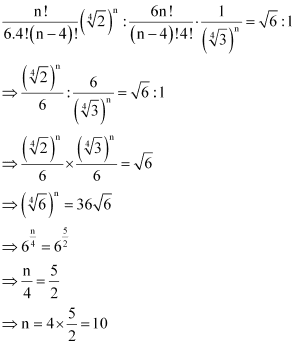
Thus, the value of n is 10.
Question 6: Expand using Binomial Theorem 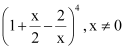 .
.
ANSWER : - Using Binomial Theorem, the given expression 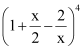 can be expanded as
can be expanded as
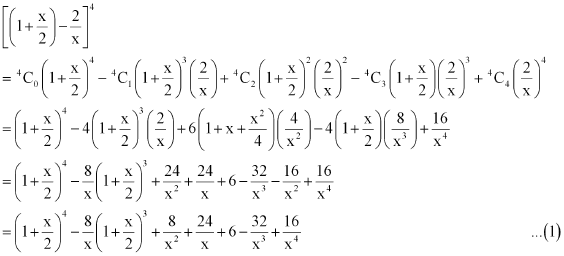
Again by using Binomial Theorem, we obtain
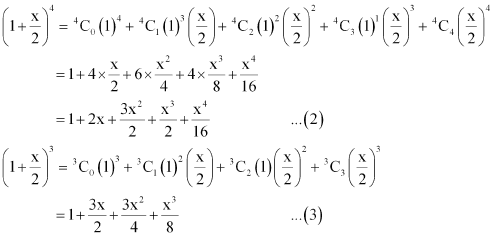
From (1), (2), and (3), we obtain
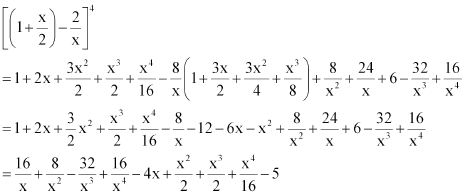
Question 7: Find the expansion of 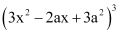 using binomial theorem.
using binomial theorem.
ANSWER : - Using Binomial Theorem, the given expression 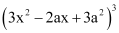 can be expanded as
can be expanded as
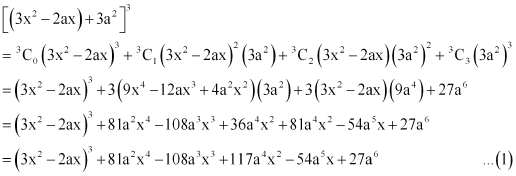
Again by using Binomial Theorem, we obtain
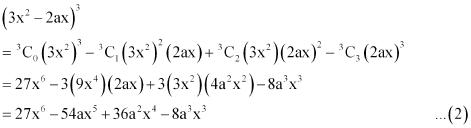
From (1) and (2), we obtain
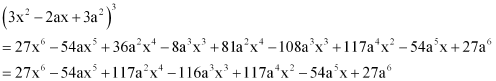
|
73 videos|264 docs|91 tests
|
















