Tips and Tricks: Logarithms | Quantitative Aptitude for SSC CGL PDF Download
Definition
A logarithm serves as the inverse function of exponentiation. It represents the power to which a specific number must be raised to yield another given number. Introduced by John Napier in the early 17th century, logarithms aimed to streamline calculations.
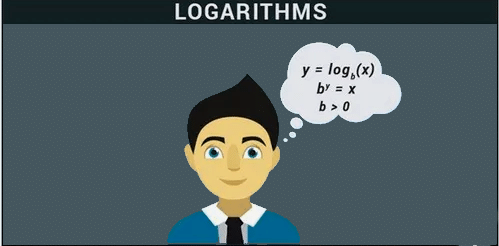
Logarithms are categorized into two types
- Common Logarithm: Logarithms with a base of 10 are termed common logarithms.
- Natural Logarithm: Logarithms with a base of 'e' are known as natural logarithms.
Example of Logarithm:
Example: log 100 = 2
Taking LHS
log 102
= 2 log 10
= 2 × 1 (∴ Log 10 = 1)
= 2.
Let’s have a look on some question that will help better in understanding Logarithm.
Basic Difference Between Logarithm and Natural Logarithm:
- Logarithm: Picture having a number and wondering how many instances you must multiply a designated "base" number to reach that given number. The response to this query is the logarithm of the specified number.
- Natural Logarithm: This is a distinctive kind of logarithm that employs a particular base denoted as "e" (a unique number approximately equal to 2.71828). It indicates the number of times you must multiply "e" to achieve a specific number.
Logarithm Tips, Tricks and Shortcuts
Q1: Which of the following statement is not correct?
(a) log (1 + 2 + 3) = log 1 + log 2 + log 3
(b) log( 2+3) = log (2×3)
(c) log10 1 = 0
(d) log10 10 = 1
Ans: (b)
(a) log( 1+2+3) = log6 = log (1×2×3) = log1+ log2+log3
(b) log (2 + 3) = log 5 and log (2 x 3) = log 6 = log 2 + log 3
log (2 + 3) ≠ log (2 x 3)
(c) Since, loga 1=0,
so log10 1=0
(d) Since, loga a = 1
so, log10 10 = 1.
Q3: Solve for x: log2 (x + 3) + log2 (x – 1) = 3
Sol: Combine the logarithms using the logarithm property log(a) + log(b) = log(a * b):
log2 ((x + 3)×(x − 1)) = 3
23 =(x+3)×(x−1)
8 = (x + 3) × (x − 1)
8 = x2 + 2x − 3
x2 + 2x − 11 = 0
(x + 4)(x - 2) = 0
x = -4, 2
Q2: If X is an integer then solve(log2 X)2– log2x4-32 = 0
Sol: Let us consider,(log2 X)2– log2x4-32 = 0 —— equation 1
let log2 x= y
equation 1 = y2– 4y-32=0
y2-8y+ 4y – 32= 0
y(y-8) + 4(y-8) =0
(y-8) (y+4)= 0
y=8, y= -4
log2X = 8 or, log2X = -4
X = 28 = 256, 0r
Since, X is an integer so X = 256.
|
317 videos|294 docs|185 tests
|
FAQs on Tips and Tricks: Logarithms - Quantitative Aptitude for SSC CGL
| 1. What is a logarithm? |  |
| 2. Why are logarithms useful in mathematics? |  |
| 3. What are the properties of logarithms? |  |
| 4. How do logarithms help solve exponential equations? |  |
| 5. Can logarithms be negative or zero? |  |
















