Functions of Single Variable | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE) PDF Download
| Table of contents |

|
| Limit of a function |

|
| Properties of Limits |

|
| Standard Formulae |

|
| Solved Numericals |

|
A real valued function y = f(x) of a real variable x is a mapping whose domain S and co-domain R are sets of real numbers. The range of the function is the set 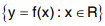 , which is a subset of R.
, which is a subset of R.
Limit of a function
The function f is said to tend to the limit l as x → a, if for a given positive real number ε > 0 we can find a real number δ > 0 such that 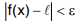 whenever
whenever 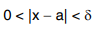 Symbolically we write
Symbolically we write 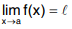
Left Hand and Right Hand Limits
Let x < a and x → a from the left hand side.
If |f(x) - l1 | < ε, a - δ < x < a or 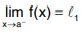
then l1 is called the left hand limit.
Let x > a and x → a from the right hand side.
If |f(x) l2 | < ε, a < x < a + δ or 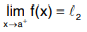
then l2 is called the right hand limit.
If l1 = l2 then 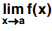 exists. If the limit exists then it is unique.
exists. If the limit exists then it is unique.
Properties of Limits
Let f and g be two functions defined over S and let a be any point, not necessarily in S
Ans if 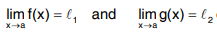 exist, then
exist, then
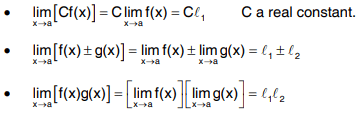
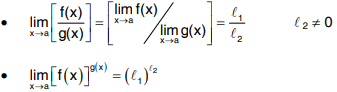
Standard Formulae
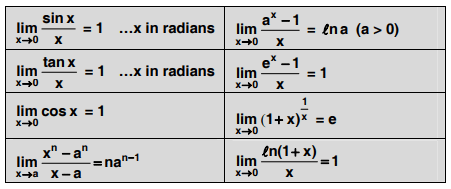
Solved Numericals
Q1. Show that 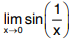 does not exist.
does not exist.
Solution : For different values of x in the interval 0 < | x | < δ the function 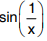 takes values between -1 and 1. Since
takes values between -1 and 1. Since 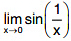 is not unique limit does not exist
is not unique limit does not exist
Q2. Show that 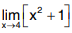 does not exist, where [] is the greatest integer function
does not exist, where [] is the greatest integer function
Solution : Let h > 0, we have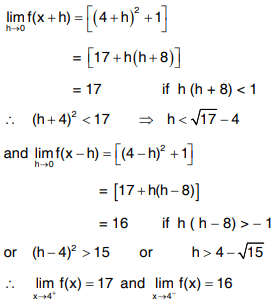
The limit does not exist.
|
44 videos|109 docs|58 tests
|
FAQs on Functions of Single Variable - Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE)
| 1. What are some common properties of limits of functions? |  |
| 2. How can the limit of a function be calculated using standard formulae? |  |
| 3. What is the significance of limits in mechanical engineering applications? |  |
| 4. How do functions of a single variable impact mechanical engineering calculations? |  |
| 5. How can an understanding of limits and functions of a single variable benefit mechanical engineering students and professionals? |  |















