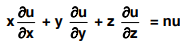Total Derivative, Gradient, Divergence, and Curl | Engineering Mathematics for Electrical Engineering - Electrical Engineering (EE) PDF Download
| Table of contents |

|
| Partial and Total Derivatives |

|
| Partial derivatives of higher order |

|
| Euler’s theorem on Homogenous functions |

|
| Composite functions |

|
Partial and Total Derivatives
Functions of two variablesIf three variables x, y, z are so related that the value of z depends upon the values of x and y, then z is called the function of two variables x and y and this is denoted by z = f(x, y)
Partial derivatives of first order
Let z = f(x, y) be a function of two independent variables x and y. If y is kept constant and x alone is allowed to vary then z becomes a function of x only. The derivative of z with respect to x, treating y as constant, is called partial derivative of z with respect to x and is denoted by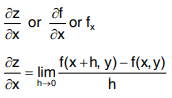 Similarly the derivative of z with respect to y, treating x as constant, is called partial derivative of z with respect to y and is denoted by
Similarly the derivative of z with respect to y, treating x as constant, is called partial derivative of z with respect to y and is denoted by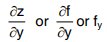
Thus 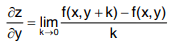
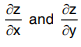 are called first order partial derivatives of z
are called first order partial derivatives of z

Partial derivatives of higher order
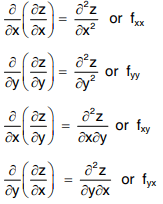
In general, 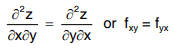
Solved Examples 1: First order partial derivative of u = yx is
Solution : u = yx
Treating y as constant
treating x as constant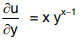
Euler’s theorem on Homogenous functions
If u is a homogenous function of degree n in x and y then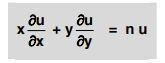 Since u is a homogenous function of degree n in x and y it can be expressed as
Since u is a homogenous function of degree n in x and y it can be expressed asu = xn f
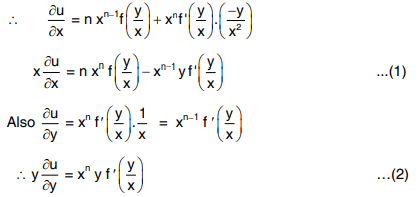
Adding (1) and (2) we get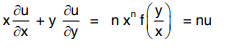
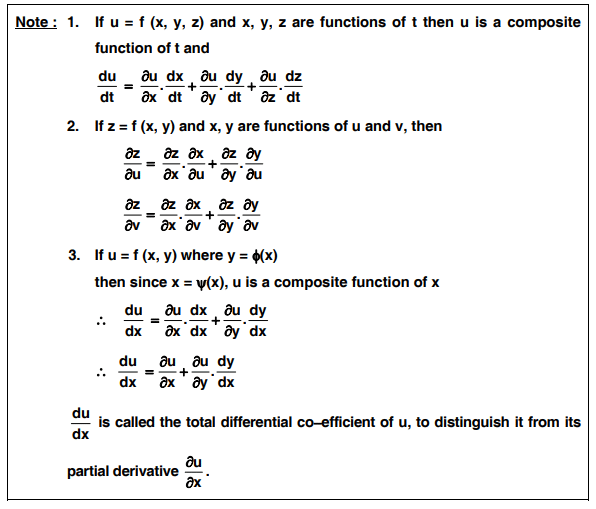
Note: Euler’s theorem can be extended to a homogenous function of any number of variables. Thus if u is a homogenous function of degree n in x, y and z then
Solved Examples 2: If 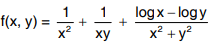 show that
show that 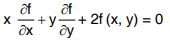 .
.
Solution: 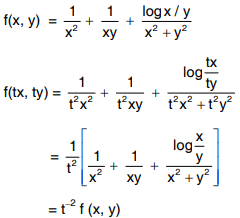
f (x, y) is a homogenous function of degree -2 in x and y
By Euler’s theorem, we have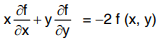
Composite functions
(i) If u = f (x, y) where x = ϕ(t), y = Ψ(t)then u is called a composite function of (the single variable) t and we can find du/dt .
(ii) If z = f(x, y) where x = ϕ(u, v), y = Ψ(u, v)
then z is called a composite function of (2 variables) u & v so that we can find 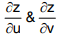
Total derivative of composite functions
If u is a composite function of t, defined by the relations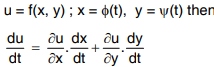
Solved Example 3: Find du/dt when u = x when u = xy2 + x2 y, x = at2, y = 2at.
Solution: The given equations define u as a composite function of t.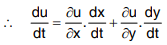
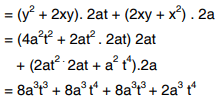
= 2a3 t3 (5t + 8)
Also u = xy2 + x2 y = at2 . 4a2 t2 + a2 t4 . 2at = 4a3 t4 + 2a3 t5 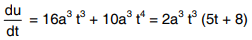
|
44 videos|109 docs|58 tests
|