Unit Test (Solutions): Triangles | Mathematics (Maths) Class 10 PDF Download
Time: 1 hour
M.M. 30
Attempt all questions.
- Question numbers 1 to 5 carry 1 mark each.
- Question numbers 6 to 8 carry 2 marks each.
- Question numbers 9 to 11 carry 3 marks each.
- Question number 12 & 13 carry 5 marks each.
Q1. In ΔPQR, if PS is the internal bisector of ∠P meeting QR at S and PQ = 15 cm, QS = (3 + x) cm, SR = (x – 3) cm and PR = 7 cm, then find the value of x. (1 Mark)
(a) 2.85 cm
(b) 8.25 cm
(c) 5.28 cm
(d) 8.52 cm
Ans: (b)
Since PS is the internal bisector of ∠P and it meets QR at S .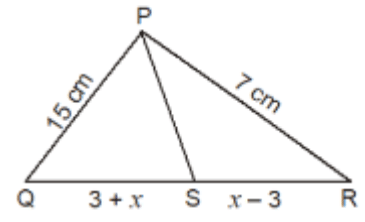
∴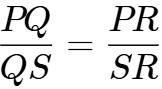
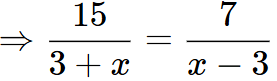
⇒ 7(3+x) = 15(x–3)
⇒ 21+7x = 15x–45
⇒ 15x–7x = 45+21
⇒ 8x = 66
⇒ 4x = 33
⇒ x = 8.25cm
Q2: If ABC and DEF are two triangles and AB/DE=BC/FD, then the two triangles are similar if (1 Mark)
(a) ∠A=∠F
(b) ∠B=∠D
(c) ∠A=∠D
(d) ∠B=∠E
Ans: (b) ∠B=∠D
Given, the triangles ABC and DEF
Also, AB/DE = BC/FD
We have to choose the correct condition so the triangles will be similar.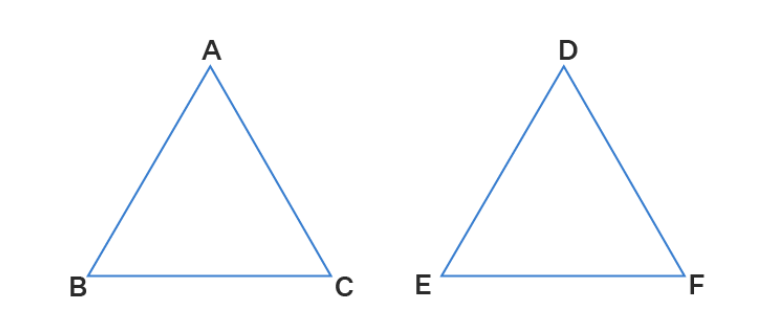
SSS criterion states that if three sides of one triangle are proportional to three sides of another triangle, then the triangles are similar.
By SSS criterion,
AB/DE = BC/DF = AC/EF
We know that similar triangles have congruent corresponding angles and the corresponding sides are in proportion.
So, ∠A = ∠E
∠B = ∠D
∠C = ∠F
So, option C is true.
Therefore, the triangles to be similar when ∠B = ∠D.
If ABC and DEF are two triangles and AB/DE = BC/FD, then the two triangles are similar if ∠B=∠D.
Q3: If in two triangles ABC and PQR, AB/QR = BC/PR = CA/PQ, then. (1 Mark)
(a) ΔPQR ~ ΔCAB
(b) ΔPQR ~ ΔABC
(c) ΔCBA ~ ΔPQR
(d) ΔBCA ~ ΔPQR
Ans: (a)
Given that, in triangles ABC and PQR, AB/QR = BC/PR = CA/PQ
It is clear that the corresponding sides of the two triangles are equal.
By SSS criterion, the corresponding vertices are as follows:
A ↔ Q
B ↔ R
C ↔ P
Therefore, we have △PQR ~ △CAB
Q4: Write the truth value (T/F) of each of the following statements : (1 Mark)
(i) Any two similar figures are congruent.
(ii)Two polygons are similar, if their corresponding sides are proportional.
Ans: (i) False : In some cases, the similar polygons can be congruent.
(ii) False : Its corresponding angles must be equal also.
Q5: D and E are respectively the points on sides AB and AC of triangle ABC such that AB = 3 cm, BD = 1.5 cm, BC = 7.5 cm, and DE || BC. What is the length of DE? (1 Mark)
(a) 2 cm
(b) 2.5 cm
(c) 3.75 cm
(d) 3 cm
Ans: (c)
Since DE∥BC, by the Basic Proportionality Theorem (Thales' Theorem), the triangles △ADE and △ABC are similar. This means the corresponding sides are proportional.
The ratio of the sides is given by: 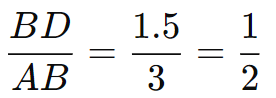
Since DE∥BC, the length of DE will be proportional to BC: 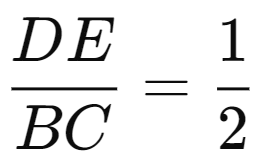
Given BC=7.5cm, we can calculate the length of DE:
DE = 1/2 x 7.5 = 3.75
Thus, the length of DE is 3.75 cm.
Q6: In the figure, DE // AC and DF // AE. Prove that BF/FE = BE/EC. (2 Marks)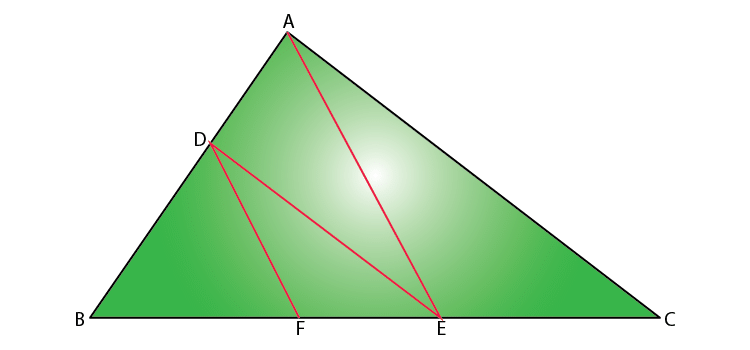
Ans:
Given that,
In triangle ABC, DE // AC.
By Basic Proportionality Theorem,
BD/DA = BE/EC……….(i)
Also, given that DF // AE.
Again by Basic Proportionality Theorem,
BD/DA = BF/FE……….(ii)
From (i) and (ii),
BE/EC = BF/FE
Hence, proved.
Q7: In the figure, DE || BC. Find the length of side AD, given that AE = 1.8 cm, BD = 7.2 cm and CE = 5.4 cm. (2 Marks)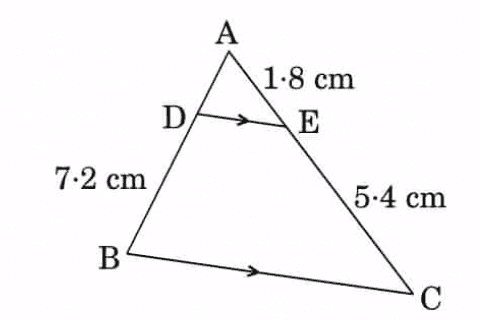
Ans: Given, DE || BC
AE = 1.8 cm, BD = 7.2 cm and CE = 5.4 cm
By basic proportionality theorem,
AD/DB = AE/EC
AD/7.2 = 1.8/5.4
AD = (1.8 × 7.2)/5.4
= 7.2/4
= 2.4
Therefore, AD = 2.4 cm.
Q8: In the given figure, XY || QR,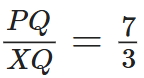 and PR = 6.3 cm, find YR. (2 Marks)
and PR = 6.3 cm, find YR. (2 Marks)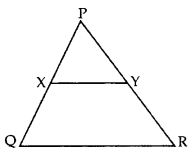
Ans: Let YR = x 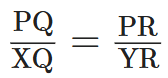 ..... Thales Theorem
..... Thales Theorem
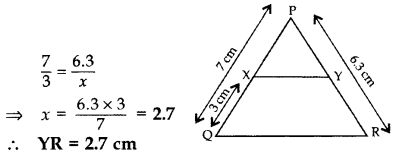
Q9: D and E are points on sides AB and AC of triangle ABC such that DE || BC. If AD = 2·4 cm, DB = 3.6 cm and AC = 5 cm, find AE. (3 Marks)
Ans: Given, DE || BC, AD = 2.4 cm, DB = 3.6 cm and AC = 5 cm
In ABC, DE || BC
∴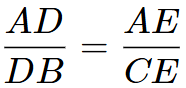 (by basic proportionality theorem)
(by basic proportionality theorem)
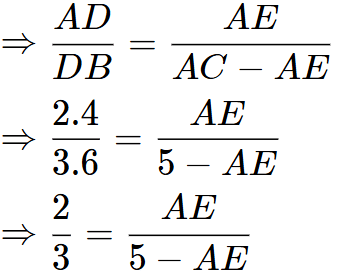
⇒3AE + 2AE = 10
⇒3AE = 10 − 2AE
⇒AE = 10/5
⇒AE = 2cm
Q10: X and Y are points on the sides AB and AC respectively of a triangle ABC such that 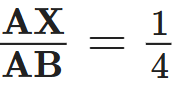 , AY = 2 cm and YC = 6 cm. Find whether XY || BC or not. (3 Marks)
, AY = 2 cm and YC = 6 cm. Find whether XY || BC or not. (3 Marks)
Ans:
Given, 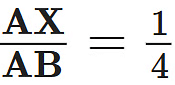
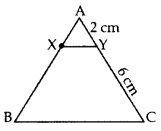
AX = 1K, AB = 4K
∴ BX = AB – AX
= 4K – 1K = 3K 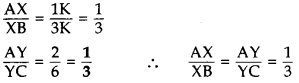
∴ XY || BC … [By converse of Thales’ theorem
Q11: If a line segment intersects sides AB and AC of a ∆ABC at D and E respectively and is parallel to BC, prove that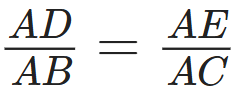 . (3 Marks)
. (3 Marks)
Ans: Given. In ∆ABC, DE || BC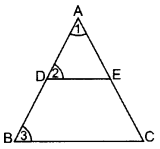
Proof.
In ∆ADE and ∆ABC
∠1 = ∠1 … Common
∠2 = ∠3 … [Corresponding angles]
∆ADE ~ ∆ABC …[AA similarity]
∴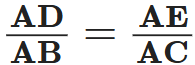 …[In ~∆s corresponding sides are proportional]
…[In ~∆s corresponding sides are proportional]
Q12: In the given figure, altitudes AD and CE of ∆ ABC intersect each other at the point P. Show that: (5 Marks)
(i) ∆AEP ~ ∆ CDP
(ii) ∆ABD ~ ∆ CBE
(iii) ∆AEP ~ ∆ADB
(iv) ∆ PDC ~ ∆ BEC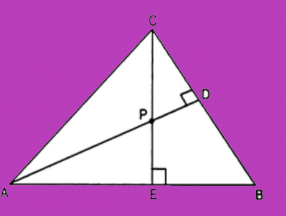
Ans:
Given that AD and CE are the altitudes of triangle ABC and these altitudes intersect each other at P.
(i) In ΔAEP and ΔCDP,
∠AEP = ∠CDP (90° each)
∠APE = ∠CPD (Vertically opposite angles)
Hence, by AA similarity criterion,
ΔAEP ~ ΔCDP
(ii) In ΔABD and ΔCBE,
∠ADB = ∠CEB ( 90° each)
∠ABD = ∠CBE (Common Angles)
Hence, by AA similarity criterion,
ΔABD ~ ΔCBE
(iii) In ΔAEP and ΔADB,
∠AEP = ∠ADB (90° each)
∠PAE = ∠DAB (Common Angles)
Hence, by AA similarity criterion,
ΔAEP ~ ΔADB
(iv) In ΔPDC and ΔBEC,
∠PDC = ∠BEC (90° each)
∠PCD = ∠BCE (Common angles)
Hence, by AA similarity criterion,
ΔPDC ~ ΔBEC
Q13: In given figure, EB ⊥ AC, BG ⊥ AE and CF ⊥ AE. (5 Marks)
Prove that:
(a) ∆ABG ~ ∆DCB
(b) 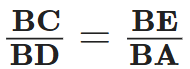
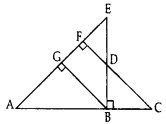
Ans: 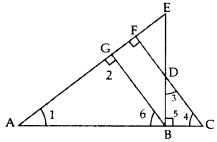
Given: EB ⊥ AC, BG ⊥ AE and CF ⊥ AE.
To prove: (a) ∆ABG – ∆DCB,
(b) 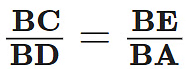
Proof: (a) In ∆ABG and ∆DCB,
∠2 = ∠5 … [each 90°
∠6 = ∠4 … [corresponding angles
∴ ∆ABG ~ ∆DCB … [By AA similarity
(Hence Proved)
∴ ∠1 = ∠3 …(CPCT … [In ~∆s, corresponding angles are equal
(b) In ∆ABE and ∆DBC,
∠1 = ∠3 …(proved above
∠ABE = ∠5 … [each is 90°, EB ⊥ AC (Given)
∆ABE ~ ∆DBC … [By AA similarity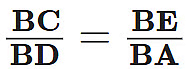 … [In ~∆s, corresponding sides are proportional ]
… [In ~∆s, corresponding sides are proportional ]
∴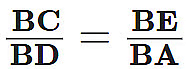 ... Hence, Proved.
... Hence, Proved.
|
127 videos|584 docs|79 tests
|
FAQs on Unit Test (Solutions): Triangles - Mathematics (Maths) Class 10
| 1. What are the different types of triangles based on their sides? |  |
| 2. How can I determine the area of a triangle? |  |
| 3. What is the Pythagorean theorem and how is it applied to right triangles? |  |
| 4. How do I find the perimeter of a triangle? |  |
| 5. What is the significance of triangle inequalities in triangle construction? |  |





















