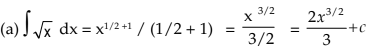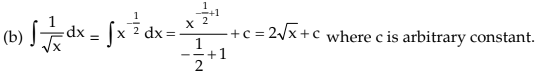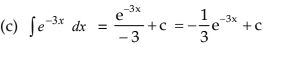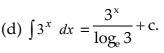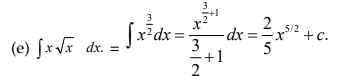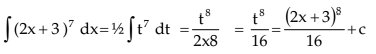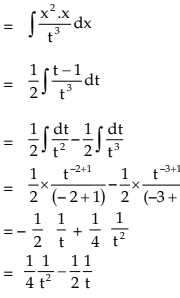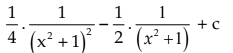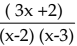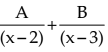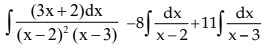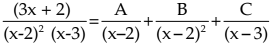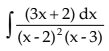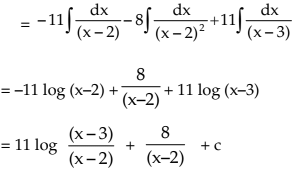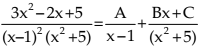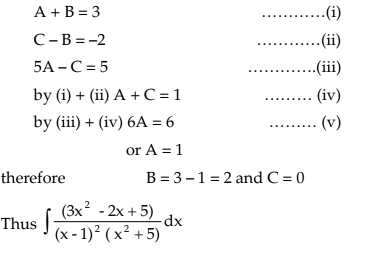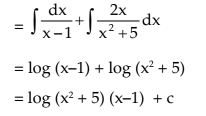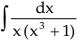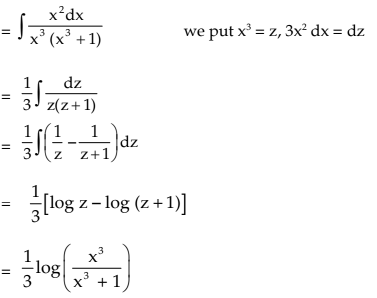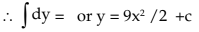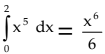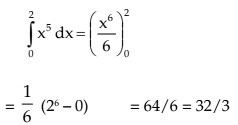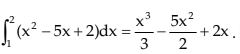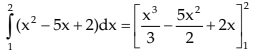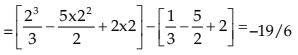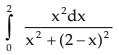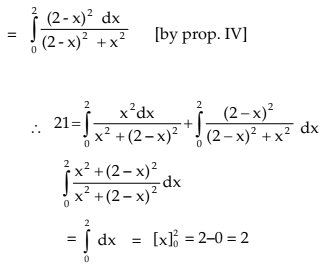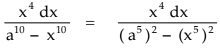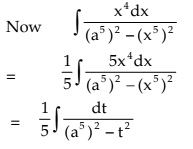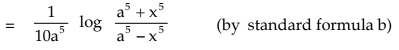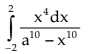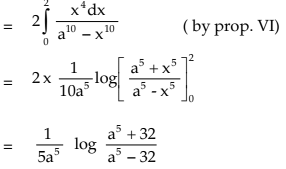Integral Calculus Chapter Notes | Quantitative Aptitude for CA Foundation PDF Download
| Table of contents |

|
| Integration |

|
| Basic Formulas |

|
| Method of Substitution (Change of Variable) |

|
| Integration by Parts |

|
| Method of Partial Fraction |

|
| Definite Integration |

|
| Important Properties of definite Integral |

|
Integration
Integration is the reverse process of differentiation.
We know
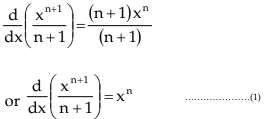
Integration is the inverse operation of differentiation and is denoted by the symbol ∫ .
Hence, from equation (1), it follows that
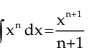
i.e. Integral of xn with respect to variable x is equal to 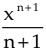
Thus if we differentiate 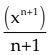 we can get back xn
we can get back xn
Again if we differentiate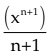 and c being a constant, we get back the same xn .
and c being a constant, we get back the same xn .
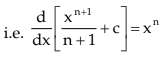
Hence 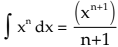 + c and this c is called the constant of integration.
+ c and this c is called the constant of integration.
Integral calculus was primarily invented to determine the area bounded by the curves dividing the entire area into infinite number of infinitesimal small areas and taking the sum of all these small areas.
Basic Formulas
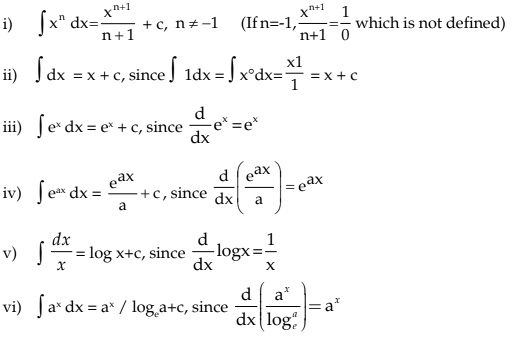
Note: In the answer for all integral sums we add +c (constant of integration) since the differentiation of constant is always zero.
Elementary Rules:
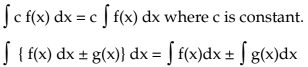
Examples : Find 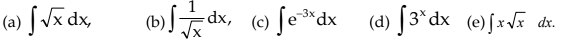
Solution:
Examples : Evaluate the following integral:
(i)
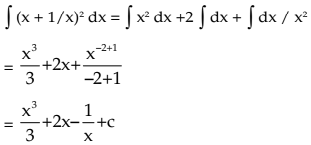
(ii)
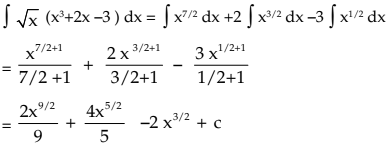
(iii)
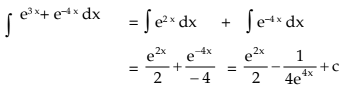
(iv)
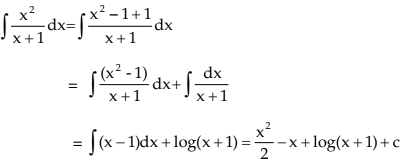
(v)
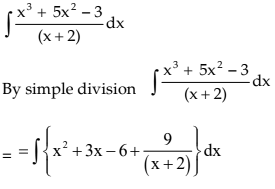
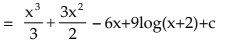
Method of Substitution (Change of Variable)
It is sometime possible by a change of independent variable to transform a function into another which can be readily integrated.We can show the following rules.
To put z = f (x) and also adjust dz = f ' (x) dx
Example:
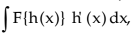 take ez = h(x) and to adjust dz = h'(d) dx
take ez = h(x) and to adjust dz = h'(d) dxthen integrate ∫ F(z) dz using normal rule.
Example: 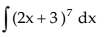
We put (2x + 3) = t
⇒ so 2 dx = dt or dx = dt / 2Therefore
This method is known as Method of Substitution
Example: 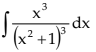
We put (x2 +1) = t
so 2x dx = dt or x dx = dt / 2
=
Important Standard Formulae
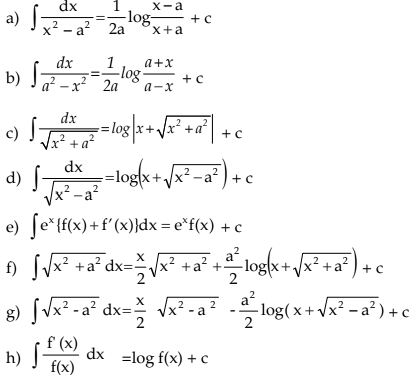
Examples: (a) 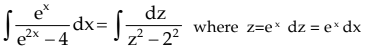
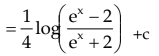
(b) 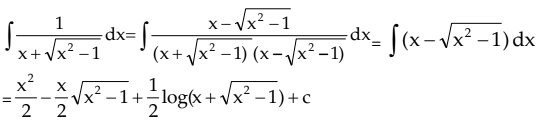
(c)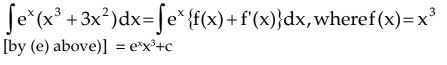
Integration by Parts
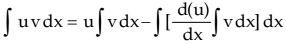
where u and v are two different functions of x
Evaluate:
(i) ∫ xex dx
Integrating by parts we see
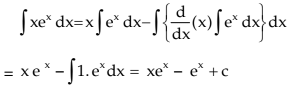
(ii) 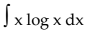
Integrating by parts,
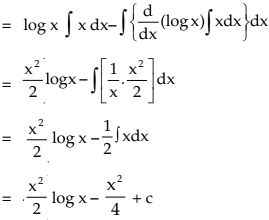
iii)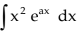
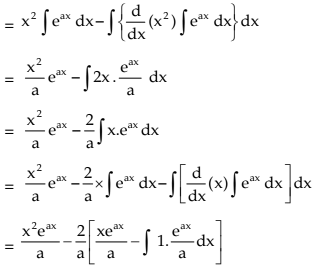
= 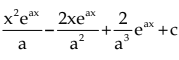
Method of Partial Fraction
Type I :
Example: 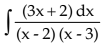
Solution: let
=
[Here degree of the numerator must be lower than that of the denominator; the denominator contains non–repeated linear factor]
so 3x + 2 = A (x – 3) + B (x – 2)
We put x = 2 and get
3.2 + 2 = A (2–3) + B (2–2) ⇒ A = –8 we put x = 3 and get
3.3 +2 = A (3–3) + B (3–2) ⇒ B= 11
= –log(x–2)+11log (x -3) + c
Type II:
Example: 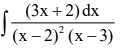
Solution: let
or 3x + 2 = A (x – 2) (x – 3) + B (x – 3) +C (x – 2)2
Comparing coefficients of x2 , x and the constant terms of both sides, we find
A+C = 0 …………(i)
–5A + B – 4C = 3 ……(ii)
6A – 3B + 4C = 2 …….(iii)
By (ii) + (iii) A – 2B = 5 ..…….(iv)
(i) – (iv) 2B + C = –5 …….(v)
From (iv) A = 5 + 2B
From (v) C = –5 – 2B
From (ii) –5 ( 5 + 2B) + B – 4 (– 5 – 2B) = 3 or – 25 – 10B + B + 20 + 8B = 3
or – B – 5 = 3
or B = – 8, A = 5 – 16 = – 11, from (iv) C = – A = 11Therefore
Type III:
Example: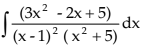
Solution: Let
so 3x2 –2x +5 = A (x2 + 5 ) + (Bx +C) (x–1)
Equating the coefficients of x2 , x and the constant terms from both sides we get
Example: 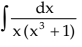
Solution:
Example : Find the equation of the curve where slope at (x, y) is 9x and which passes through the origin.
Solution: dy / dx = 9x
Since it passes through the origin, c = 0; thus required eqn . is 9x2 = 2y.
Definite Integration
Suppose F(x) dx = f (x)As x changes from a to b the value of the integral changes from f (a) to f (b). This is as
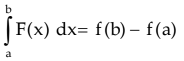
‘b’ is called the upper limit and ‘a’ the lower limit of integration. We shall first deal with indefinite integral and then take up definite integral.
Example: 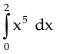
Solution:
Note: In definite integration the constant (c) should not be added
Example: 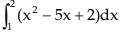
Solution:
Now,
Important Properties of definite Integral
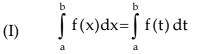
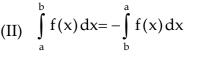
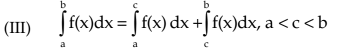
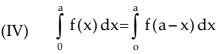
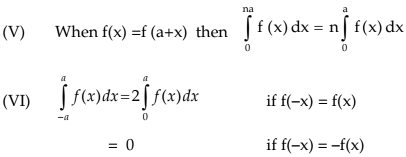
Example: 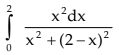
Solution: Let I =
or I = 2/2 = 1
Example: Evaluate 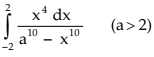
Solution:
let x5 = t so that 5x4 dx = dt
Therefore,
|
101 videos|276 docs|89 tests
|
FAQs on Integral Calculus Chapter Notes - Quantitative Aptitude for CA Foundation
| 1. What are the basic formulas of integration that I should know for my CA Foundation exam? |  |
| 2. How does the method of substitution work in integration? |  |
| 3. Can you explain the method of integration by parts and its formula? |  |
| 4. What is the method of partial fractions, and when is it used? |  |
| 5. What are some important properties of definite integrals that I should remember? |  |