Parabola

The general second-degree equation of a conic is

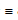ax² + 2hxy + by² + 2gx + 2fy + c = 0

C. Distinguishing between the conics

The nature of a conic depends on the value of the eccentricity e and other invariants of the general second-degree equation such as the determinant D = abc + 2fgh - af² - bg² - ch² and the sign of h² - ab.

Case (i) - Focus lies on the directrix

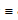When the focus lies on the directrix, the determinant D = abc + 2 fgh - af² - bg² - ch² = 0 and the general equation represents a pair of straight lines. Their nature depends on e:

• e > 1 - the lines are real and distinct, intersecting at the focus S.
• e = 1 - the lines are coincident.
• e < 1 - the lines are imaginary.

Case (ii) - Focus does not lie on the directrix

The conic represents different curves according to values of e and h² - ab:

Conic	e	D	Condition (h2 vs ab)
Parabola	e = 1	0	h2 = ab
Ellipse	0 < e < 1	0	h2 < ab
Hyperbola	e > 1	0	h2 > ab
Rectangular hyperbola	e > 1	0	h2 > ab ; a + b = 0

D. Parabola

A parabola is the locus of a point which moves in a plane such that its distance from a fixed point (the focus) is equal to its perpendicular distance from a fixed straight line (the directrix).

The standard equation of a parabola with axis along the x-axis is y² = 4ax. For this parabola:

• Vertex: (0, 0)
• Focus: (a, 0)
• Axis: y = 0
• Directrix: x + a = 0 (i.e. x = -a)

Important terms for the parabola:

• Focal distance - distance of a point on the parabola from the focus.
• Focal chord - a chord passing through the focus.
• Double ordinate - a chord perpendicular to the axis of symmetry.
• Latus rectum - the focal chord perpendicular to the axis (a double ordinate through the focus).

• Length of the latus rectum = 4a.
• Length of the semi latus rectum = 2a.
• Ends of the latus rectum: L( a, 2a ) and L( a, -2a ).

Notes

• Perpendicular distance from focus to the directrix = half the latus rectum.
• The vertex is the midpoint of the focus and the point where the directrix meets the axis.
• Two parabolas are equal if they have the same latus rectum.

E. Type of parabola

Four standard orientations are:

• y² = 4ax (opens right)
• y² = -4ax (opens left)
• x² = 4ay (opens upward)
• x² = -4ay (opens downward)

F. Parametric representation

A convenient parametric form for the parabola y² = 4ax is

x = at²,   y = 2at, where t is the parameter.

Ex.1 Find the vertex, axis, directrix, focus, latus rectum and the tangent at vertex for the parabola 9y² - 16x - 12y - 57 = 0.

Sol.

The given equation can be rewritten so that it is of the form Y² = 4AX.

Hence the vertex is (-61/16, 2/3).

The axis is y - 2/3 = 0 ⇒ y = 2/3.

The directrix is X + A = 0.

The focus is X = A and Y = 0.

Length of the latus rectum = 4A = 16/9.

The tangent at the vertex is X = 0 ⇒ x = -61/16.

Ex.2 The length of latus rectum of a parabola, whose focus is (2, 3) and directrix is the line x - 4y + 3 = 0 is

Sol.

The length of latus rectum = 2 × perpendicular distance from focus to the directrix.

Ex.3 Find the equation of the parabola whose focus is (-6, -6) and vertex (-2, 2).

Sol.

Let S(-6, -6) be the focus and A(-2, 2) the vertex. Construct the directrix as the perpendicular bisector of SA.

Compute an intermediate point on SA to locate the directrix.

-6 + x₁ = -4 and -6 + y₁ = 4 ⇒ (x₁, y₁) = (2, 10).

Hence the directrix KM is y - 10 = m(x + 2) ....(1)

Gradient of SK is

The directrix equation obtained is x + 2y - 22 = 0.

For any point P(x, y) on the parabola, SP = perpendicular distance from P to the directrix KM.

Thus the locus equation becomes

5(x² + y² + 12x + 12y + 72) = (x + 2y - 22)²

or 4x² + y² - 4xy + 104x + 148y - 124 = 0

or (2x - y)² + 104x + 148y - 124 = 0.

Ex.4 The extreme points of the latus rectum of a parabola are (7, 5) and (7, 3). Find the equation of the parabola

Sol.

Focus is the midpoint of the latus rectum, so S = (7, 4).

The axis is perpendicular to the latus rectum and passes through the focus; its equation is y - 4 = 0.

Length of the latus rectum = 5 - 3 = 2.

The vertex is at distance (latus rectum length)/4 = 0.5 from the focus along the axis.

Two parabolas are possible (concave rightward and leftward):

First: vertex (6.5, 4) ⇒ (y - 4)² = 2(x - 6.5) and it meets the x-axis at (14.5, 0).

Second: vertex (7.5, 4) ⇒ (y - 4)² = -2(x - 7.5) and it meets the x-axis at (-0.5, 0).

G. Position of a point relative to a parabola

For the parabola y² = 4ax, a point (x₁, y₁) lies

• outside the parabola if y₁² - 4ax₁ > 0,
• on the parabola if y₁² - 4ax₁ = 0,
• inside the parabola if y₁² - 4ax₁ < 0.

Ex.5 Find the value of a for which the point (α - 1, α) lies inside the parabola y² = 4x.

Sol.

Point (α - 1, α) lies inside the parabola y² = 4x.

y₁² - 4x₁ < 0 ⇒ α² - 4(α - 1) < 0

⇒ α² - 4α + 4 < 0 ⇒ (α - 2)² < 0 ⇒ α ∈ ∅

Thus there is no real α for which the point lies inside the parabola.

H. Chord joining two points

The chord of the parabola y² = 4ax joining two points with parameters t₁ and t₂ (i.e. points (at₁², 2at₁) and (at₂², 2at₂)) has equation

y(t₁ + t₂) = 2x + 2a t₁ t₂

Note

• If PQ is a focal chord then t₁ t₂ = -1.
• Extremities of a focal chord can be taken as (at², 2at) and (a/t², -2a/t).

Ex.6 Through the vertex O of a parabola y² = 4x chords OP and OQ are drawn at right angles to one another. Show that for all positions of P, PQ cuts the axis of the parabola at a fixed point.

Sol.

The given parabola is y² = 4x ....(1)

Hence the variable line PQ passes through a fixed point which is the intersection of the two fixed lines L₁ = 0 and L₂ = 0, i.e. (4, 0).

I. Line & a parabola

(a) The line y = mx + c meets the parabola y² = 4ax in two points which are real, coincident or imaginary according as the quadratic in x has discriminant >, =, < 0. The condition of tangency is

c = a/m (for m ≠ 0).

Note: For the parabola x² = 4ay, the line y = mx + c is tangent if c = -am².

(b) Length of the chord intercepted by the parabola y² = 4ax on the line y = mx + c is

Note: Length of a focal chord making angle α with the x-axis is 4a cosec² α.

Ex.7 If the line y = 3x + λ intersect the parabola y² = 4x at two distinct points then set of values of λ is

Sol.

Substitute y = 3x + λ into y² = 4x to get

(3x + λ)² = 4x ⇒ 9x² + (6λ - 4)x + λ² = 0.

For two distinct points, discriminant > 0.

Compute discriminant D = (6λ - 4)² - 4·9·λ² = 36λ² - 48λ + 16 - 36λ² = -48λ + 16.

So D > 0 ⇒ -48λ + 16 > 0 ⇒ λ < 1/3.

Therefore λ ∈ (-∞, 1/3).

J. Tangent to the parabola y² = 4ax

(a) Point form: Tangent at (x₁, y₁) is

yy₁ = 2a (x + x₁)

(b) Slope form: Tangent with slope m (m ≠ 0) is

y = mx + a/m, and the point of contact is

(c) Parametric form: Tangent at parameter t is

ty = x + at²

Note: Intersection of tangents at t₁ and t₂ is [a t₁ t₂, a(t₁ + t₂)].

Ex.8 A tangent to the parabola y² = 8x makes an angle of 45º with the straight line y = 3x + 5. Find its equation and its point of contact.

Sol.

Let the slope of the tangent be m.

Equation of tangent to y² = 4ax is y = mx + a/m. For y² = 8x, a = 2, so tangent is y = mx + 2/m.

If it makes 45° with line y = 3x + 5, find m such that angle between slopes m and 3 is 45°.

Solving gives m = -2 or m = 1/2.

For m = -2, tangent: y = -2x - 1 and point of contact is (1/2, -2).

For m = 1/2, tangent: y = (1/2)x + 4 and point of contact is (8, 8).

Ex.9 Find the equation to the tangents to the parabola y² = 9x which go through the point (4, 10).

Sol.

Tangent form: y = mx + 9/(4m).

Since it passes through (4, 10): 10 = 4m + 9/(4m).

Multiply by 4m: 40m = 16m² + 9 ⇒ 16m² - 40m + 9 = 0.

Solve quadratic: m = 1/4 or 9/4.

Ex.10 Find the locus of the point P from which tangents are drawn to the parabola y² = 4ax having slopes m₁ and m₂ such that

where θ₁ and θ₂ are the inclinations of the tangents from positive x-axis.

Sol.

Equation of tangent: y = mx + a/m. If it passes through P(h, k), then m² h - m k + a = 0.

The locus of P(h, k) is of the form y² - 2ax = λx².

K. Director circle

The locus of the point of intersection of perpendicular tangents to the parabola y² = 4ax is called the director circle. For this parabola its equation coincides with the directrix: x + a = 0.

Ex.11 The angle between the tangents drawn from a point (-a, 2a) to y² = 4ax is

Sol.

The point (-a, 2a) lies on the directrix x = -a, so the tangents drawn from it are at right angles.

Ex.12 The circle drawn with variable chord x + ay - 5 = 0 (a being a parameter) of the parabola y² = 20x as diameter will always touch the line

Sol.

The line x + ay - 5 = 0 always passes through the focus (5, 0) of y² = 20x. Thus the circle with this chord as diameter will always touch the directrix x + 5 = 0.

L. Normal to the parabola y² = 4ax

(a) Point form: Equation of the normal at (x₁, y₁) is

(b) Slope form: Normal with slope m is

y = mx - 2am - a m³ and the foot of this normal is (am², -2am).

(c) Parametric form: Normal at parameter t is

y + tx = 2at + at³

Notes

• Point of intersection of normals at t₁ and t₂ is given by a formula (see image):
  
• If normal at t₁ meets the parabola again at t₂, then
  
• If normals at t₁ and t₂ meet again on the parabola at t₃ then t₁ t₂ = 2; t₃ = -(t₁ + t₂), and the line joining t₁ & t₂ passes through fixed point (-2a, 0).
• If a normal to the parabola passes through a fixed point P(h, k), then k = mh - 2am - am³, i.e. am³ + m(2a - h) + k = 0.

For three concurrent normals with slopes m₁, m₂, m₃ we have

m₁ + m₂ + m₃ = 0

and algebraic sum of ordinates of the three co-normal points is zero. The centroid of the triangle formed by the three co-normal points lies on the axis of the parabola.

Ex.13 Prove that the normal chord to a parabola y² = 4ax at the point whose ordinate is equal to abscissa subtends a right angle at the focus.

Sol.

Let the normal at P (at₁², 2at₁) meet the curve at Q (a t₂², 2 a t₂). Then for a normal chord PQ we have t₂ = -t₁ - 2/t₁.

Given 2at₁ = a t₁² ⇒ t₁ = 2.

Then t₂ = -3, so P(4a, 4a), Q(9a, -6a), and focus S(a, 0).

Using vector/distance or dot product it follows that the angle at S is 90°.

Ex.14 If two normals drawn from any point to the parabola y² = 4ax make angles α and β with the axis such that tan α · tan β = 2, then find the locus of this point.

Sol.

Let the point be (h, k). Equation of a normal is y = mx - 2am - am³, passing through (h, k) gives am³ + m(2a - h) + k = 0.

Let m₁, m₂, m₃ be roots; using symmetric relations and condition tan α · tan β = 2 leads to k² = 4ah.

Thus the locus is y² = 4ax.

Ex.15 Three normals are drawn from the point (14, 7) to the curve y² - 16x - 8y = 0. Find the coordinates of the feet of the normals.

Sol.

Write parabola: y² - 16x - 8y = 0 .....(i)

Let foot of normal be P(α, β). Equation of tangent at P to (i) is

β y - 8(x + α) - 4(y + β) = 0 ⇒ (β - 4)y = 8x + 8α + 4β ...(ii)

Slope of tangent = 8/(β - 4).

Equation of normal at P then is y - β = -(β - 4)/8 (x - α).

It passes through (14, 7); substitute and simplify with the condition that (α, β) lies on parabola β² - 16α - 8β = 0.

Elimination and simplification give β(β - 16)(β + 4) = 0 ⇒ β = 0, 16, -4.

Corresponding α: when β = 0 ⇒ α = 0; when β = 16 ⇒ α = 8; when β = -4 ⇒ α = 3.

Hence feet of normals are (0, 0), (8, 16) and (3, -4).

M. Length of subtangent & subnormal

At point P(x, y) on the parabola y² = 4ax, let PT be tangent and PG be normal. Then:

Length of subtangent = TN = 2 × abscissa of P (i.e. 2x_P).

Length of subnormal = NG = constant for the parabola and equal to its semi latus rectum = 2a.

N. Pair of tangents

The equation of the pair of tangents which can be drawn from an external point P(x₁, y₁) to the parabola y² = 4ax is given by

S S₁ = T₂

where S ≡ y² - 4ax, S₁ ≡ y₁² - 4ax₁, and T ≡ yy₁ - 2a(x + x₁).

O. Chord of contact

Equation of the chord of contact of tangents drawn from P(x₁, y₁) is

yy₁ = 2a(x + x₁)

Area of the triangle formed by the two tangents from (x₁, y₁) and their chord of contact is given by an expression (see image):

Note: chord of contact exists only if P is not inside the parabola.

Ex.16 If the line x - y - 1 = 0 intersect the parabola y² = 8x at P & Q, then find the point of intersection of tangents at P & Q

Sol.

Let (h, k) be the intersection point of tangents; the chord of contact is

yk = 4(x + h) ⇒ 4x - yk + 4h = 0 ............(i)

Given line: x - y - 1 = 0 ............(ii)

Compare (i) and (ii) to obtain h = -1, k = 4.

Therefore intersection point is (-1, 4).

Ex.17 Find the locus of point whose chord of contact w.r.t. the parabola y² = 4bx is the tangent of the parabola y² = 4ax

Sol.

Equation of tangent to y² = 4ax is y = mx + a/m ....(1)

If it is chord of contact for y² = 4bx with respect to point P(h, k), then chord of contact is yk = 2b(x + h).

From (1) and chord form compare coefficients and eliminate m to find the locus (details in images):

P. Chord with a given middle point

Equation of the chord of the parabola y² = 4ax whose midpoint is (x₁, y₁) is

y - y₁ = (2a / y₁) (x - x₁)

This reduces to T = S₁ where T ≡ yy₁ - 2a(x + x₁) and S₁ ≡ y₁² - 4ax₁.

Ex.18 Find the locus of middle of the chord of the parabola y² = 4ax which pass through a given (p, q).

Sol.

Let P(h, k) be the midpoint of the chord. Equation of the chord: yk - 2a(x + h) = k² - 4ah.

Since the chord passes through (p, q): qk - 2a(p + h) = k² - 4ah.

Simplify to obtain the locus: y² - 2ax - qy + 2ap = 0.

Ex.19 Find the locus of the middle point of a chord of a parabola y² = 4ax which subtends a right angle at the vertex.

Sol.

Let the middle point be (α, β). The chord with this midpoint has equation (see image for derivation):

Using homogeneization and condition that the two lines from origin to the chord's intersections are perpendicular, one obtains

β² = 2a(α - 4a), i.e. locus is y² = 2a(x - 4a).

Q. An important concept

If a family of straight lines is given by λ²P + λ Q + R = 0 (λ is parameter and P, Q, R are linear functions of x,y), then the family of lines is tangent to the curve

Q² = 4PR

Ex.20 If the equation m²(x + 1) + m(y - 2) + 1 = 0 represents a family of lines, where 'm' is parameter then find the equation of the curve to which these lines will always be tangents.

Sol.

Consider quadratic in m: m²(x + 1) + m(y - 2) + 1 = 0. For tangency, discriminant = 0.

(y - 2)² - 4(x + 1) = 0 ⇒ y² - 4y + 4 - 4x - 4 = 0.

Thus y² = 4(x + y).

R. Diameter

The locus of midpoints of a system of parallel chords of a parabola is called a diameter. For chords with slope m of y² = 4ax, the equation of the diameter is y = (2a)/m.

Ex.21 The common tangent of the parabola y² = 8ax and the circle x² + y² = 2a² is

Sol.

Any tangent to parabola: y = mx + 2a/m.

Solving for m gives m = ±1. Therefore tangents: y = ±x ± a (see images for sign and constant verification).

Ex.23 If P(-3, 2) is one end of the focal chord PQ of the parabola y² + 4x + 4y = 0, then the slope of the normal at Q is

Sol.

Tangent at (-3, 2) to y² + 4x + 4y = 0 is 2y + 2(x - 3) + 2(y + 2) = 0 ⇒ 2x + 4y - 2 = 0 ⇒ x + 2y - 1 = 0.

Since tangent at one end of a focal chord is parallel to the normal at the other end, the slope of the normal at Q equals slope of this tangent = -1/2.

Ex.25 If r₁, r₂ be the length of the perpendicular chords of the parabola y² = 4ax drawn through the vertex, then show that

Sol.

If one chord makes angle θ with x-axis, the other makes (90° - θ). Let AP = r₁ and AQ = r₂.

Coordinates: P = (r₁ cos θ, r₁ sin θ), Q = (r₂ sin θ, -r₂ cos θ).

Since P and Q lie on y² = 4ax, substitute and simplify (details and algebra shown in images):

S. Important highlights of parabola

Key results and geometric properties that are important to remember for the parabola y² = 4ax:

• If the tangent and normal at P meet the axis at T and G respectively, then ST = SG = SP where S is the focus. Tangent and normal at P bisect the angle between SP and the perpendicular from P to the directrix. Therefore rays from the focus reflect into rays parallel to the axis.
• The portion of a tangent cut off between the directrix and the curve subtends a right angle at the focus.
• Tangents at the extremities of a focal chord intersect at right angles on the directrix; a circle on any focal chord as diameter touches the directrix. A circle on any focal radius as diameter touches the tangent at the vertex and intercepts a chord on the normal (see image):
  
• Any tangent to a parabola and the perpendicular from the focus to it meet on the tangent at the vertex.
• If tangents at P and Q meet at T, then TP and TQ subtend equal angles at the focus S; ST² = SP · SQ; triangles SPT and STQ are similar (proof provided in images).
• Tangents and normals at the extremities of the latus rectum of y² = 4ax form a square whose intersection points are (-a, 0) and (3a, 0).
• Semi latus rectum of y² = 4ax is the harmonic mean between segments of any focal chord (detailed relation in image):
  
• The circle circumscribing the triangle formed by any three tangents to a parabola passes through the focus.
• The orthocentre of any triangle formed by three tangents lies on the directrix (proof and derivation in images).
• The area of the triangle formed by three points on the parabola is twice the area of the triangle formed by tangents at those points (proof in images).
• A circle circumscribing the triangle formed by three co-normal points (normals concurrent at (h, k)) passes through the vertex; its equation is 2(x² + y²) - 2(h + 2a)x - ky = 0.

(Proofs and algebra for many of the above results are provided through the worked examples and image references in the relevant sections.)

All image placeholders