Limits, Chapter Notes, Class 11, Maths PDF Download
Introduction to Limits - Limits and Derivatives, Class 11, Maths
A. Definition of Limit
Let f be a function defined on an open interval containing c (except possibly at c) and let L be a real number. The statement 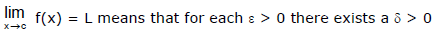 such that if
such that if
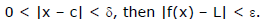
B. The Existence of a Limit
Let f be a function and let c and L be real numbers. The limit of f(x) as x approaches c is L if and only if
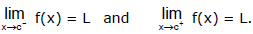
In other words limit of a function f(x) is said to exist as, x→a when
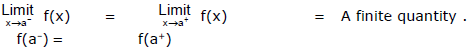


Ex.1 The graph of a function g is shown in the figure. Use it to state the values (if they exist) of the following
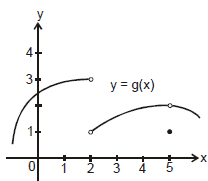
(a) 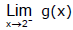
(b) 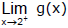
(c) 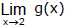
(d) 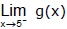
(e) 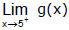
(f) 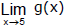
Sol. From the graph we see that the values of g(x) approach 3 as x approaches 2 from the left, but they approach 1 as x approaches 2 from the right. Therefore
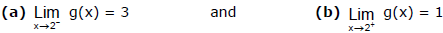
(c) Since the left and right limits are different, we conclude that  g(x) does not exist.
g(x) does not exist.
The graph also show that
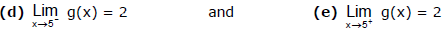
(f) This time the left and right limits are the same and so, we have 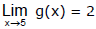
Despite this fact, notice that 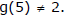
Ex.2 If f(x) = 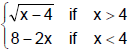 determine whether
determine whether 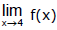 exists.
exists.
Sol.

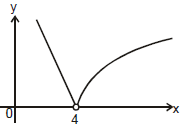
The right and left-hand limits are equal. Thus, the limit exists and 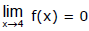
The graph of f is shown in the figure.
Ex.3 Evaluate 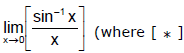 denotes the greatest integer function)
denotes the greatest integer function)
Sol.
Let P = 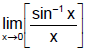
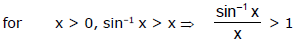
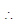 R.H.L. =
R.H.L. = 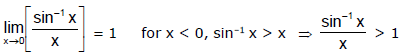
∴ L.H.L. = 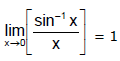 Hence P = 1.
Hence P = 1.
C. fundamental theorems on limits
Let b and c be real numbers, let n be a positive integer, and let f and g be functions with the following limits. 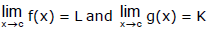
1. Sum Rule : 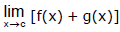 = L + K
= L + K
2. Difference Rule : 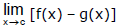 = L - K
= L - K
3. Product Rule : 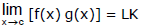
4. Quotient Rule : 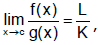 provided
provided 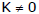
5. Constant Multiplication Rule : 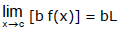
6. Composition Rule : 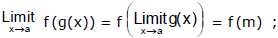 provided f is continuous at x = m.
provided f is continuous at x = m.
For example 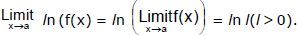
Ex.4 Evaluate the following limits and justify each step.
(a) 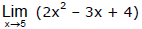
(b) 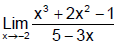
Sol. (a)
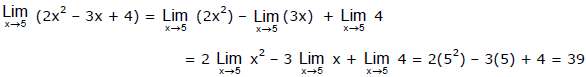
(b) We start by using laws of limit, but their use is fully justified only at the final stage when we see that the limits of the numerator and denominator exist and the limit of the denominator is not 0.
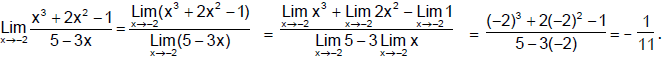
D. Non-existence of limit
Three of the most common types of behaviour associated with the non-existence of a limit.
1. f(x) approaches a different number from the right side of c than it approaches from the left side.
2. f(x) increases or decreases without bound as x approaches c.
3. f(x) oscillates between two fixed values as x approaches c.
There are many other interesting functions that have unusual limit behaviour. An often cited one is the Dirichlet function f(x) = 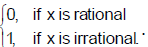 This function has no limit at any real number c.
This function has no limit at any real number c.
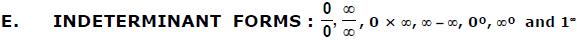
Ex.5 Which of the following limits are in indeterminant forms. Also indicate the form
(i) 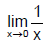
(ii) 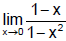
(iii) 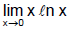
(iv) 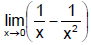
(v) 
(vi) 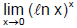
(vii) 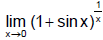
(viii) 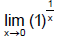
Sol. (i) No
(ii) Yes  form
form
(iii) Yes 0 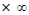 form
form
(iv) Yes (∞ - ∞) form
(v) Yes, 00 form
(vi) Yes ∞0 form
(vii) Yes 1∞ form
(viii) No
Remark :
(i) `0' doesn't means exact zero but represent a value approaching towards zero similarly to `1' and infinity.
(ii) ∞ + ∞ = ∞
(iii) ∞ × ∞ = ∞
(iv) (a/∞) = 0 if a is finite
(v)  is not defined for any a ε R.
is not defined for any a ε R.
(vi) a b = 0, if & only if a = 0 or b = 0 and a & b are finite.
F. Methods of evaluating limits
(Rationalization, Factorization and Cancellation of Common Factors)
Ex.6 Evaluate 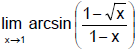
Sol. 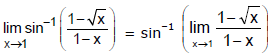 =
= 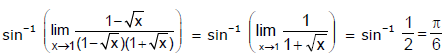
Ex.7 Evaluate 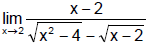
Sol.
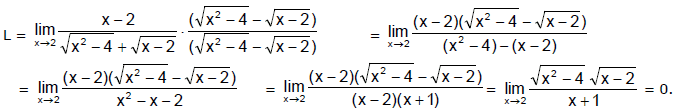
Ex.8 Evaluate 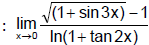
Sol.
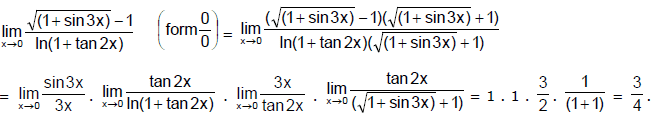
Ex.9 Evaluate 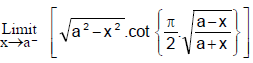 .
.
Sol.
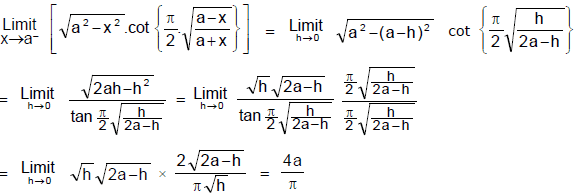
Ex.10 Find 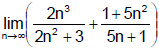
Sol. 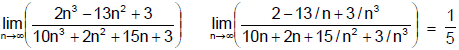
Ex.11 Find 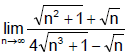
Sol.
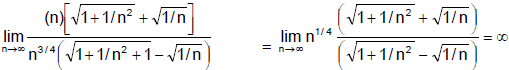
Ex.12 Find 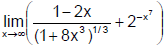
Sol.
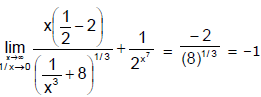
Ex.13 Evaluate 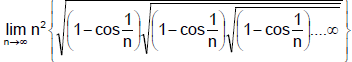
Sol.
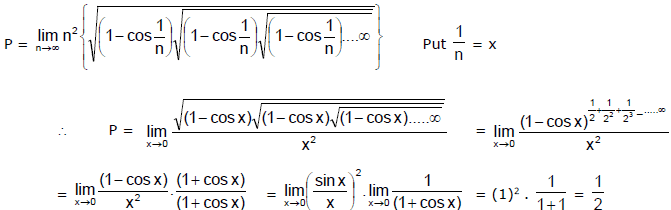
G. standard theorem
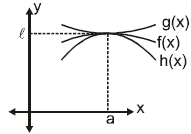
(1) Sandwich Theorem / Squeeze Play Theorem
Statement : If 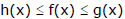 for all x in an open interval containing c, except possibly at c itself, and if
for all x in an open interval containing c, except possibly at c itself, and if
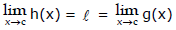
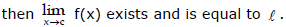
Ex.14 Find 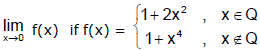
Sol.
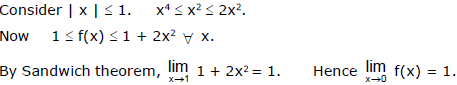
Ex.15 Let a function f(x) be such that 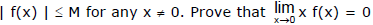
Sol.
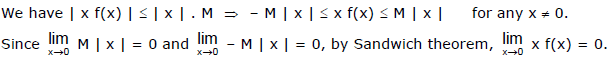
Ex.16 Use Sandwich theorem to evaluate: 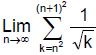
Sol.
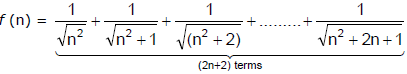
terms of the sequence are decreasing and number of terms are (2n + 2)
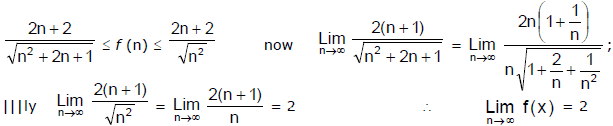
FAQs on Limits, Chapter Notes, Class 11, Maths
| 1. What is a limit in calculus? |  |
| 2. How do you evaluate a limit? |  |
| 3. What is the difference between a left and right-hand limit? |  |
| 4. What is the importance of limits in real-life applications? |  |
| 5. How do you use limits to calculate derivatives? |  |



















