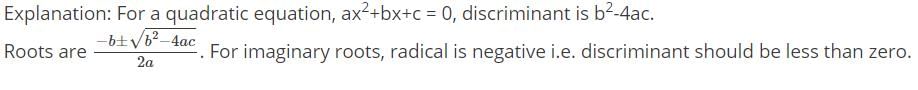CAT Quadratic Equations : Basic Concepts and Notes PDF
| Table of contents |

|
| PolynomialsDefinition of a Polynomial |

|
| Remainder Theorem |

|
| Factor Theorem |

|
| General Theory of Equations |

|
| Linear Equation |

|
| Quadratic Equations |

|
Polynomials

Definition of a Polynomial
Let
be real numbers and x is a real variable. Then f(x) =
is called a real polynomial of real variable x with coefficients
Example:  is a polynomial
is a polynomial
Degree of a Polynomial
The degree of a polynomial is the highest power of the variable in the polynomial.
- Example: Degree of
 is 3 as the maximum power of the variable x is 3.
is 3 as the maximum power of the variable x is 3. - On the basis of degree, the polynomials are classified as Linear (Degree 1), Quadratic (degree 2), Cubical (Degree 3), bi-Quadratic (degree 4), and so on.
Examples on Degree of a Polynomial
Q.1. Find the degree of the polynomial 6x4+ 3x2+ 5x +19
Ans: The degree of the polynomial is 4.
Q.2. Find the degree of the polynomial 6x3+4x3+3x+1
Ans: The degree of the polynomial is 3.
Remainder Theorem
If any polynomial f(x) is divided by (x - a) then f(a) is the remainder.
- Example: f(x) = x2 - 5x + 7 = 0 is divided by x - 2. What is the remainder?
R = f(2) = 22 - 5 × 2 + 7 = 1.
Factor Theorem
If (x - a) is a factor of f(x), then remainder f(a) = 0. (Or) if f(a) = 0, then (x - a) is a factor of f(x),
- Example: When f(x) = x2 - 5x + 6 = 0 is divided by x - 2, the remainder f(2) is zero which shows that x - 2 is the factor of f(x)
Examples on Remainder & Factor Theorem
Q.1. Find the remainder by using the remainder theorem when polynomial x3−3x2+x+1 is divided by x−1.
Ans:

Q.2. Find the remainder when p(x)= −x+3x2−1 is divided by x+1.
Ans:
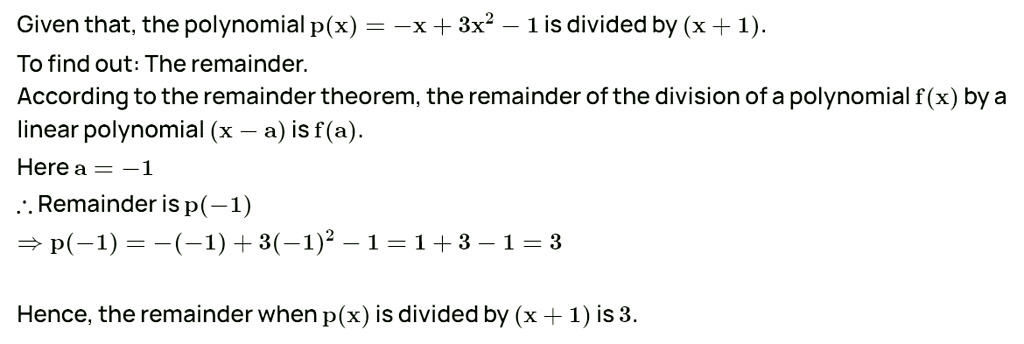
General Theory of Equations
- An equation is the form of a polynomial which has been equated to some real value.
- Example: 2x + 5 = 0, x2 - 2x + 5 = 7, 2x2 - 5x2 + 1 = 2x + 5 etc. are polynomial equations.
Root or Zero of a Polynomial Equation
- If f (x) = 0 is a polynomial equation and f (α) = 0, then α is called a root or zero of the polynomial equation f(x) = 0.
Linear Equation
Linear Equation with One Variable
A linear equation is 1st degree equation. It has only one root. Its general form is a
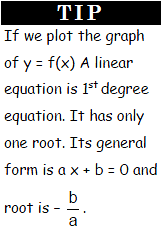
Linear Equation with Two Variables
- Example: 2x + 3y = 0. We need two equations to find the values of x and y.
If there are n variables in an equation, we need n equations to find the values of the variables uniquely. - Sometimes, even the number of equations are equal to the number of variables, we cannot find the values of x and y uniquely.
For example, 3x + 5y = 6 & 6x +10y = 12 - These are two equations, but both are one and the same. So different values of x and y satisfy the equation and there is no unique solution. It will has infinite number of solutions.
- The number of solutions is clearly described below for the set of equations with 2 variables.

- These equations can be:
(i) Inconsistent means have no solution if
(ii) Consistent and has infinitely many solutions if
(iii) Consistent and have unique solution if
Quadratic Equations
Quadratic Equation in “x” is one in which the highest power of “x” is 2. The equation is generally satisfied by two values of “x”.
- The quadratic form is generally represented by ax2 + bx + c = 0 where a ≠ 0, and a, b, c are constants.
- For Example:
x2 - 6x + 4 = 0
3x2 + 7x - 2 = 0 - A quadratic equation in one variable has two and only two roots, which are


Nature of Roots
- The two roots of any quadratic equation always depend on the value of b2 - 4ac called discriminant (D).
(i) D > 0 Real and unequal roots
(ii) D = 0 Real and equal
(iii) D < 0 Imaginary and unequal
Sum and Product of Roots
- If α and β are the two roots of ax2 + bx + c = 0,
- Then sum of roots = α + β =

- And product of roots = αβ =

Formation of Equation from Roots
- If α and β are the roots of any quadratic equation then that equation can be written in the form: X2 − (α + β)X + αβ = 0
i.e. X2 - (sum of the roots) X + Product of the roots = 0
Some Important Results



-----------------------------------------------

Maximum and Minimum value of a Quadratic Equation


-----------------------------------------------

The quadratic equation ax2 + bx + c = 0 will have maximum or minimum value at x = - b/2a. If a < 0, it has maximum value and if a > 0, it has minimum value.
The maximum or minimum value is given by 
Solved Examples on Quadratic Equation

Ex.2. A and B went to a hotel paid Rs. 84 for 3 plates of Idli and 5 plates of Dosa. Whereas B took 5 plates of Idli and 3 plates of Dosa and paid Rs. 76. What is the cost of one plate of Idli.
- 3I + 5D = 84 ……….(1)
- 5I + 3D = 76 ………(2)
- Equation (1) × 3 - equation (2) × 5, we get
⇒ 16I = 128
⇒ I = 8- Each plate of Idli cost Rs. 8.
Ex.3. Find the values of x and y from the equations 
Ex.4. Find x and y from 
Ex.5. Aman won a competition and so he got some prize money. He gave Rs. 2000 less than the half of prize money to his son and Rs. 1000 more than the two third of the remaining to his daughter. If both they got same amount, what is the prize money Aman got?
Ex.6. How many non negative integer pairs (x, y) satisfy the equation, 3x + 4y = 21?
- Since x and y are non negative integers. Start from x = 0.
- If x = 0 or 2, y cannot be integer.
- For x = 3, y = 3.
And for x = 7, y = 0.- These two pairs only satisfy the given equation.
Ex.7. If (x - 2) is a factor of x3 - 3x2 + px + 4. Find the value of p.
- Since (x - 2) is a factor, f(2) = 0.
- ∴ 23 - 3(22) + (2)p + 4 = 0
⇒ p = 0
Ex.8. When x3 - 7x2 + 3x - P is divided by x + 3, the remainder is 4, then what is the value of P ?
- f(- 3) = 4
- ∴ (- 3)3 - 7(- 3)2 + 3(- 3) - P = 4
- P = - 103.
Ex.9. If (x - 1) is the HCF of (x3 - px2 + qx - 3) and (x3 - 2x2 + px + 2). What is the value of ‘q’?
- Since (x - 1) is HCF, it is a factor for both the polynomials.
- ∴ 13 - p(1)2 + q(1) - 3 = 0
- - p + q = 2
- And 13 - 2(12) + p(1) + 2 = 0
- p = - 1
- ∴ q = 1
Ex.10. Find the roots of the quadratic equation x2 - x - 12 = 0.
- x2 - x - 12 = 0
- x2 - 4x + 3x - 12 = 0
- x(x - 4) + 3(x - 4) = 0
- (x - 4) (x + 3) = 0
- x = 4 or -3
- The roots are 4 and -3.
|
115 videos|106 docs|113 tests
|
FAQs on CAT Quadratic Equations : Basic Concepts and Notes PDF
| 1. What is the definition of a polynomial? |  |
| 2. What is the Remainder Theorem? |  |
| 3. What is the Factor Theorem? |  |
| 4. What is the General Theory of Equations? |  |
| 5. What are linear equations and how are they different from quadratic equations? |  |

|
Explore Courses for GMAT exam
|

|