CAT Exam > CAT Notes > Quantitative Aptitude (Quant) > Boats and Streams - Important Formulas, Logical Reasoning
Boats and Streams - Important Formulas, Logical Reasoning | Quantitative Aptitude (Quant) - CAT PDF Download
Important Formulas - Boats and Streams
1. Downstream
In water, the direction along the stream is called downstream.
2. Upstream
In water, the direction against the stream is called upstream.
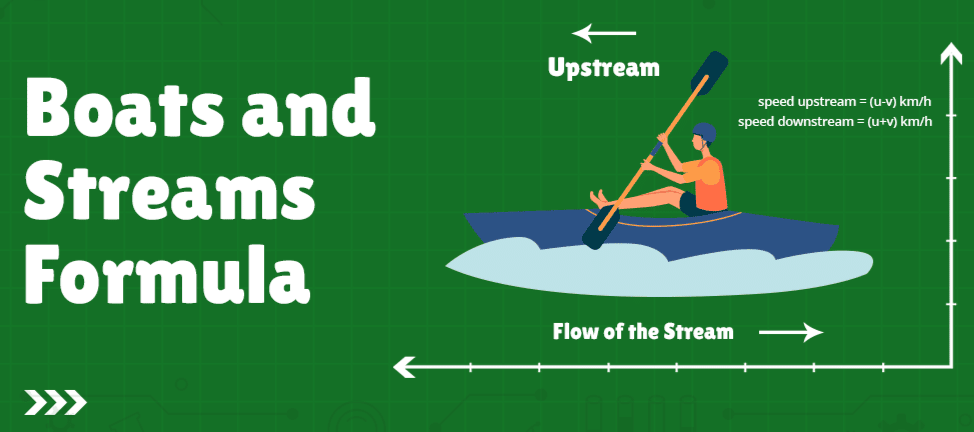
3. Let the speed of a boat in still water be u km/hr and the speed of the stream be v km/hr, then
Speed downstream = (u + v) km/hr
Speed upstream = (u - v) km/hr.
4. Let the speed downstream be a km/hr and the speed upstream be b km/hr, then 
Some more shortcut methods
5. Assume that a man can row at the speed of x km/hr in still water and he rows the same distance up and down in a stream which flows at a rate of y km/hr. Then his average speed throughout the journey
6. Let the speed of a man in still water be x km/hr and the speed of a stream be y km/hr. If he takes tt hours more in upstream than to go downstream for the same distance, the distance 
7. A man rows a certain distance downstream in t1 hours and returns the same distance upstream in t2 hours. If the speed of the stream is y km/hr, then the speed of the man in still water 
8. A man can row a boat in still water at x km/hr in a stream flowing at y km/hr. If it takes him tt hours to row a place and come back, then the distance between the two places 
9. A man takes nn times as long to row upstream as to row downstream the river. If the speed of the man is x km/hr and the speed of the stream is y km/hr, then 
The document Boats and Streams - Important Formulas, Logical Reasoning | Quantitative Aptitude (Quant) - CAT is a part of the CAT Course Quantitative Aptitude (Quant).
All you need of CAT at this link: CAT
|
191 videos|131 docs|110 tests
|
FAQs on Boats and Streams - Important Formulas, Logical Reasoning - Quantitative Aptitude (Quant) - CAT
| 1. What are the important formulas to solve problems related to Boats and Streams? |  |
Ans. The important formulas to solve problems related to Boats and Streams are:
1. Speed of a boat in still water = (Speed of downstream + Speed of upstream) / 2
2. Speed of a boat in still water = (Speed of downstream - Speed of upstream) / 2
3. Speed of downstream = Speed of boat in still water + Speed of stream
4. Speed of upstream = Speed of boat in still water - Speed of stream
5. Time taken to cover a certain distance downstream = Distance / Speed of downstream
6. Time taken to cover a certain distance upstream = Distance / Speed of upstream
| 2. How can logical reasoning be applied to solve problems related to Boats and Streams? | |
Ans. Logical reasoning can be applied to solve problems related to Boats and Streams by analyzing the given information and making logical deductions. Here are a few steps to solve such problems using logical reasoning:
1. Read the problem carefully and understand the given information.
2. Identify the variables involved, such as the speed of the boat, speed of the stream, and the distance.
3. Use the given information to form equations or inequalities.
4. Make logical deductions based on the equations to find the required solution.
5. Verify the solution by checking if it satisfies all the given conditions.
| 3. How can the concept of Boats and Streams be applied in real-life scenarios? | |
Ans. The concept of Boats and Streams can be applied in real-life scenarios in various ways. Here are a few examples:
1. Calculating the time taken by a boat to travel downstream or upstream in a river.
2. Determining the speed at which a boat should travel to cross a river in the shortest time.
3. Calculating the speed of a boat in still water based on the time taken to travel a certain distance downstream or upstream.
4. Analyzing the effect of the speed of a stream on the overall speed of a boat.
5. Solving problems related to rowing, where the speed of a person rowing against or with a stream is involved.
| 4. What are some common mistakes to avoid while solving problems related to Boats and Streams? | |
Ans. Some common mistakes to avoid while solving problems related to Boats and Streams are:
1. Misinterpreting the given information or not understanding the question properly.
2. Using incorrect formulas or equations.
3. Forgetting to consider the relative speeds of the boat and the stream while calculating the overall speed.
4. Making calculation errors or not simplifying the equations properly.
5. Not checking the solution against the given conditions or constraints.
| 5. How can I improve my problem-solving skills related to Boats and Streams? | |
Ans. To improve your problem-solving skills related to Boats and Streams, you can follow these tips:
1. Practice solving a variety of problems related to Boats and Streams regularly.
2. Understand the underlying concepts and formulas thoroughly.
3. Break down complex problems into smaller, manageable parts.
4. Develop a systematic approach to solve problems, such as identifying variables, forming equations, and making logical deductions.
5. Review your solutions and analyze any mistakes or areas for improvement.
6. Seek additional resources, such as textbooks or online tutorials, to enhance your understanding of the topic.
7. Collaborate with peers or join study groups to discuss and solve problems together.
Related Exams
About this Document
|
4.1K Views |
|
4.98/5 Rating |
|
Dec 22, 2024 Last updated |
Document Description: Boats and Streams - Important Formulas, Logical Reasoning for CAT 2024 is part of Quantitative Aptitude (Quant) preparation.
The notes and questions for Boats and Streams - Important Formulas, Logical Reasoning have been prepared according to the CAT exam syllabus. Information about Boats and Streams - Important Formulas, Logical Reasoning covers topics
like and Boats and Streams - Important Formulas, Logical Reasoning Example, for CAT 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Boats and Streams - Important Formulas, Logical Reasoning.
Introduction of Boats and Streams - Important Formulas, Logical Reasoning in English is available as part of our Quantitative Aptitude (Quant)
for CAT & Boats and Streams - Important Formulas, Logical Reasoning in Hindi for Quantitative Aptitude (Quant) course.
Download more important topics related with notes, lectures and mock test series for CAT
Exam by signing up for free. CAT: Boats and Streams - Important Formulas, Logical Reasoning | Quantitative Aptitude (Quant) - CAT
Description
Full syllabus notes, lecture & questions for Boats and Streams - Important Formulas, Logical Reasoning | Quantitative Aptitude (Quant) - CAT - CAT | Plus excerises question with solution to help you revise complete syllabus for Quantitative Aptitude (Quant) | Best notes, free PDF download
Information about Boats and Streams - Important Formulas, Logical Reasoning
In this doc you can find the meaning of Boats and Streams - Important Formulas, Logical Reasoning defined & explained in the simplest way possible. Besides explaining types of
Boats and Streams - Important Formulas, Logical Reasoning theory, EduRev gives you an ample number of questions to practice Boats and Streams - Important Formulas, Logical Reasoning tests, examples and also practice CAT
tests
|
Explore Courses for CAT exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
study material
,practice quizzes
,Previous Year Questions with Solutions
,Logical Reasoning | Quantitative Aptitude (Quant) - CAT
,Exam
,Summary
,Extra Questions
,Semester Notes
,Logical Reasoning | Quantitative Aptitude (Quant) - CAT
,Boats and Streams - Important Formulas
,Free
,Important questions
,Logical Reasoning | Quantitative Aptitude (Quant) - CAT
,Boats and Streams - Important Formulas
,ppt
,mock tests for examination
,Objective type Questions
,MCQs
,video lectures
,past year papers
,Viva Questions
,shortcuts and tricks
,Sample Paper
,Boats and Streams - Important Formulas
;
Additional Information about Boats and Streams - Important Formulas, Logical Reasoning for CAT Preparation
Boats and Streams - Important Formulas, Logical Reasoning Free PDF Download
The Boats and Streams - Important Formulas, Logical Reasoning is an invaluable resource that delves deep into the core of the CAT exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Boats and Streams - Important Formulas, Logical Reasoning now and kickstart your journey towards success in the CAT exam.
Importance of Boats and Streams - Important Formulas, Logical Reasoning
The importance of Boats and Streams - Important Formulas, Logical Reasoning cannot be overstated, especially for CAT aspirants.
This document holds the key to success in the CAT exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Boats and Streams - Important Formulas, Logical Reasoning Notes
Boats and Streams - Important Formulas, Logical Reasoning Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Boats and Streams - Important Formulas, Logical Reasoning.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Boats and Streams - Important Formulas, Logical Reasoning Notes on EduRev are your ultimate resource for success.
Boats and Streams - Important Formulas, Logical Reasoning CAT Questions
The "Boats and Streams - Important Formulas, Logical Reasoning CAT Questions" guide is a valuable resource for all aspiring students preparing for the
CAT exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Boats and Streams - Important Formulas, Logical Reasoning on the App
Students of CAT can study Boats and Streams - Important Formulas, Logical Reasoning alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Boats and Streams - Important Formulas, Logical Reasoning,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Boats and Streams - Important Formulas, Logical Reasoning is prepared as per the latest CAT syllabus.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up
within 7 days!
within 7 days!
Takes less than 10 seconds to signup