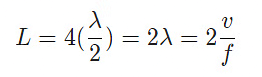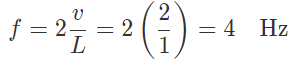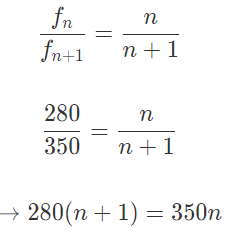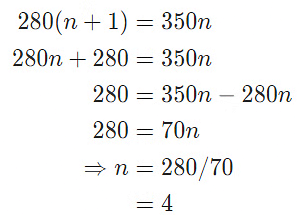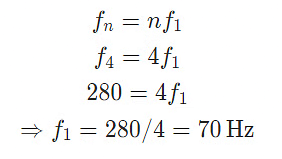Reflection of Waves & Standing Waves | Physics for JAMB PDF Download
| Table of contents |

|
| Reflection of Waves |

|
| Standing Waves & Normal Modes |

|
| Stretched String fixed at Both Ends |

|
| Air Column with One End Closed |

|
Reflection of Waves
- Reflection of a wave happens when it changes direction at the point where two different materials meet, bouncing back into the material it came from.
- When this happens, some of the wave's energy is taken in by the material it hits, especially if the surface isn't smooth. This bouncing follows a rule called the Law of Reflection.
- Imagine a wave moving along a rope tied to a surface; when it hits the surface, it pushes against it, causing the surface to push back with an equal force.
- This interaction creates a new wave, moving in the opposite direction. Reflection occurs with all kinds of waves, like sound, light, and radio waves, and it's useful in many different situations.
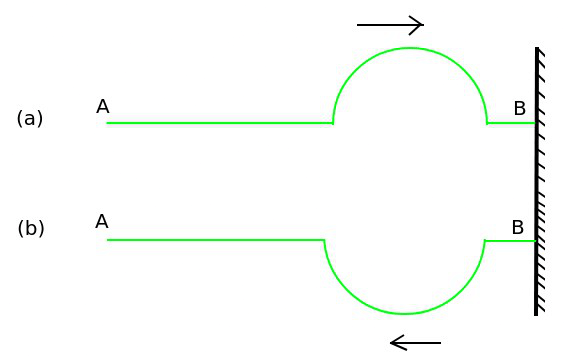 Reflection of Waves
Reflection of Waves
Standing Waves & Normal Modes
In certain situations, like a string fixed at both ends or an air column in a closed pipe, waves can bounce back and forth multiple times, creating what we call standing waves or stationary waves. Imagine a wave traveling in one direction along a string, reflecting at one end, then bouncing back from the other end. This process repeats until a steady wave pattern forms on the string.
- Mathematically, when we consider a wave moving in the positive x-direction and its reflection with the same amplitude and wavelength in the negative x-direction, we get a resultant wave described by the equation y(x, t) = 2a sin(kx)cos(ωt).
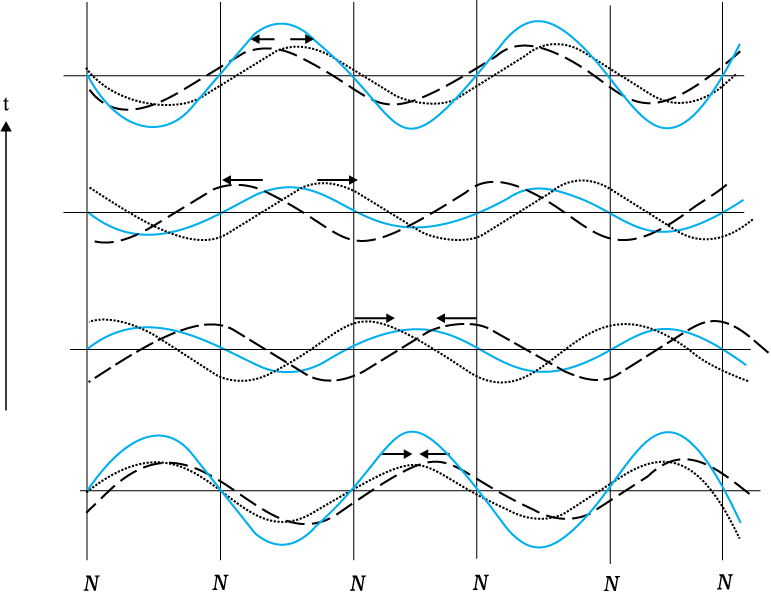 Stationary Waves Arising from Superposition
Stationary Waves Arising from Superposition
- This wave pattern is different from a regular traveling wave because the terms kx and ωt appear separately, not combined. The amplitude varies from point to point along the string, but each element of the string oscillates with the same frequency. This type of wave doesn't move left or right; it stands still, hence the name "standing" or "stationary" waves.
- In standing waves, the amplitude is fixed at a specific location, but it varies at different points.
- Nodes are points where there is no motion, and antinodes are points with the largest amplitude.
- The system of standing waves is limited by boundary conditions, meaning it has specific wavelengths or frequencies it can oscillate with. These are called natural frequencies or normal modes of oscillation.
- For example, a stretched string fixed at both ends follows these constraints in its vibration patterns.
Nodes
The positions of nodes (where the amplitude is zero) in a standing wave are given by the condition sin(kx) = 0 , implying kx = nπ where ( n = 0, 1, 2, 3, .....).
Since k = 2π/λ, we get x = nλ/2 , where n = 0, 1, 2, 3, .....
The distance between any two consecutive nodes is λ/2.
Antinodes
Similarly, the positions of antinodes (where the amplitude is largest) are given by the condition
|sin(kx)|= 1 , implying kx = (n + 1/2)π where ( n = 0, 1, 2, 3, .....).
With k = 2π/λ, we get x = (n+1/2)λ/2, where ( n = 0, 1, 2, 3, .....).
The distance between any two consecutive antinodes is λ/2.
Stretched String fixed at Both Ends
For a stretched string fixed at both ends, Taking one end to be at x = 0, the boundary conditions are that x = 0 and x = L are positions of nodes. The x = 0 condition is already satisfied.
- The x = L node condition requires that the length L is related to λ by L = nλ/2, where ( n = 0, 1, 2, 3, .....).
- This constraint results in possible wavelengths for stationary waves given by λ = 2L/n, where ( n = 0, 1, 2, 3, .....), with corresponding frequencies v = nv/2L for ( n = 0, 1, 2, 3, .....).
- These frequencies correspond to normal modes of oscillation, where the lowest frequency (n = 1) is called the fundamental mode or first harmonic, For the stretched string fixed at either end it is given by v = v/2L , . Higher frequencies (n = 2, 3, ...) are termed the second harmonic, third harmonic, and so on.
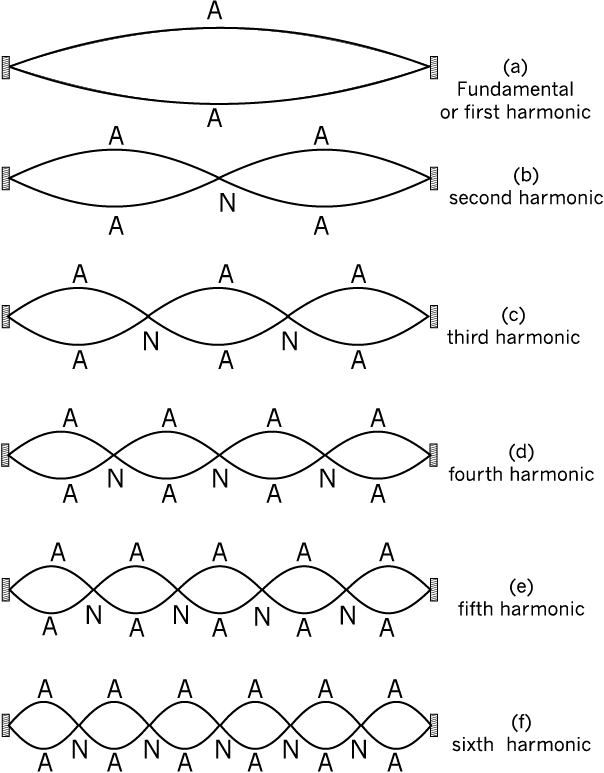 The First six harmonics of a Stretched String Fixed at Both Ends
The First six harmonics of a Stretched String Fixed at Both Ends
Musical instruments, like sitars or violins, use these principles, producing a superposition of different modes when plucked or bowed. The illustration in Figure 15.13 depicts the first six harmonics of a stretched string fixed at both ends.
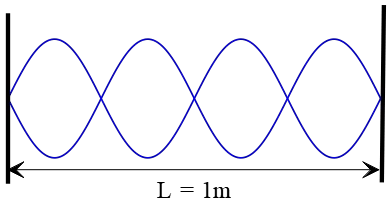
Example 1: The standing wave pattern is observed in a stretched string fixed at both ends, as shown in the figure below. If the speed of the waves in the string is 2m/s, at what frequency is this standing wave vibrating?
Answer: In the standing wave pattern shown, there are four loops or antinodes. These loops fit into the length of the string L. On the other side, recall that each loop has a length of ℓ=. So, the whole length of this string can accommodate four of these loops i.e. L=4ℓ or L=4(λ/2).
The wave speed and wavelength are also related together by λ=v/f. Substituting this into the length formula above, we get
Rearranging this relation and putting the numerical values, the required frequency is obtained as
Example 2: Two successive overtones of a vibrating guitar string are 280Hz and 350Hz. Find the frequency of the first harmonic?
Solution: Overtone is a name for other frequencies except the fundamental frequency. Suppose fn=280Hz, where n is not equal to 1, to be an unknown overtone. The next overtone after this is obtained by increasing n by one as n->n+1. So, the other next overtone is fn+1=350Hz.
Standing wave formula relates the other overtones to the first harmonic or fundamental frequency as fn=nf1. Divide those two overtones by each other and solve for n.
Solving the above equation, gets
This indicates that this particular standing wave formed on the string has four antinode or four loops.
Using the value of one of the given above overtone and substitute n=4 into it, we can find the fundamental frequency, f1.
Hence, the frequency of the first harmonic is 70Hz.
Air Column with One End Closed
Let's now consider the normal modes of oscillation for an air column with one end closed and the other open, such as a partially filled glass tube. In this system, the end in contact with water serves as a node (maximum pressure change, zero displacement), while the open end acts as an antinode (least pressure change, maximum displacement).
- Assuming the end in contact with water is at x = 0, the node condition is already satisfied. If the other end at x = L is an antinode, the equation L = (n+1/2)λ , where ( n = 0, 1, 2, 3, .....), gives the possible wavelengths: λ = 2L/2n + 1 for ( n = 0, 1, 2, 3, .....).
- The natural frequencies (normal modes) of the system are given by v = (n + 1/2)v/L for ( n = 0, 1, 2, 3, .....).
- The fundamental frequency corresponds to n = 0, given by v = v/4L. The higher frequencies are odd harmonics, i.e., odd multiples of the fundamental frequency: 3v/4L, 5v/4L and so on.
 Normal Modes of an Air Column Open at One End and Closed at Other
Normal Modes of an Air Column Open at One End and Closed at Other
For a pipe open at both ends, both ends act as antinodes, producing all harmonics.
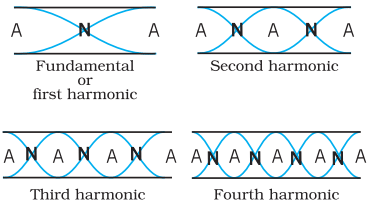 Standing Waves in an Open Pipe
Standing Waves in an Open Pipe
Both string and air column systems can also experience forced oscillations. When an external frequency is close to one of the natural frequencies, the system exhibits resonance.
In the case of a circular membrane, like a tabla, the normal modes are determined by the condition that no point on the membrane's circumference vibrates. Estimating the frequencies of these modes is more complex, involving wave propagation in two dimensions, although the underlying physics remains the same.
|
260 videos|253 docs|230 tests
|
FAQs on Reflection of Waves & Standing Waves - Physics for JAMB
| 1. What is the reflection of waves? |  |
| 2. What are standing waves and normal modes? |  |
| 3. How is a stretched string fixed at both ends related to standing waves? |  |
| 4. How does an air column with one end closed create standing waves? |  |
| 5. How do reflection of waves and standing waves play a role in music and acoustics? |  |