NCERT Solutions Class 11 Maths Chapter 2 - Relations and Functions
Exercise - 2.1
Q1: If 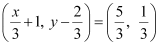 , find the values of x and y.
, find the values of x and y.
Ans: It is given that 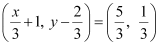 .
.
Since the ordered pairs are equal, the corresponding elements will also be equal.
Therefore, 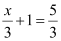 and
and 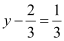 .
.
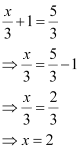
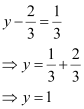
∴ x = 2 and y = 1
Q2: If the set A has 3 elements and the set B = {3, 4, 5}, then find the number of elements in (A × B)?
Ans: It is given that set A has 3 elements and the elements of set B are 3, 4, and 5.
⇒ Number of elements in set B = 3
Number of elements in (A × B)
= (Number of elements in A) × (Number of elements in B)
= 3 × 3 = 9
Thus, the number of elements in (A × B) is 9.
Q3: If G = {7, 8} and H = {5, 4, 2}, find G × H and H × G.
Ans: G = {7, 8} and H = {5, 4, 2}
We know that the Cartesian product P × Q of two non-empty sets P and Q is defined as
P × Q = {(p, q): p∈ P, q ∈ Q}
∴ G × H = {(7, 5), (7, 4), (7, 2), (8, 5), (8, 4), (8, 2)}
H × G = {(5, 7), (5, 8), (4, 7), (4, 8), (2, 7), (2, 8)}
Q4: State whether each of the following statement are true or false. If the statement is false, rewrite the given statement correctly.
(i) If P = {m, n} and Q = {n, m}, then P × Q = {(m, n), (n, m)}.
(ii) If A and B are non-empty sets, then A × B is a non-empty set of ordered pairs (x, y) such that x ∈ A and y ∈ B.
(iii) If A = {1, 2}, B = {3, 4}, then A × (B ∩ Φ) = Φ.
Ans: (i) False
If P = {m, n} and Q = {n, m}, then
P × Q = {(m, m), (m, n), (n, m), (n, n)}
(ii) True
(iii) True
Q5: If A = {–1, 1}, find A × A × A.
Ans: It is known that for any non-empty set A, A × A × A is defined as
A × A × A = {(a, b, c): a, b, c ∈ A}
It is given that A = {–1, 1}
∴ A × A × A = {(–1, –1, –1), (–1, –1, 1), (–1, 1, –1), (–1, 1, 1),
(1, –1, –1), (1, –1, 1), (1, 1, –1), (1, 1, 1)}
Q6: If A × B = {(a, x), (a, y), (b, x), (b, y)}. Find A and B.
Ans: It is given that A × B = {(a, x), (a, y), (b, x), (b, y)}
We know that the Cartesian product of two non-empty sets P and Q is defined as P × Q = {(p, q): p ∈ P, q ∈ Q}
∴ A is the set of all first elements and B is the set of all second elements.
Thus, A = {a, b} and B = {x, y}
Q7: Let A = {1, 2}, B = {1, 2, 3, 4}, C = {5, 6} and D = {5, 6, 7, 8}. Verify that
(i) A × (B ∩ C) = (A × B) ∩ (A × C)
(ii) A × C is a subset of B × D
Ans: (i) To verify: A × (B ∩ C) = (A × B) ∩ (A × C)
We have B ∩ C = {1, 2, 3, 4} ∩ {5, 6} = Φ
∴L.H.S. = A × (B ∩ C) = A × Φ = Φ
A × B = {(1, 1), (1, 2), (1, 3), (1, 4), (2, 1), (2, 2), (2, 3), (2, 4)}
A × C = {(1, 5), (1, 6), (2, 5), (2, 6)}
∴ R.H.S. = (A × B) ∩ (A × C) = Φ
∴L.H.S. = R.H.S
Hence, A × (B ∩ C) = (A × B) ∩ (A × C)
(ii) To verify: A × C is a subset of B × D
A × C = {(1, 5), (1, 6), (2, 5), (2, 6)}
B × D = {(1, 5), (1, 6), (1, 7), (1, 8), (2, 5), (2, 6), (2, 7), (2, 8), (3, 5), (3, 6), (3, 7), (3, 8), (4, 5), (4, 6), (4, 7), (4, 8)}
We can observe that all the elements of set A × C are the elements of set B × D.
Therefore, A × C is a subset of B × D.
Q8: Let A = {1, 2} and B = {3, 4}. Write A × B. How many subsets will A × B have? List them.
Ans: A = {1, 2} and B = {3, 4}
∴ A × B = {(1, 3), (1, 4), (2, 3), (2, 4)}
⇒ n(A × B) = 4
We know that if C is a set with n(C) = m, then n[P(C)] = 2m.
Therefore, the set A × B has 24 = 16 subsets. These are
Φ, {(1, 3)}, {(1, 4)}, {(2, 3)}, {(2, 4)}, {(1, 3), (1, 4)}, {(1, 3), (2, 3)},
{(1, 3), (2, 4)}, {(1, 4), (2, 3)}, {(1, 4), (2, 4)}, {(2, 3), (2, 4)},
{(1, 3), (1, 4), (2, 3)}, {(1, 3), (1, 4), (2, 4)}, {(1, 3), (2, 3), (2, 4)},
{(1, 4), (2, 3), (2, 4)}, {(1, 3), (1, 4), (2, 3), (2, 4)}
Q9: Let A and B be two sets such that n(A) = 3 and n (B) = 2. If (x, 1), (y, 2), (z, 1) are in A × B, find A and B, where x, y and z are distinct elements.
Ans: It is given that n(A) = 3 and n(B) = 2; and (x, 1), (y, 2), (z, 1) are in A × B.
We know that A = Set of first elements of the ordered pair elements of A × B
B = Set of second elements of the ordered pair elements of A × B.
∴ x, y, and z are the elements of A; and 1 and 2 are the elements of B.
Since n(A) = 3 and n(B) = 2, it is clear that A = {x, y, z} and B = {1, 2}.
Q10: The Cartesian product A × A has 9 elements among which are found (–1, 0) and (0, 1). Find the set A and the remaining elements of A × A.
Ans: We know that if n(A) = p and n(B) = q, then n(A × B) = pq.
∴ n(A × A) = n(A) × n(A)
It is given that n(A × A) = 9
∴ n(A) × n(A) = 9
⇒ n(A) = 3
The ordered pairs (–1, 0) and (0, 1) are two of the nine elements of A × A.
We know that A × A = {(a, a): a ∈ A}. Therefore, –1, 0, and 1 are elements of A.
Since n(A) = 3, it is clear that A = {–1, 0, 1}.
The remaining elements of set A × A are (–1, –1), (–1, 1), (0, –1), (0, 0),
(1, –1), (1, 0), and (1, 1)
Exercise - 2.2
Q1: Let A = {1, 2, 3, … , 14}. Define a relation R from A to A by R = {(x, y): 3x – y = 0, where x, y ∈ A}. Write down its domain, codomain and range.
Ans: The relation R from A to A is given as
R = {(x, y): 3x – y = 0, where x, y ∈ A}
i.e., R = {(x, y): 3x = y, where x, y ∈ A}
∴ R = {(1, 3), (2, 6), (3, 9), (4, 12)}
The domain of R is the set of all first elements of the ordered pairs in the relation.
∴ Domain of R = {1, 2, 3, 4}
The whole set A is the codomain of the relation R.
∴ Codomain of R = A = {1, 2, 3, …, 14}
The range of R is the set of all second elements of the ordered pairs in the relation.
∴ Range of R = {3, 6, 9, 12}
Q2: Define a relation R on the set N of natural numbers by R = {(x, y): y = x + 5, x is a natural number less than 4; x, y ∈ N}. Depict this relationship using roster form. Write down the domain and the range.
Ans: R = {(x, y): y = x + 5, x is a natural number less than 4, x, y ∈ N}
The natural numbers less than 4 are 1, 2, and 3.
∴ R = {(1, 6), (2, 7), (3, 8)}
The domain of R is the set of all first elements of the ordered pairs in the relation.
∴ Domain of R = {1, 2, 3}
The range of R is the set of all second elements of the ordered pairs in the relation.
∴ Range of R = {6, 7, 8}
Q3: A = {1, 2, 3, 5} and B = {4, 6, 9}. Define a relation R from A to B by R = {(x, y): the difference between x and y is odd; x ∈ A, y ∈ B}. Write R in roster form.
Ans: A = {1, 2, 3, 5} and B = {4, 6, 9}
R = {(x, y): the difference between x and y is odd; x ∈ A, y ∈ B}
∴R = {(1, 4), (1, 6), (2, 9), (3, 4), (3, 6), (5, 4), (5, 6)}
Q4: The given figure shows a relationship between the sets P and Q. write this relation
(i) in set-builder form (ii) in roster form.
What is its domain and range?
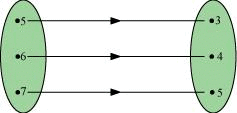 Ans: According to the given figure, P = {5, 6, 7}, Q = {3, 4, 5}
Ans: According to the given figure, P = {5, 6, 7}, Q = {3, 4, 5}
(i) R = {(x, y): y = x – 2; x ∈ P} or R = {(x, y): y = x – 2 for x = 5, 6, 7}
(ii) R = {(5, 3), (6, 4), (7, 5)}
Domain of R = {5, 6, 7}
Range of R = {3, 4, 5}
Ques 5: Let A = {1, 2, 3, 4, 6}. Let R be the relation on A defined by
{(a, b): a, b ∈ A, b is exactly divisible by a}.
(i) Write R in roster form
(ii) Find the domain of R
(iii) Find the range of R.
Ans: A = {1, 2, 3, 4, 6}, R = {(a, b): a, b ∈ A, b is exactly divisible by a}
(i) R = {(1, 1), (1, 2), (1, 3), (1, 4), (1, 6), (2, 2), (2, 4), (2, 6), (3, 3), (3, 6), (4, 4), (6, 6)}
(ii) Domain of R = {1, 2, 3, 4, 6}
(iii) Range of R = {1, 2, 3, 4, 6}
Q6: Determine the domain and range of the relation R defined by R = {(x, x + 5): x ∈ {0, 1, 2, 3, 4, 5}}.
Ans: R = {(x, x + 5): x ∈ {0, 1, 2, 3, 4, 5}}
∴ R = {(0, 5), (1, 6), (2, 7), (3, 8), (4, 9), (5, 10)}
∴ Domain of R = {0, 1, 2, 3, 4, 5}
Range of R = {5, 6, 7, 8, 9, 10}
Q7: Write the relation R = {(x, x3): x is a prime number less than 10} in roster form.
Ans: R = {(x, x3): x is a prime number less than 10}
The prime numbers less than 10 are 2, 3, 5, and 7.
∴R = {(2, 8), (3, 27), (5, 125), (7, 343)}
Q8: Let A = {x, y, z} and B = {1, 2}. Find the number of relations from A to B.
Ans: It is given that A = {x, y, z} and B = {1, 2}.
∴ A × B = {(x, 1), (x, 2), (y, 1), (y, 2), (z, 1), (z, 2)}
Since n(A × B) = 6, the number of subsets of A × B is 26.
Therefore, the number of relations from A to B is 26.
Q9: Let R be the relation on Z defined by R = {(a, b): a, b ∈ Z, a – b is an integer}. Find the domain and range of R.
Ans: R = {(a, b): a, b ∈ Z, a – b is an integer}
It is known that the difference between any two integers is always an integer.
∴ Domain of R = Z
Range of R = Z
Exercise - 2.3
Q1: Which of the following relations are functions? Give reasons. If it is a function, determine its domain and range.
(i) {(2, 1), (5, 1), (8, 1), (11, 1), (14, 1), (17, 1)}
(ii) {(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
(iii) {(1, 3), (1, 5), (2, 5)}
Ans: (i) {(2, 1), (5, 1), (8, 1), (11, 1), (14, 1), (17, 1)}
Since 2, 5, 8, 11, 14, and 17 are the elements of the domain of the given relation having their unique images, this relation is a function.
Here, domain = {2, 5, 8, 11, 14, 17} and range = {1}
(ii) {(2, 1), (4, 2), (6, 3), (8, 4), (10, 5), (12, 6), (14, 7)}
Since 2, 4, 6, 8, 10, 12, and 14 are the elements of the domain of the given relation having their unique images, this relation is a function.
Here, domain = {2, 4, 6, 8, 10, 12, 14} and range = {1, 2, 3, 4, 5, 6, 7}
(iii) {(1, 3), (1, 5), (2, 5)}
Since the same first element i.e., 1 corresponds to two different images i.e., 3 and 5, this relation is not a function.
Q2: Find the domain and range of the following real function:
(i) f(x) = –|x| (ii)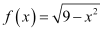
Ans: (i) f(x) = –|x|, x ∈ R
We know that |x| = 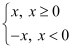
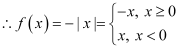
Since f(x) is defined for x ∈ R, the domain of f is R.
It can be observed that the range of f(x) = –|x| is all real numbers except positive real numbers.
∴The range of f is (–, 0].
(ii) 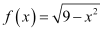
Since 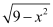 is defined for all real numbers that are greater than or equal to –3 and less than or equal to 3, the domain of f(x) is {x : –3 ≤ x ≤ 3} or [–3, 3].
is defined for all real numbers that are greater than or equal to –3 and less than or equal to 3, the domain of f(x) is {x : –3 ≤ x ≤ 3} or [–3, 3].
For any value of x such that –3 ≤ x ≤ 3, the value of f(x) will lie between 0 and 3.
∴The range of f(x) is {x: 0 ≤ x ≤ 3} or [0, 3].
Q3: A function f is defined by f(x) = 2x – 5. Write down the values of
(i) f(0), (ii) f(7), (iii) f(–3)
Ans: The given function is f(x) = 2x – 5.
Therefore,
(i) f(0) = 2 × 0 – 5 = 0 – 5 = –5
(ii) f(7) = 2 × 7 – 5 = 14 – 5 = 9
(iii) f(–3) = 2 × (–3) – 5 = – 6 – 5 = –11
Q4: The function ‘t’ which maps temperature in degree Celsius into temperature in degree Fahrenheit is defined by 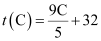 .
.
Find (i) t (0) (ii) t (28) (iii) t (–10) (iv) The value of C, when t(C) = 212
Ans: The given function is 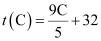 .
.
Therefore,
(i)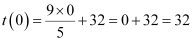
(ii)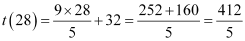
(iii)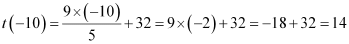
(iv) It is given that t(C) = 212
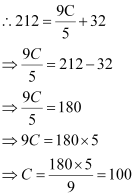
Thus, the value of t, when t(C) = 212, is 100.
Q5: Find the range of each of the following functions.
(i) f(x) = 2 – 3x, x ∈ R, x > 0.
(ii) f(x) = x2 + 2, x, is a real number.
(iii) f(x) = x, x is a real number
Ans: (i) Let x > 0
⇒ 3x > 0
⇒ 2 –3x < 2
⇒ f(x) < 2
∴ Range of f = (–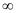 , 2)
, 2)
(ii) Given f (x) = x2 + 2, x is a real number
We know x2≥ 0 ⇒ x2 + 2 ≥ 0 + 2
⇒ x2 + 2 > 2 ∴ f (x) ≥ 2
∴ The range of f (x) is [2, ∞).
(iii) Given f (x) = x, x is a real number.
Let y =f (x) = x ⇒ y = x
∴ Range of f (x) = Domain of f (x)
∴ Range of f (x) is R.
|
73 videos|264 docs|91 tests
|
FAQs on NCERT Solutions Class 11 Maths Chapter 2 - Relations and Functions
| 1. What are relations and functions in mathematics? |  |
| 2. How do you determine if a relation is a function? |  |
| 3. What is the domain and range of a function? |  |
| 4. How can you represent a function algebraically? |  |
| 5. What is the difference between a one-to-one function and an onto function? |  |

















