Test: Moments Of Inertia For An Area About Inclined Axis - Mechanical Engineering MCQ
30 Questions MCQ Test - Test: Moments Of Inertia For An Area About Inclined Axis
If the non-Uniform loading is of the type of parabola then for calculating the moment of inertia for areas inclined at an axis?
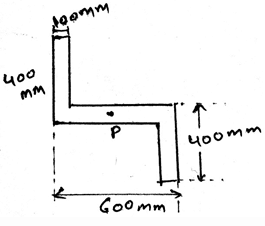
Determine the orientation of the principle axis for the cross section of area of member shown whose width is 100mm.
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The product of Inertia for an area is required so as to__________
Moment of Inertia about an inclined axis is the integration of the square of the distance of the centroid and the del area along the whole area of the structure and after this calculations we multiply the moment of areas.
The product of moment of inertia is the sum of _____________ and _________________. It can be used in the calculations of moments of inertia for an area about inclined axis.
There is perpendicular axis theorem for the area, and it is can be used to determine the moment of inertia of an area about inclined axis.
If any external force also is applied on the structure and we are determining the moment of inertia for areas about inclined axis then what should we consider?
Determine the magnitude of the principle moment of inertia for the cross section of area of member shown whose width is 100mm.
The calculation of moment of inertia about inclined axis due to the loadings involve a quantity called ____________
Whenever the distributed loading acts perpendicular to an area its intensity varies __________ for the determination of moment of inertia about the inclined axis.
The distance in the parallel axis theorem for the use in the determination of the moment of inertia about an inclined axis is multiplied by:
One of the use of the centre of mass or centroid is as in the determination of the moment of inertia about an inclined plane is that the net force acts at the ___________ of the loading body.
What is parallel axis theorem and to whom it is applied so that it can give the product of inertia of an area of an area inclined about an axis?
The body is sometimes acted by two or three force members and we need to find the moment of inertia about the inclined axis for the same. The difference between the two and the three force members is:
The parallel axis theorem can add any angle varied moment of inertias to give the perpendicular moment of inertia and it can be used in the determination of the moment of inertia about inclined axis.
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
In the calculations of moments of inertia for an area about inclined axis we use the product of moment of inertia. It is the the sum of _____________ and _________________
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
There is parallel axis theorem for the area, and it is can be used to determine the moment of inertia of an area about inclined axis.
Determine the moment of inertia of the area about y-axis.
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
Moment of Inertia about an inclined axis is the integration of the cube of the distance of the centroid and the del area along the whole area of the structure and after this calculations we multiply the moment of areas.
Determine the moment of inertia of the area about x-axis.
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
In the calculations of the moment of inertia of the area about an inclined axis, we have some transformation done. They are:
If any external moment along with the force is applied on the structure and we are determining the moment of inertia for areas about inclined axis then what should we consider?

















