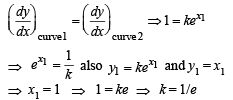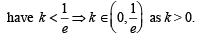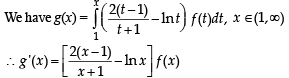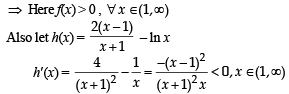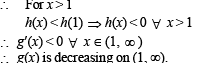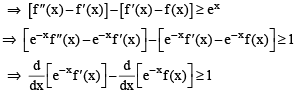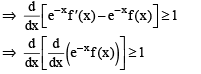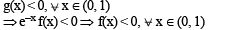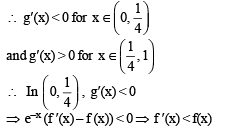All Courses
Get the App Signup / Login
Sign in Open App
JEE Exam  >  JEE Tests  >  Test: Comprehension Based Questions: Applications of Derivatives - JEE MCQ

Test: Comprehension Based Questions: Applications of Derivatives - JEE MCQ


Test Description

7 Questions MCQ Test - Test: Comprehension Based Questions: Applications of Derivatives

Test: Comprehension Based Questions: Applications of Derivatives for JEE 2024 is part of JEE preparation. The Test: Comprehension Based Questions: Applications of Derivatives questions and answers have been prepared according to the JEE exam syllabus.The Test: Comprehension Based Questions: Applications of Derivatives MCQs are made for JEE 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Comprehension Based Questions: Applications of Derivatives below.
Solutions of Test: Comprehension Based Questions: Applications of Derivatives questions in English are available as part of our course for JEE & Test: Comprehension Based Questions: Applications of Derivatives solutions in Hindi for JEE course. Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt Test: Comprehension Based Questions: Applications of Derivatives | 7 questions in 10 minutes | Mock test for JEE preparation | Free important questions MCQ to study for JEE Exam | Download free PDF with solutions
Test: Comprehension Based Questions: Applications of Derivatives - Question 1
Save

PASSAGE - 1

If a continuous function f defined on the real line R, assumes positive and negative values in R then the equation f(x) = 0 has a root in R. For example, if it is known that a continuous function f on R is positive at some point and its minimum value is negative then the equation f(x) = 0 has a root in R.

Consider f(x) = kex – x for all real x where k is a real constant.

Q. The line y = x meets y = kex for k < 0 at

Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 1

For k = 0, line y = x meets y = 0, i.e., x-axis only at one point.
For k < 0, y = kex meets y = x only once as shown in the graph.

Test: Comprehension Based Questions: Applications of Derivatives - Question 2
Save

PASSAGE - 1

If a continuous function f defined on the real line R, assumes positive and negative values in R then the equation f(x) = 0 has a root in R. For example, if it is known that a continuous function f on R is positive at some point and its minimum value is negative then the equation f(x) = 0 has a root in R.

Consider f(x) = kex – x for all real x where k is a real constant.

Q. The positive value of k for which kex – x = 0 has only one root is

Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 2

Let f (x) = kex – x

Now for f (x) = 0 to have only one root means the line y = x must be tangential to the curve y = kex.
Let it be so at (x1, y1) then

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Comprehension Based Questions: Applications of Derivatives - Question 3
Save

PASSAGE - 1

If a continuous function f defined on the real line R, assumes positive and negative values in R then the equation f(x) = 0 has a root in R. For example, if it is known that a continuous function f on R is positive at some point and its minimum value is negative then the equation f(x) = 0 has a root in R.

Consider f(x) = kex – x for all real x where k is a real constant.

Q. For k > 0, the set of all values of k for which kex – x = 0 has two distinct roots is

Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 3

∵ For y = x to be tangent to the curve y = kex, k = 1/e
∴ For y = kex to meet y = x at two points we should 

Test: Comprehension Based Questions: Applications of Derivatives - Question 4
Save

PASSAGE - 2

Let f (x) = (1 – x)2 sin2x + x2 for all x ∈ I R and let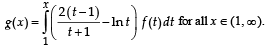 

Q.

Consider the statements:

P : Th er e exists some x ∈ R such th at f (x) + 2 x =  2(1 + x2)

Q : There exists some x ∈ R such that 2 f (x) + 1 = 2x (1 + x) Then
Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 4

For the statement P
f(x) + 2x = 2 (1 + x2)
⇒ (1 – x)2 sin2x + x2 + 2x = 2(1+ x2)
⇒ (1 – x)2 sin2x = x2 – 2x + 1 + 1
⇒ (1 – x)2 sin2x = (1 – x)2 + 1
⇒ (1 – x)2 cos2x = –1

Which is not possible for any real value of x.
∴ P is not true.
Also let H(x) = 2f(x) + 1 – 2x (1 + x)
H(0) = 2f(0) + 1 – 0 = 1
and H(1) = 2f(1) + 1 – 4 = – 3
⇒ H(x) has a solution in (0, 1)
∴ Q is true.

Test: Comprehension Based Questions: Applications of Derivatives - Question 5
Save

PASSAGE - 2

Let f (x) = (1 – x)2 sin2x + x2 for all x ∈ I R and let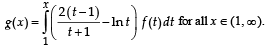 

Q. Which of the following is true?
Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 5



∴ h(x) is decreasing function.

Test: Comprehension Based Questions: Applications of Derivatives - Question 6
Save

PASSAGE - 3

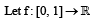(the set of all real numbers) be a function. Suppose the function f is twice differentiable, f(0) = f(1) = 0 and satisfies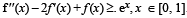 

Q. Which of the following is true for 0 < x < 1?
Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 6

We have f ''(x) – 2f '(x) +f(x) > ex


Let  g(x) = e–xf(x)
Then we have g ''(x) > 1> 0
So g is concave upward.
Also g(0) = g(1) = 0

Test: Comprehension Based Questions: Applications of Derivatives - Question 7
Save

PASSAGE - 3

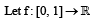(the set of all real numbers) be a function. Suppose the function f is twice differentiable, f(0) = f(1) = 0 and satisfies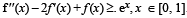 

Q. If the function e–x f(x) assumes its minimum in the interval   which of the following is true? 

Detailed Solution for Test: Comprehension Based Questions: Applications of Derivatives - Question 7

g(x) = e–xf(x)
⇒ g'(x) = e–xf '(x) – e–xf(x) = e–x (f '(x) – f(x))
As = 1/4 is point of local minima in [0, 1]

Information about Test: Comprehension Based Questions: Applications of Derivatives Page
In this test you can find the Exam questions for Test: Comprehension Based Questions: Applications of Derivatives solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Comprehension Based Questions: Applications of Derivatives, EduRev gives you an ample number of Online tests for practice

Top Courses for JEE

Download as PDF

Top Courses for JEE

Important Questions for Comprehension Based Questions: Applications of Derivatives

Find all the important questions for Comprehension Based Questions: Applications of Derivatives at EduRev.Get fully prepared for Comprehension Based Questions: Applications of Derivatives with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Comprehension Based Questions: Applications of Derivatives syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Comprehension Based Questions: Applications of Derivatives resources.

Comprehension Based Questions: Applications of Derivatives MCQs with Answers

Prepare for the Comprehension Based Questions: Applications of Derivatives within the JEE exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Comprehension Based Questions: Applications of Derivatives

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Comprehension Based Questions: Applications of Derivatives with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup