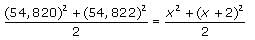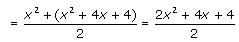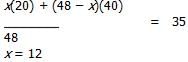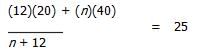Test: Mean - GMAT MCQ
10 Questions MCQ Test - Test: Mean
During 2005, a company produced an average of 2,000 products per month. How many products will thecompany need to produce from 2006 through 2008 in order to increase its monthly average for the periodfrom 2005 through 2008 by 200% over its 2005 average?
After his first semester in college, Thomas is applying for a scholarship that has a minimum Grade Point Average (GPA) requirement of 3.5. The point values of pertinent college grades are given in the table below. If Thomas took 5 courses, each with an equal weight for GPA calculations, and received two grades of A-, one grade of B+, and one grade of B, what is the lowest grade that Thomas could receive for his fifth class to qualify for the scholarship?


| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A certain portfolio consisted of 5 stocks, priced at $20, $35, $40, $45, and $70, respectively. On a givenday, the price of one stock increased by 15%, while the price of another stock decreased by 35% and theprices of the remaining three remained constant. If the average price of a stock in the portfolio rose byapproximately 2%, which of the following could be the prices of the shares that remained constant?
If John makes a contribution to a charity fund at school, the average contribution size will increase by50%, reaching $75 per person. If there were 5 other contributions made before John’s, what is the size ofhis donation?
What is the minimum percentage increase in the mean of set X {-4, -1, 0, 6, 9} if its two smallest elementsare replaced with two different primes?
Which of the following series of numbers, if added to the set {1, 6, 11, 16, 21}, will not change the set’smean?
I. 1.5, 7.11 and 16.89
II. 5.36, 10.7 and 13.24
III. -21.52, 23.3, 31.22
If numbers N and K are added to set X {2, 8, 10, 12}, its mean will increase by 25%. What is the value of N2 + 2NK + K2 ?
Set S consists of integers 7, 8, 10, 12, and 13. If integer n is included in the set, the average (arithmetic
mean) of set S will increase by 20%. What is the value of integer n?
A convenience store currently stocks 48 bottles of mineral water. The bottles have two sizes of either 20 or 40 ounces each. The average volume per bottle the store currently has in stock is 35 ounces. How many 40 ounce bottles must be sold for the average volume per bottle to be reduced to 25 ounces if no 20 ounce bottles are sold?