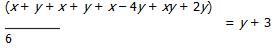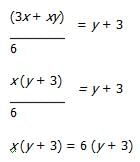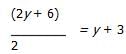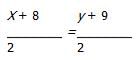Test: Median - GMAT MCQ
10 Questions MCQ Test - Test: Median
Which of the following could be the median of a set consisting of 6 different primes?
The median annual household income in a certain community of 21 households is $50,000. If themean income of a household increases by 10% per year over the next 2 years, what will the medianincome in the community be in 2 years?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
T is a set of y integers, where 0 < y < 7. If the average of Set T is the positive integer x, which of thefollowing could NOT be the median of Set T?
a, b, and c are integers and a < b < c. S is the set of all integers from a to b, inclusive. Q is the setof all integers from b to c, inclusive. The median of set S is (3/4) b. The median of set Q is (7/8) c.If R is the set of all integers from a to c, inclusive, what fraction of c is the median of set R?
Jim Broke’s only source of income comes from his job as a question writer. In this capacity, Jim earnsa flat salary of $200 per week plus a fee of $9 for every question that he writes. Every year, Jim takesexactly two weeks of unpaid vacation to visit his uncle, a monk in Tibet, and get inspired for the nextyear. If a regular year consists of 52 weeks and the number of questions that Jim wrote in each ofthe past 5 years was an odd number greater than 20, which of the following could be Jim’s medianannual income over the past 5 years?
During a behavioral experiment in a psychology class, each student is asked to compute his or herlucky number by raising 7 to the power of the student's favorite day of the week (numbered 1through 7 for Monday through Sunday respectively), multiplying the result by 3, and adding this tothe doubled age of the student in years, rounded to the nearest year. If a class consists of 28students, what is the probability that the median lucky number in the class will be a non-integer?
For the set of terms [x, y, x + y, x – 4y, xy, 2y], if y > 6 and the mean of the set equals y + 3, then
the median must be
Set A: 3, x, 8, 10 Set B: 4, y, 9, 11. The terms of each set above are given in ascending order. If the median of Set A is equal to the median of Set B, what is the value of y – x?
Set S includes elements {8, 2, 11, x, 3, y} and has a mean of 7 and a median of 5.5. If x < y, then
which of the following is the maximum possible value of x?
The temperatures in Celsius recorder at 6 in the morning in various parts of a certain country were
10,5,-2,-1,-5 and 15. What is the median of these temperatures?


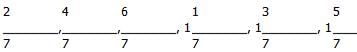
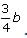
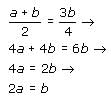
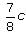 . We can set up and simplify the following equation:
. We can set up and simplify the following equation: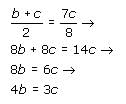
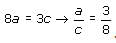
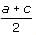 . We can use the ratio
. We can use the ratio 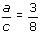 to substitute 3c/8 for a:
to substitute 3c/8 for a: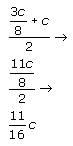
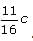 The correct answer is C
The correct answer is C