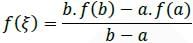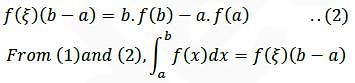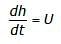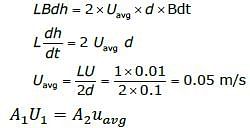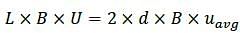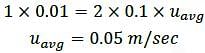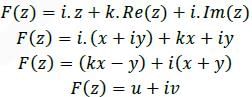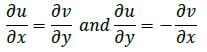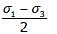Past Year Paper - Mechanical Engineering GATE : 2018 (Session - 1) - Mechanical Engineering MCQ
30 Questions MCQ Test - Past Year Paper - Mechanical Engineering GATE : 2018 (Session - 1)
A rectangle becomes a square when its length and breadth are reduced by 10 m and 5 m, respectively. During this process, the rectangle loses 650 m2 . What is the area of the original rectangle in square meters?
A number consists of two digits. The sum of the digits is 9. If 45 is subtracted from the number. Its digits are interchanged. What is the number?
Seven machines take 7 minutes to make 7 identical toys. At the same rate, how many minutes would it take for 100 machines to make 100 toys?
“Her _______ should not be confused with miserliness, she is ever willing to assist those in need.” The word that best fills the bank in the above sentence is :
Going by the __________ that many hands make light work, the school __________ involved all the students in the task.”
Given that a and b are integers and a + a2b3 is odd, which one of the following statementsis correct?
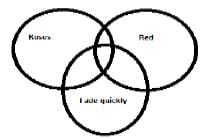
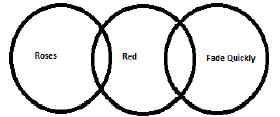
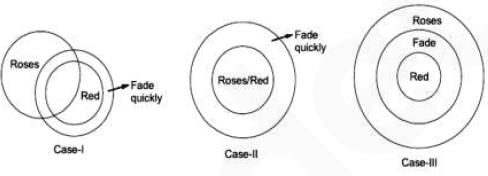
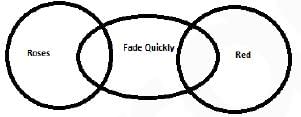
Consider the following three statements:
1. Some roses are red
2. All red flowers fade quickly.
3. Some roses fade quickly.
Q. Which of the following statements can be logically inferred from the above statements?
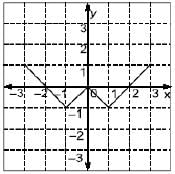
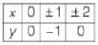
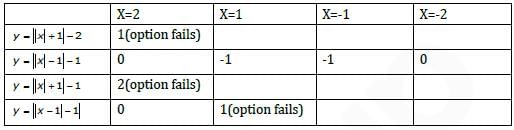
Which of the following functions describe the graph shows in the below figure.
For integers a, b and c, what would be the minimum and maximum values respectively of a + b + c if log| a|+ log| b |+ log| c | = 0
From the time the front of a train enters a platform, it takes 25 seconds for the back ofthe train to leave the platform, while travelling at a constant speed of 54 km/h. At thesame speed, it takes 14 seconds to pass a man running at 9 km/h in the same directionas the train. What is the length of the train and that of the platform in meter,respectively?
For a two-dimensional incompressible flow field given by where A > 0, whichone of the following statements is FALSE?
An ideal gas undergoes a process from state 1 (T1 = 300 K,p1 = 100 kPa) to state( ) 2(T2 = 600k,p2 = 500 kPa . The specific heats of the ideal gas are: cp=1 kJ/kg-k = and cv 0.7 kJ/kg-K. The change in specific entropy of the ideal gas from state 1 to state 2 (in kJ/kg-K) is ________ (correct to two decimal places)
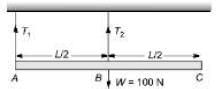
A bar of uniform cross section and weighing 100 N is held horizontally using two massless and inextensible strings S1 and S2 as shows in the figure.
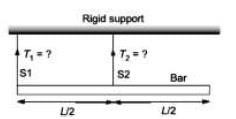
Tension in the springs are
For a Pelton wheel with a given water jet velocity, the maximum output power from the Pelton wheel is obtained when the ratio of the bucket speed to the water jet speed is_______ (correct to two decimal places).
A six- faced fair dice is rolled five times. The probability (in%)of obtaining “ONE” at leastfour times is
Using the Taylors tool life equation with exponent n = 0.5, if the cutting speed is reducedby 50% the ratio of new tool life to original tool life is
A grinding ratio of 200 implies that the
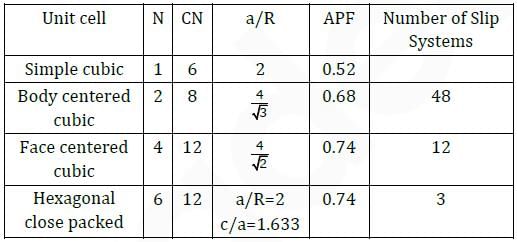
The number of atoms per unit cell and the number of slip systems, respectively, for aface-centered cubic (FCC) crystal are
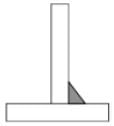
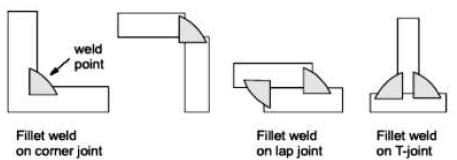
The type of weld represented by the shaded region in the figure is
The height (in mm) for a 125 mm sine bar to measure a taper of 27°32’ on a flat work piece is __________ (correct to three decimal places).
Interpolator in a CNC machine
If the wire diameter of a compressive helical spring is increased by 2% the change in spring stiffness (in %) is __________ (correct to two decimal places)
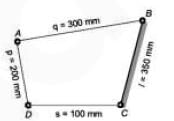
A four bar mechanism is made up of links of length 100,200,300 and 350 mm. if the 350 mm link is fixed, the number of links that can rotate fully is _________
Four red balls, four green balls and four blue balls are put in a box. Three balls are pulledout of the box at random one after another without replacement. The probability thatall the three balls are red is
The rank of the matrix 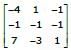 is
is
According to the Mean Value Theorem for a continuous function f (x) in the interval [a,b], there exists a value 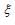 in this interval such that
in this interval such that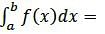
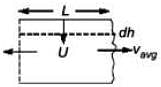
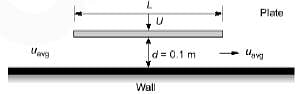
A flat plate of width L = 1 m is pushed down with a velocity U = 0.01 m/s towards a wall resulting in the drainage of the fluid between the plate and the wall as shown in the figure. Assume two-dimensional incompressible flow and that the plate remains parallel to the wall. The average velocity, Uavg of the fluid (in m/s) draining out at the instant shows in the figure is ___________ (correct to three decimal places).
F (z) is a function of the complex variable z = x + iy given by
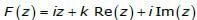
For what value of k will f(z) satisfy the Cauchy-Riemann equations?
If σ1 and σ3 are the algebraically largest and smallest principal stresses respectively the value of the maximum shear stress is.
The time series forecasting method that gives equal weightage to each of the M mostrecent observation is



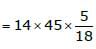
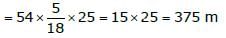
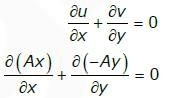
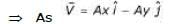
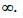 velocity vector field will not be defined along y axis.
velocity vector field will not be defined along y axis.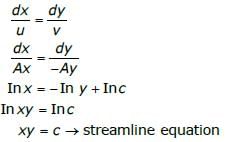
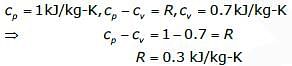
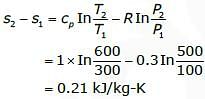
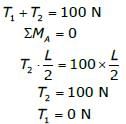
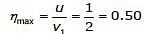
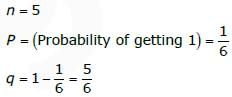
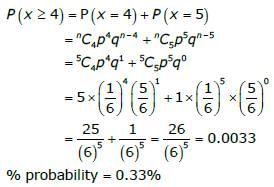
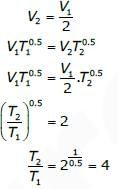

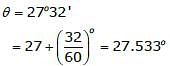
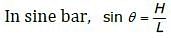
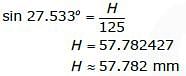
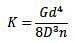
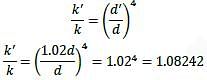

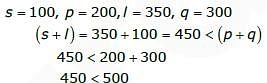
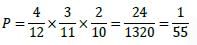
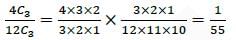
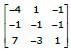

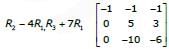
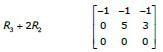
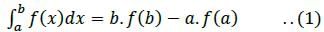
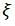 such that
such that