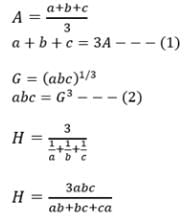JEE Advanced Level Test: Sequences and Series- 3 - JEE MCQ
25 Questions MCQ Test - JEE Advanced Level Test: Sequences and Series- 3
The sum of integers from 1 to 100 that are divisible by 2 or 5 is
Consider an A.P. with first term `a' and the common difference `d'. Let Sk denote the sum of its first K terms. If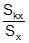 
is independent of x, then
is independent of x, then| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The next term of the sequence, 2, 6, 12, 20, …..is
If A, G & H are respectively te A.M., G.M. & H.M. of three positive numbers a, b, & c then the equation whose roots are a, b & c is given by
If ax = by = cz = dt and a, b, c, d are in G.P., then x, y, z, t are in
If xi > 0, i = 1, 2,....,50 ans x1 + x2 + ....+x50 = 50, then the minimum value of 
+ 
+ ......+
equal to
If a, a1, a2, a3, ....... , a2n, b are in A.P. and a, g1, g2, g3,.....g2n, b are in G.P. and h is the harmonic mean of a and b, then
+ 
+........ .....+ 
, is equal to
One side of an equilateral triangle is 24 cm. The mid-points of its sides are joined to form another triangle whose mid-points are in turn joined to form still another triangle. This process continues indefinitely. Then the sum of the perimeters of all the trianlges is
If a1, a2....an are in A.P. with common difference d > 0, then the sum of the series (sin d) [cosec a1 cosec a2 + cosec a2 cosec a3 +..... ......+ cosec an – 1 cosec an]
An infinite GP has first term x and sum 5, then x belongs to
If S1, S2, S3 are the sums of first n natural numbers, their squares, their cubes respectively, then
is equal to
If a1, a2, ...., an are in HP, then the expression a1a2 + a2a3 + ....+ an – 1 an is equal to
If x2 + 9y2 + 25z2 = xyz
, then x, y and z are in
The sum of all possible products of first n natural numbers taken two by two is
If G1 and G2 and two geometric means and A is the arithmetic means inserted between two positive numbers then the value of 
is
{an} and {bn} are two sequences given byan = 
and bn = 
for all n ∈ N. The value of a1 a2 a3...... an is equal to
If 12 + 22 + 32 + ..... + 20032 = (2003) (4007) (334) and (1) (2003) + (2) (2002) + (3) (2001) +.... ....+ (2003) (1) = (2003) (334) (x)., then x equals
Sum of first fifteen terms of series 5+10+20+… is
The common difference d of the A.P. in which T7 = 9 and T1T2T7 is least is
The H.M. between two numbers is , their A.M. is A and G.M. is G. If 2A + G2 = 26, then the numbers are
If 1, 2, 3.... are first terms; 1, 3, 5..... are common differences and S1, S2, S3.... are sums of n terms of given p AP's; then S1 + S2 + S3 + .... + Sp is equal to
For the A.P. given by a1, a2,...............an,..........., the equations satisfied are
If sum of the infinite G.P., p,1,1/p2 ,1/p3,...... is 9 /2, the value of p is
If positive numbers a, b, c are in A.P. and a2, b2, c2 are in H.P., then
If the arithmetic mean of two positive numbers a & b (a > b) is twice their geometric mean, then a : b is
Top Courses for JEE
Important Questions for JEE Advanced Level Test: Sequences and Series- 3
JEE Advanced Level Test: Sequences and Series- 3 MCQs with Answers
Online Tests for JEE Advanced Level Test: Sequences and Series- 3
|
© EduRev
|
Education Revolution
|
Follow Us
|
