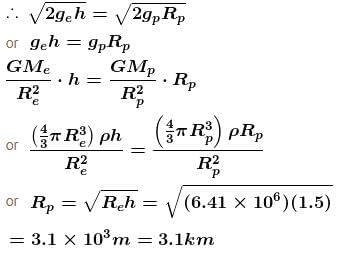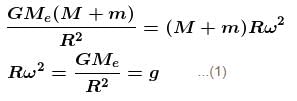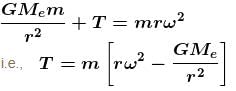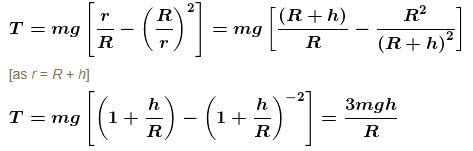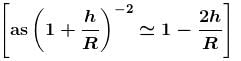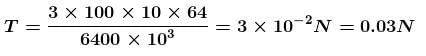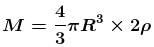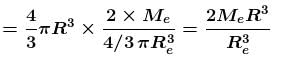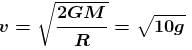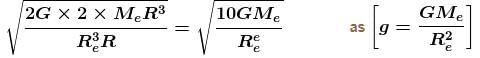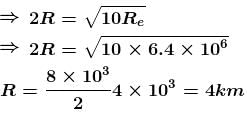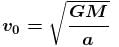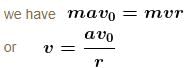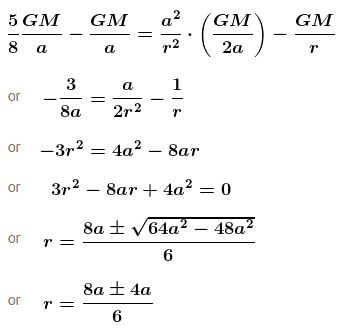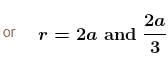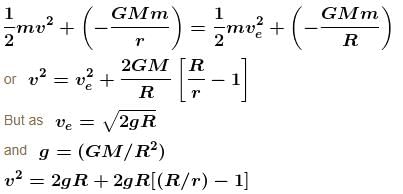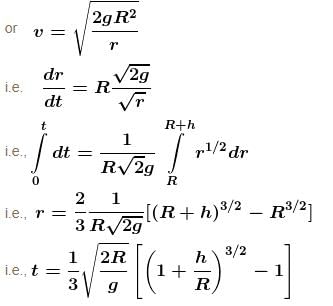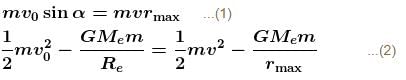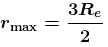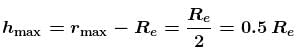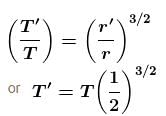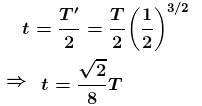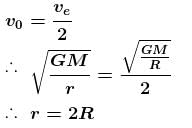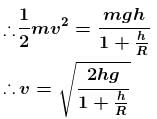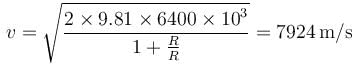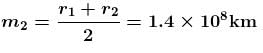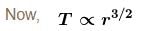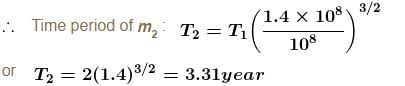Open App

IIT JAM Exam > IIT JAM Tests > Central Forces NAT Level – 2 - IIT JAM MCQ

Central Forces NAT Level – 2 - IIT JAM MCQ

Test Description

10 Questions MCQ Test - Central Forces NAT Level – 2

Central Forces NAT Level – 2 for IIT JAM 2024 is part of IIT JAM preparation. The Central Forces NAT Level – 2 questions and answers have been prepared according to the IIT JAM exam syllabus.The Central Forces NAT Level – 2 MCQs are made for IIT JAM 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Central Forces NAT Level – 2 below.

Solutions of Central Forces NAT Level – 2 questions in English are available as part of our course for IIT JAM & Central Forces NAT Level – 2 solutions in Hindi for IIT JAM course. Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free. Attempt Central Forces NAT Level – 2 | 10 questions in 45 minutes | Mock test for IIT JAM preparation | Free important questions MCQ to study for IIT JAM Exam | Download free PDF with solutions

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 1

A man can jump vertically to a height of 1.5m on the earth. Calculate the radius of a planet (in kms ) of the same mean density as that of the earth from whose gravitational field he could escape by jumping. Radius of earth is 6.41 × 10⁶m.

Detailed Solution for Central Forces NAT Level – 2 - Question 1

For the asked planet this u should be equal to the escape velocity from its surface.

The correct answer is: 3.1

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 2

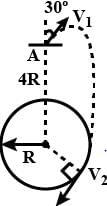
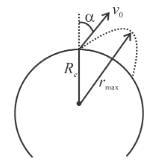
A particle is projected from point A that is at a distance 4R from the centre of the Earth will speed v₁ in a direction making joining the centre of the Earth and point A as shown.find the speed v₂if particle passes grazing the surface of the earth Constant interaction only between these two Express you answer in the form (500 × √2X)m/s and write the value of X?

Detailed Solution for Central Forces NAT Level – 2 - Question 2

let v₂ to be speed when it grazes earth

Conserving angular momentum we get
mv₁sin30^o4R = m.v₂R.....(i)
Conserving total energy we get
1/2mV₁² − GmM/4R

= 1/2 mV₂²− GmM/R

putting V₂= V₁sin30^o× 4
= 2V₁ we get
1/2mV₁²(1−4) = −3GmM/4R
or

V₁ =(√Gm/2R)

= (√6.4*10⁷)/2

= 500X√2

X = (8000)/(500*2)

X = 8

1 Crore+ students have signed up on EduRev. Have you? Download the App

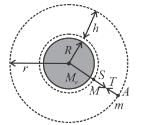
*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 3

A cord of length 64m is used to connect a 100kg astronaut. Estimate the value of the tension (in Newton) in the cord. Assume that the spaceship is orbiting near earth surface. Also assume that the spaceship and the astronaut fall on a straight line from the earth’s centre. The radius of the earth is 6400km.

Detailed Solution for Central Forces NAT Level – 2 - Question 3

As according to given problem the mass of satellite M is much greater than that of astronaut m so the centre of mass of the system will be close to satellite and as the satellite is orbiting close to the surface of earth, the equation of motion of the system (S + A) will be :

And the equation motion of the astronaut will be

So substituting the given data,

The correct answer is: 0.03

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 4

Ravi can throw a ball a speed on earth which can cross a river of width 10m. Ravi reaches on an imaginary planet whose mean density is twice of the earth. If maximum radius of planet so that if Ravi throws the ball at same speed it may escape from planet is xkm. then x is.

(Given radius of earth = 6.4 × 10⁶m.)

Detailed Solution for Central Forces NAT Level – 2 - Question 4

Speed of the ball which can cross 10 m wide river is

Let the radius of planet is R,
Then, mass of planet

Escape velocity of planet

The correct answer is: 4

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 5

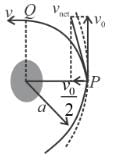
An earth satellite is revolving in a circular orbit of radius a with velocity v₀. There is a gun in the satellite and is aimed directly towards the earth. A bullet is fired from the gun with muzzle velocity Neglecting resistance offered by cosmic dust and recoil of gun, the maximum and minimum distance of bullet from the centre of earth during its subsequent motion is na and 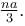Find the value of n.

Detailed Solution for Central Forces NAT Level – 2 - Question 5

Orbital speed of satellite is
From conservation of angular momentum at P and Q,

From conservation of mechanical energy at P and Q, we have

Substituting values of v and v₀ from Eqs. (i) and (ii), we get

Hence, the maximum and minimum distance are 2a and 2a/3 respectively.
The correct answer is: 2

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 6

A body is projected vertically upwards from the surface of earth with a velocity sufficient to carry it to infinity. The time taken by it to reach height h is given by 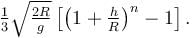Find the value of n.

Detailed Solution for Central Forces NAT Level – 2 - Question 6

If at a distance r from the centre of the earth the body has velocity v, by conservation of mechanical energy,

The correct answer is: 1.5

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 7

A projectile of mass m is fired from the surface of the earth at an angle α = 60° from the vertical. The initial speed v₀ is equal to 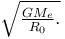The height projectile rises is given by nR_e. Find the value of n. Neglect air resistance and the earth’s rotation.

Detailed Solution for Central Forces NAT Level – 2 - Question 7

Let v be the speed of the projectile at highest point and r_max its distance from the centre of the earth. Applying conservation of angular momentum and mechanical energy,

Solving these two equation with the given data we get,

or the maximum height
The correct answer is: 0.5

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 8

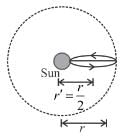
If a planet was suddenly stopped in its orbit supposed to be circular, it will fall onto the sun in a time 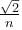times the period of the planet’s revolution. Find the value of n.

Detailed Solution for Central Forces NAT Level – 2 - Question 8

Consider and imaginary planet moving along a strongly extended flat ellipse, the extreme points of which are located on the planet’s orbit and at the centre of the sun. The semi-major axis of the orbit of such a planet would apparently be half the semi-major axis of the planet’s orbit. So the time period of the imaginary planet T' according to Kepler’s law will be given by

∴ Time taken by the planet to fall onto the sun is

The correct answer is: 8

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 9

An artificial satellite is moving in a circular orbit around the earth with a speed equal to half the magnitude of escape velocity from the surface of earth. (Radius of the earth = 6400km ). If the satellite is stopped suddenly in its orbit and allowed to fall freely on the earth, find the speed (in m/s) with which it hits the surface of earth.

Detailed Solution for Central Forces NAT Level – 2 - Question 9

or h - r - R = R or height = radius of earth.
Increase in kinetic energy = decrease in potential energy

Substituting the values we have,

The correct answer is: 7924

*Answer can only contain numeric values

Central Forces NAT Level – 2 - Question 10

Two planets of equal mass orbit a much more massive star (figure). Planet m₁moves in a circular orbit of radius 1 × 10⁸km with period 2year. Planet m₂ moves in an elliptical orbit with closed distance r₁ = 1 × 10⁸km and farthest distance r₂ = 1.8 × 10⁸km, as shown.

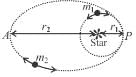
Using the fact that the mean radius of an elliptical orbit is the length of the semi-major axis, find the period of m₂'s orbit.

Detailed Solution for Central Forces NAT Level – 2 - Question 10

Mean radius of planet,

The correct answer is: 3.31

Information about Central Forces NAT Level – 2 Page

In this test you can find the Exam questions for Central Forces NAT Level – 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Central Forces NAT Level – 2, EduRev gives you an ample number of Online tests for practice

Important Questions for Central Forces NAT Level – 2

Find all the important questions for Central Forces NAT Level – 2 at EduRev.Get fully prepared for Central Forces NAT Level – 2 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Central Forces NAT Level – 2 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Central Forces NAT Level – 2 resources.

Central Forces NAT Level – 2 MCQs with Answers

Prepare for the Central Forces NAT Level – 2 within the IIT JAM exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Central Forces NAT Level – 2

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Central Forces NAT Level – 2 with ease. Join thousands of successful students who have benefited from our trusted online resources.

Education Revolution