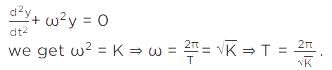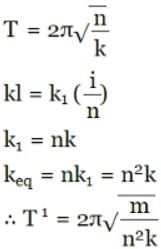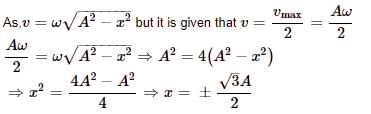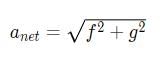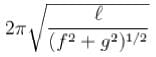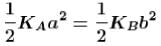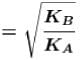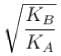Simple Harmonic Motion MCQ - IIT JAM MCQ
10 Questions MCQ Test - Simple Harmonic Motion MCQ
The equation of S.H.M of a particle is 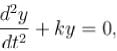
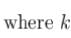 is a positive constant. The time period of motion is given by :
is a positive constant. The time period of motion is given by :
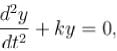
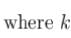 is a positive constant. The time period of motion is given by :
is a positive constant. The time period of motion is given by :If length of simple pendulum is increased by 6 % then percentage change in time period will be :
A particle is executing S.H.M. from mean position at 5 cm distance, acceleration is 20 cm/s2 then value of angular velocity will be :
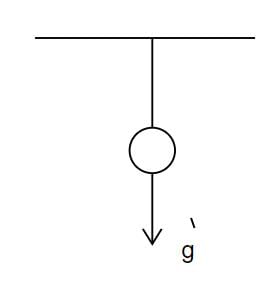
A man measures the time period of a simple pendulum inside a stationary lift and finds it to be 10 sec. If the lift accelerates upwards with an acceleration g/4, then find the time period of the pendulum.
The maximum velocity of harmonic oscillator is a, and its maximum acceleration is β Its time period will be :
One mass m is suspended from a spring. Time period of oscillation is T. Now if spring is divided into n piece and these are joined in parallel order then time period of oscillation if same mass is suspended.
Amplitude of harmonic oscillator is a when velocity of particle is half of maximum velocity, then position of particle will be :
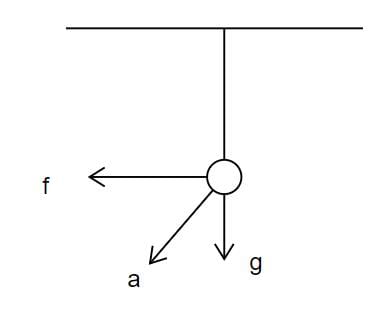
A simple pendulum suspended from the ceiling of a stationary trolley has a length 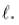 Its period of oscillation is
Its period of oscillation is  What will be its period of oscillation if the trolley moves forward with an acceleration f ?
What will be its period of oscillation if the trolley moves forward with an acceleration f ?
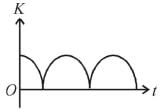
In S.H.M, the graph between kinetic energy 'K' and time 't' is:
Two object A and B of equal mass are suspended from two springs of spring constants KA and KB if the objects oscillate vertically in such a manner that their maximum kinetic energies are equal, then the ratio of their amplitude is :