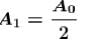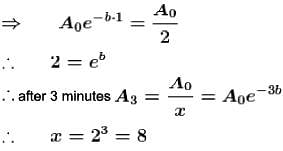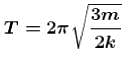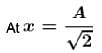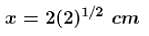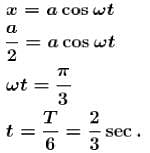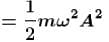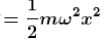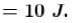Simple Harmonic Motion NAT Level - 1 - IIT JAM MCQ
10 Questions MCQ Test - Simple Harmonic Motion NAT Level - 1
A spring has a certain mass suspended from it and its period for vertical oscillation is T1. The spring is now cut into two equal halves and the same mass is suspended from one of the half. The period of vertical oscillation is now T2. The ratio of 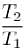 is :
is :
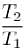 is :
is :For a simple harmonic vibrator of frequency n, the frequency of kinetic energy changing completely to potential energy is αn. Find the value of α.
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The total energy of the body executing S.H.M is E. Then the kinetic energy when the displacement is half of the amplitude is αE Find the value of α.
The amplitude of a damped harmonic oscillator become halved in 1 minute. After three minutes the amplitude will become 1/x of initial amplitude where x is :
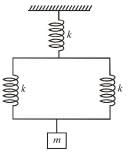
One body of mass m is suspended from three springs as shown in figure each spring has spring constant k. If mass m is displaced slightly then time period of oscillation is 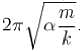 Find the value of α.
Find the value of α.
A particle is executing SHM with an amplitude 4 cm. The displacement (in cm) at which its energy it half kinetic and half potential is :
A particle executing S.H.M of amplitude 4 cm and T = 4 sec. The time taken (in sec) by it to move from positive extreme position to half the amplitude is :
The potential energy of a particle executing S.H.M. is 2.5 J when its displacement is half of amplitude. The total energy (in Joule) of the particle is :
If the time period of oscillation of mass M suspended from a spring is one second, then the time period (in sec) of 4M will be :
A linear harmonic oscillation of force constant 2 x 106 Nlm and amplitude 0.01 m has a total mechanical energy of 160 joules. Its maximum K.E. (in Joule) will be.


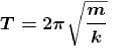
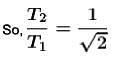
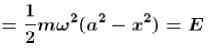
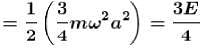
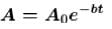
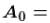 initial amplitude,
initial amplitude,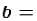 damped factor
damped factor