Olympiad Test: Square And Square Roots - Class 8 MCQ
20 Questions MCQ Test - Olympiad Test: Square And Square Roots
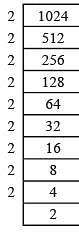
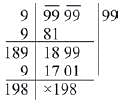
What is the greatest number of five digits which is a perfect square?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
What is the least number which must be subtracted from 6459 to make a perfect square?
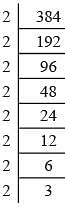
By what least number 384 be multiplied so that the product may be a perfect square?
What is the smallest number of four digits which is a perfect square?
What is the greatest number of four digits which is a perfect square?
Find the least number which must be added to 2292 to make it a perfect square.
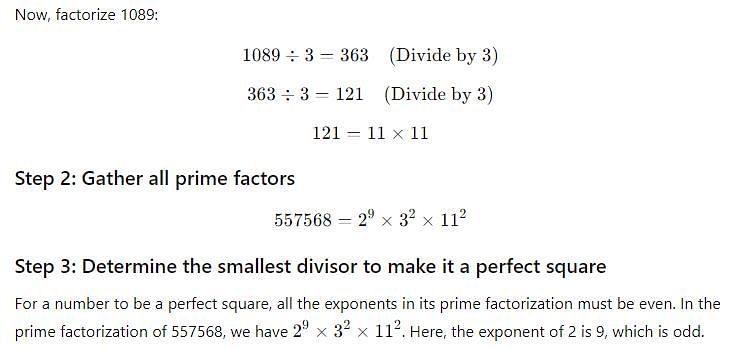
Find the smallest number by which 557568 must be divided so that it become a perfect square.
Find the smallest number by which 396 must be multiplied so that the product becomes a perfect square?
What is the least number which must be added to 6203 to obtain a perfect square ?
Find the smallest square number that is divisible by each of the number 8, 15 and 20.
What is the least number that must be added to 1300 so as to get a perfect square?
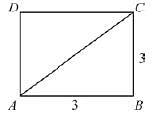
What is the length of the diagonal of a square whose perimeter is equal to the perimeter of an equilateral triangle of side 4 cm?
The product of two positive numbers is 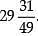 and one of them is three times the other. Find the larger number.
and one of them is three times the other. Find the larger number.
The area of a rectangular field whose length is three times its breadth is 348 m2. What is the perimeter of the field?
Which of the following is not a Pythagorean triplet?
The area of a square field is 60025 m2. A man cycles along its boundary at 18 km/hour. In how much time will he return to the starting point?
What is the length of each side of a square whose area is equal to the area of a rectangle of length 13.6 m and breadth 3.4 meters?
The perimeter of a square field is 76m. What is its area?
Which of the following is the square of an even number?


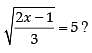

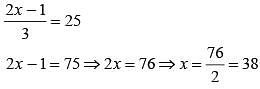
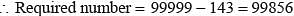
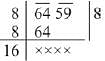
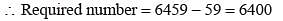
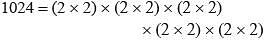
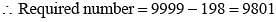
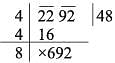
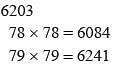
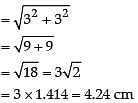
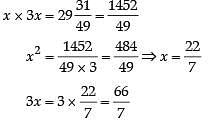
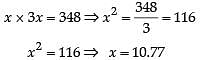
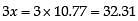
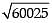 = 245 m
= 245 m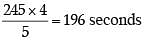
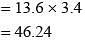
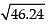 = 6.8 m
= 6.8 m














