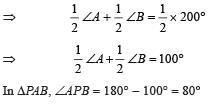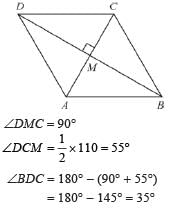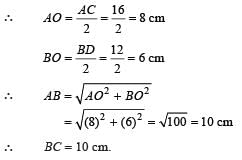All Courses
Get the App Signup / Login
Sign in Open App
Class 8 Exam  >  Class 8 Tests  >  Olympiad Test: Quadrilaterals - 1 - Class 8 MCQ

Olympiad Test: Quadrilaterals - 1 - Class 8 MCQ


Test Description

20 Questions MCQ Test - Olympiad Test: Quadrilaterals - 1

Olympiad Test: Quadrilaterals - 1 for Class 8 2024 is part of Class 8 preparation. The Olympiad Test: Quadrilaterals - 1 questions and answers have been prepared according to the Class 8 exam syllabus.The Olympiad Test: Quadrilaterals - 1 MCQs are made for Class 8 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Olympiad Test: Quadrilaterals - 1 below.
Solutions of Olympiad Test: Quadrilaterals - 1 questions in English are available as part of our course for Class 8 & Olympiad Test: Quadrilaterals - 1 solutions in Hindi for Class 8 course. Download more important topics, notes, lectures and mock test series for Class 8 Exam by signing up for free. Attempt Olympiad Test: Quadrilaterals - 1 | 20 questions in 40 minutes | Mock test for Class 8 preparation | Free important questions MCQ to study for Class 8 Exam | Download free PDF with solutions
Olympiad Test: Quadrilaterals - 1 - Question 1
Save

The four angles of a quadrilateral are in the ratio 2 : 3 : 5 : 8. Then what is the difference between largest and smallest angle of the quadrilateral?

Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 1

Let the angles are 2x, 3x, 5x, 8x.
∴ 2x + 3x + 5x + 8x = 360°
⇒ 18x = 360° ⇒ x = 20°
2x = 2 × 20° = 40°; 3x = 3 × 20° = 60°
5x = 5 × 20° = 100°; 8x = 8 × 20° = 160°
Required difference = 160° - 40° = 120°.

Olympiad Test: Quadrilaterals - 1 - Question 2
Save

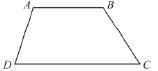
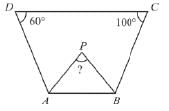
In the given figure the bisector of ∠A and ∠B meet in a point P. If ∠C = 100°, ∠D = 60°, what is the the measure of ∠APB?

Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 2

In figure ∠A + ∠B + ∠C + ∠D = 360°
⇒  ∠A + ∠B + 100° + 60° = 360°
⇒  ∠A + ∠B = 200°

1 Crore+ students have signed up on EduRev. Have you? Download the App
Olympiad Test: Quadrilaterals - 1 - Question 3
Save

Three angles of a quadrilateral are equal and the measure of fourth angle is 120°. What  is the measure of each of the equal angle?

Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 3

Let the angle be x.
∴ x + x + x + 120° = 360°
⇒ 3x = 240° ⇒ x = 80°

Olympiad Test: Quadrilaterals - 1 - Question 4
Save

The length of diagonals of a rhombus are 16 cm and 12 cm respectively. What is the length of each of its sides?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 4

Here ABCD is a rhombus and in ∆AOD

Olympiad Test: Quadrilaterals - 1 - Question 5
Save

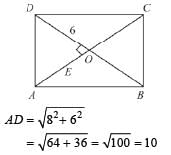
The sum of two opposite angles of a parallelogram is 130°. What is the difference between largest and smallest angle of parallelogram?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 5

Here ABCD is a parallelogram and ∠A + ∠C = 130°

∠B = ∠D = 115°
Difference = 115° - 65° = 50°

Olympiad Test: Quadrilaterals - 1 - Question 6
Save

The bisectors of any two adjacent angles of a parallelogram intersect at
Olympiad Test: Quadrilaterals - 1 - Question 7
Save

If one angle of a parallelogram is 24° less than twice the smallest angle then what is the value of largest angle of the parallelogram?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 7

Let ∠A be the smallest angle.

Olympiad Test: Quadrilaterals - 1 - Question 8
Save

The ratio of two sides of a parallelogram is 4 : 3. If its perimeter is 56 cm, what is the difference between largest and smallest side?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 8

The opposite sides of parallelogram are equal.
∴ 4x + 3x + 4x + 3x = 56
⇒ 14x = 56 ⇒ x = 4
∴ 4x = 4 × 4 = 16
and 3x = 4 × 3 = 12
∴ Difference = 16 – 12 = 4 cm

Olympiad Test: Quadrilaterals - 1 - Question 9
Save

In the given Fig. ABCD is a rhombus. If ∠DAB = 110°, what is ∠BDC ? 
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 9

In rhombus ABCD,
∠DAB = 110° = ∠BCD

Olympiad Test: Quadrilaterals - 1 - Question 10
Save

Two adjacent sides of a parallelogram are 5 cm and 7 cm long what is the perimeter of parallelogram?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 10

Perimeter of parallelogram
= 5 + 7 + 5 +7 = 24 cm

Olympiad Test: Quadrilaterals - 1 - Question 11
Save

Of any two adjacent sides of a parallelogram one is longer than the other by 3 cm. If the perimeter is 36 cm, then what is the length of smaller side of parallelogram?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 11

Let smaller side be x.
Longer side = x +3
∴ x + x + 3 + x + x + 3 = 36
⇒ 4x + 6 = 36 ⇒ 4x = 30

Olympiad Test: Quadrilaterals - 1 - Question 12
Save

ABCD is a rhombus, AC = 16 cm and BD = 12 cm, then what is the measure of BC?
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 12

∵ ABCD is a rhombus.
∴ AB = BC = CD = DA
∵ Diagonals of a rhombus bisect each other. 

Olympiad Test: Quadrilaterals - 1 - Question 13
Save

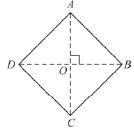
In ∆BCE, BE = EC, and ABCD is a square, and ∠BEC = 60°, then the measure of ∠BEA will be : 
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 13

Given BE = EC
∴ ∠EBC = ∠ECB
In ∆BEC,
2∠EBC + ∠BEC = 180°
⇒ ∠EBC = ∠ECB = 60°
∴ ∆EBC is an equilateral triangle having
EB = BC = EC
AB = BC = CD = DA = EB = EC
[∵ABCD is a square].
∠ABE = 90° + 60° = 150°
In ∆ABE,
∠ABE + 2∠AEB = 180° [Q AEB =  BAE].
2∠AEB = 180° - ∠ABE = 180 - 150 = 30°
⇒ ∠AEB = 15°

Olympiad Test: Quadrilaterals - 1 - Question 14
Save

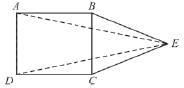
From the adjoining figure, find the measure of ∠EFD if AB || CD, EF || BC.
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 14

∠ABC + ∠BCF = 180°
[Interior angles or same side of transversal].
⇒ ∠BCF =180° - 120° = 60°
∠BCF = ∠EFD (corresponding angles)
∴ ∠EFD = 60°

Olympiad Test: Quadrilaterals - 1 - Question 15
Save

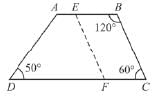
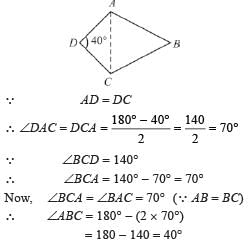
In the adjoining figure ABCD, AB = DC and AB = BC and, ∠ADC = 40° and ∠BCD = 140°, then, ∠ABC =
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 15

Joining A to C,

Olympiad Test: Quadrilaterals - 1 - Question 16
Save

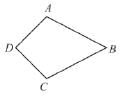
ABCD is a parallelogram having its sides, AB = 4x + 1 BC = 2y + 3, CD = 25, DA = y + 28, then, x + y =
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 16

In a parallelogram.
AB = CD, and, BC = DA
⇒  4x + 1 = 25 and 2y + 3 = y + 28
⇒ x = 6, and, y = 25
∴ x + y = 6 + 25 = 31

Olympiad Test: Quadrilaterals - 1 - Question 17
Save

PQRS is a parallelogram and ∠SPR = 50°, then find y.
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 17

PQRS is a parallelogram.
∴ ∠S = ∠Q = y = 70°

Olympiad Test: Quadrilaterals - 1 - Question 18
Save

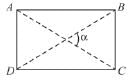
If α < 90°, then, ABCD, may be a : (Given : ABCD is a parallelogram) and ∠B = 90°
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 18

∵ ∠B = 90°, and, ABCD is a parallelogram
∴ ∠C = 90°, ∠A = 90° and ∠D = 90°
So, ABCD may be a rectangle or square, but, since, α < 90°.
∴ΑΒCD must be a rectangle.

Olympiad Test: Quadrilaterals - 1 - Question 19
Save

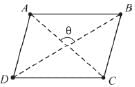
If θ = 90°, and, ∠A = ∠C = 110°, then, ABCD is :
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 19

∵ θ = 90° (Angle between the diagonals)
∴ ABCD may be a rhombus or square.
But, since, ∠A = ∠C = 110°
∴ ABCD must be a rhombus.

 In a rhombus or any parallelogram, the opposite angles are always equal 

Olympiad Test: Quadrilaterals - 1 - Question 20
Save

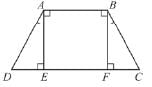
ABCD is an isosceles trapezium , i.e., AD = BC, then 
Detailed Solution for Olympiad Test: Quadrilaterals - 1 - Question 20

∵ ABCD is an isosceles trapezium.
∴ AD = BC

Draw AE ⊥ DC and BF ⊥ DC, then, in ∆AED and ∠BFC.
∠AED = ∠BFC =  90°,
AB = BC,
AE = BF (perpendicular distance between two parallel lines)
∴ ∆AEB ≅ ∆BFC (ets congruency)
∴ ∠A = ∠B

Information about Olympiad Test: Quadrilaterals - 1 Page
In this test you can find the Exam questions for Olympiad Test: Quadrilaterals - 1 solved & explained in the simplest way possible. Besides giving Questions and answers for Olympiad Test: Quadrilaterals - 1, EduRev gives you an ample number of Online tests for practice

Top Courses for Class 8

Download as PDF

Top Courses for Class 8

Important Questions for Olympiad Test: Quadrilaterals - 1

Find all the important questions for Olympiad Test: Quadrilaterals - 1 at EduRev.Get fully prepared for Olympiad Test: Quadrilaterals - 1 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Olympiad Test: Quadrilaterals - 1 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Olympiad Test: Quadrilaterals - 1 resources.

Olympiad Test: Quadrilaterals - 1 MCQs with Answers

Prepare for the Olympiad Test: Quadrilaterals - 1 within the Class 8 exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Olympiad Test: Quadrilaterals - 1

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Olympiad Test: Quadrilaterals - 1 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup