IIT JAM Exam > IIT JAM Tests > Sequences And Series Of Real Numbers -2 - IIT JAM MCQ
Sequences And Series Of Real Numbers -2 - IIT JAM MCQ
Test Description
21 Questions MCQ Test - Sequences And Series Of Real Numbers -2
Sequences And Series Of Real Numbers -2 for IIT JAM 2025 is part of IIT JAM preparation. The Sequences And Series Of Real Numbers -2 questions and answers have been prepared
according to the IIT JAM exam syllabus.The Sequences And Series Of Real Numbers -2 MCQs are made for IIT JAM 2025 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Sequences And Series Of Real Numbers -2 below.
Solutions of Sequences And Series Of Real Numbers -2 questions in English are available as part of our course for IIT JAM & Sequences And Series Of Real Numbers -2 solutions in
Hindi for IIT JAM course.
Download more important topics, notes, lectures and mock test series for IIT JAM Exam by signing up for free. Attempt Sequences And Series Of Real Numbers -2 | 21 questions in 60 minutes | Mock test for IIT JAM preparation | Free important questions MCQ to study for IIT JAM Exam | Download free PDF with solutions
Sequences And Series Of Real Numbers -2 - Question 1
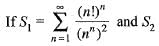
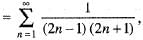 then which one of the following statement is correct?
then which one of the following statement is correct?
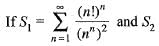
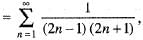 then which one of the following statement is correct?
then which one of the following statement is correct?
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 1
Sequences And Series Of Real Numbers -2 - Question 2
For which real number m does the infinite series 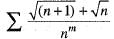 converge
converge
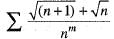 converge
converge
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 2
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 3
Sequences And Series Of Real Numbers -2 - Question 4
Let the sequence be 1 × 2, 3 × 22, 5 × 23, 7 × 24, 9 × 25……… then this sequence is _________
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 4
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 5
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 6
Sequences And Series Of Real Numbers -2 - Question 7
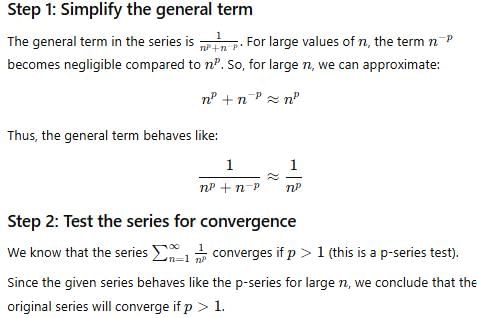
Let Tr be the r th term of an A.P., for r = 1, 2, 3, … If for some positive integers m, n, we have Tm = 1/n and Tn = 1/m, then Tm n equals
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 7
Sequences And Series Of Real Numbers -2 - Question 8
For what value of x the infinite series 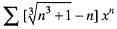 converges, if
converges, if
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 8
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 9
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 10
Sequences And Series Of Real Numbers -2 - Question 11
For a positive term series ∑an , the ratio test states that
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 11
Sequences And Series Of Real Numbers -2 - Question 12
The third term of a geometric progression is 4. The product of the first five terms is
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 12
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 13
Sequences And Series Of Real Numbers -2 - Question 14
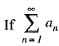 converges, then lim(n-->∞) (an-1)/n1/n is equal to
converges, then lim(n-->∞) (an-1)/n1/n is equal to
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 14
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 15
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 16
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 17
Sequences And Series Of Real Numbers -2 - Question 18
The radius of convergent of the series (1+0.2)/1 + (x+0.2)2/2 +.......+ (x+0.2)n/n +.....
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 18
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 19
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 20
Detailed Solution for Sequences And Series Of Real Numbers -2 - Question 21
Information about Sequences And Series Of Real Numbers -2 Page
In this test you can find the Exam questions for Sequences And Series Of Real Numbers -2 solved & explained in the simplest way possible.
Besides giving Questions and answers for Sequences And Series Of Real Numbers -2, EduRev gives you an ample number of Online tests for practice
Download as PDF


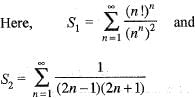

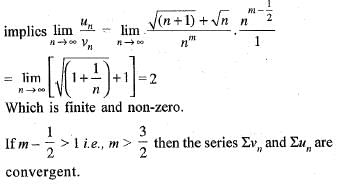

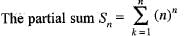
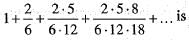
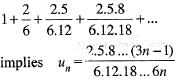
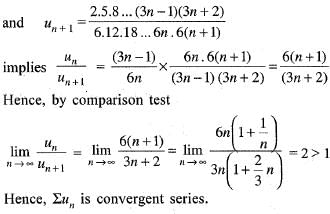
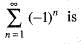
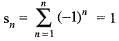 or 0 as n is odd or even implies {Sn} - { 1 , 0 , 1 , 0 , . . . }
or 0 as n is odd or even implies {Sn} - { 1 , 0 , 1 , 0 , . . . }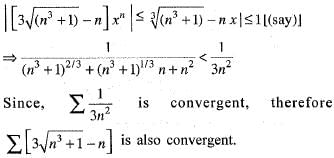
 convergent for
convergent for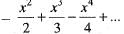 is convergent.
is convergent.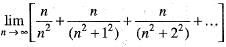 is equal to
is equal to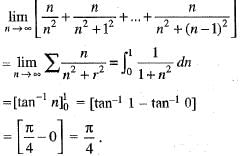
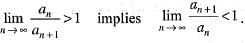
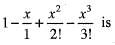
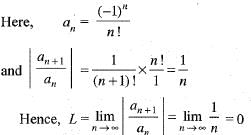
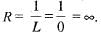
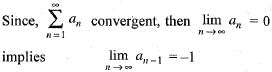
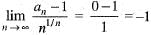
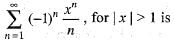
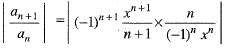
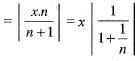
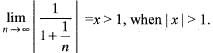
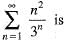
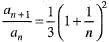

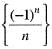 is equal to
is equal to 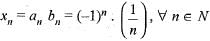
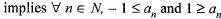

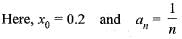
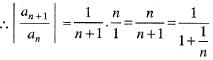
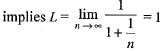
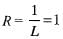
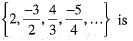
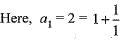

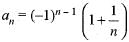
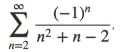
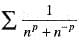 converges, if
converges, if