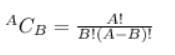Test: JEE Previous Year Questions- Binomial Numbers - JEE MCQ
14 Questions MCQ Test - Test: JEE Previous Year Questions- Binomial Numbers
If in the expansion of (1 + x)m (1 – x)n, the co-efficients of x and x2 are 3 and – 6 respectively, then m is [JEE 99,2 ]
Find the largest co-efficient in the expansion of (1 + x)n, given that the sum of co-efficients of the terms in its expansion is 4096. [REE 2000 (Mains)]
In the binomial expansion of (a - b)n, n ³ 5, the sum of the 5th and 6th terms is zero. Then equals.
Find the coefficient of x49 in the polynomial [REE 2001 (Mains), 3] where Cr = 50Cr
The sum , (where
= O if P < q) is maximum when m is [JEE 2002 (Scr.), 3]
(a) Coefficient of t24 in the expansion of (1 + t2)12 (1 + t12) (1 + t24) is [JEE 2003 (Scr.), 3]
(b) Prove that :
[JEE 2003 (Mains),2]
n_1Cr = (k2 – 3). nCr + 1, if k Î [JEE 2004 (Scr.)]
n-1Cr = (k2 - 3). nCr + 1 [JEE 2004 (Scr.)]
The value of
is, where
= nCr [JEE 2005 (Scr.)]
The number of seven digit integers, with sum of the digits equal to 10 and formed by using the digits 1, 2 and 3 only, is [JEE 2009]
For r = 0, 1, ...., 10 let Ar, Br, Cr denote, respectively, the coefficient of xr in the expansions of (1 + x)10, (1 + x)20 and (1 + x)30. Then is equal to [JEE 2010]
Let an denote the number of all n-digit positive integers formed by the digits 0, 1 or both such that no consecutive digits in them are 0. Let bn = the number of such n-digit integers ending with digit 1 and cn = the number of such n-digit integers ending with digit 0. [JEE 2012]
Which of the following is correct ?
Let an denote the number of all n-digit positive integers formed by the digits 0, 1 or both such that no consecutive digits in them are 0. Let bn = the number of such n-digit integers ending with digit 1 and cn = the number of such n-digit integers ending with digit 0. [JEE 2012]
The value of b6 is