CAT DI And LR Mock Test - 2 - CAT MCQ
25 Questions MCQ Test - CAT DI And LR Mock Test - 2
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
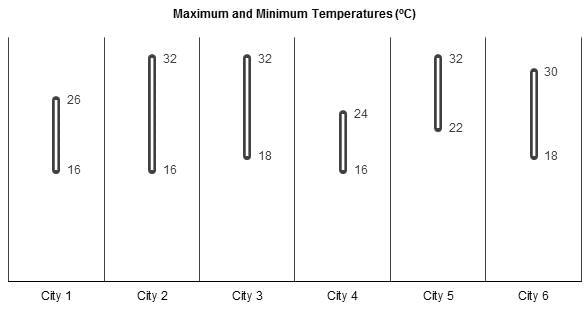
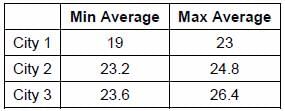
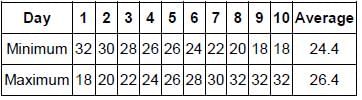
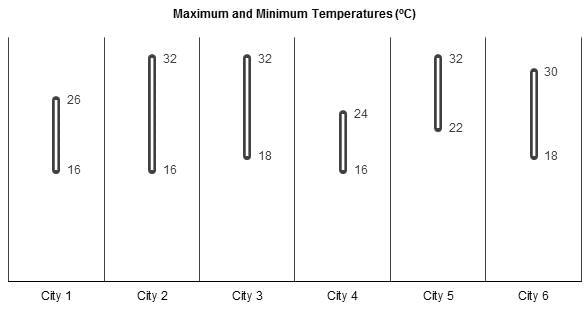
A meteorological agency measured the temperatures (in oC) across six cities for ten consecutive days. Every day, the agency measured the temperature in each city exactly once and at a specified time of the day. The agency also observed that, during this ten-day period, the temperature in any city across two consecutive days either remained constant or changed by exactly 2oC. The following chart provides the maximum and the minimum temperature recorded during the ten days for each city.
Q. If it is known that the average temperature over the ten days in one of the six cities was 25oC, which of the following cities can it be?

DIRECTIONS for questions: Answer the questions on the basis of the information given below.
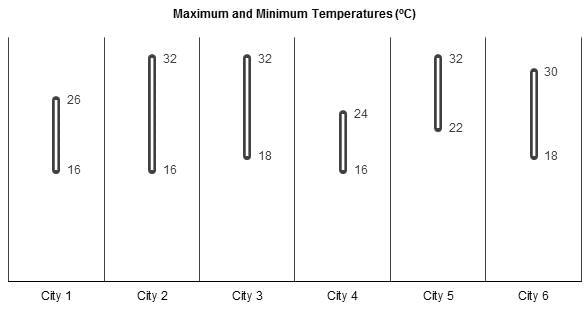
A meteorological agency measured the temperatures (in oC) across six cities for ten consecutive days. Every day, the agency measured the temperature in each city exactly once and at a specified time of the day. The agency also observed that, during this ten-day period, the temperature in any city across two consecutive days either remained constant or changed by exactly 2oC. The following chart provides the maximum and the minimum temperature recorded during the ten days for each city.
Q. If any day on which all the six cities had the same temperature is called an ‘isothermal’ day, then what is the maximum possible number of ‘isothermal’ days during the given period?

DIRECTIONS for questions: Answer the questions on the basis of the information given below.
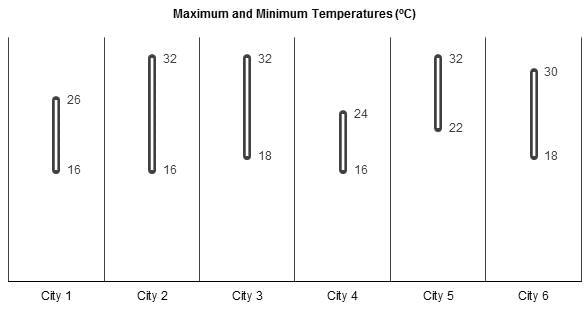
A meteorological agency measured the temperatures (in oC) across six cities for ten consecutive days. Every day, the agency measured the temperature in each city exactly once and at a specified time of the day. The agency also observed that, during this ten-day period, the temperature in any city across two consecutive days either remained constant or changed by exactly 2oC. The following chart provides the maximum and the minimum temperature recorded during the ten days for each city.
Q. On which of the following days can all the six cities have a temperature of 22oC?

DIRECTIONS for questions: Answer the questions on the basis of the information given below.
A meteorological agency measured the temperatures (in oC) across six cities for ten consecutive days. Every day, the agency measured the temperature in each city exactly once and at a specified time of the day. The agency also observed that, during this ten-day period, the temperature in any city across two consecutive days either remained constant or changed by exactly 2oC. The following chart provides the maximum and the minimum temperature recorded during the ten days for each city.
Q. If the temperature of City 3 is 26oC on day 5, which of the following can be the average temperature for this city over the ten days?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
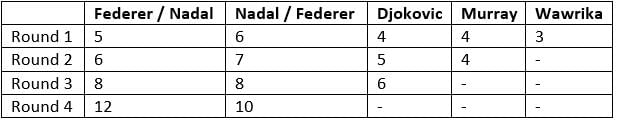
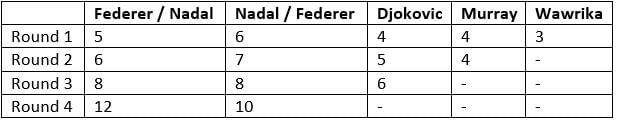
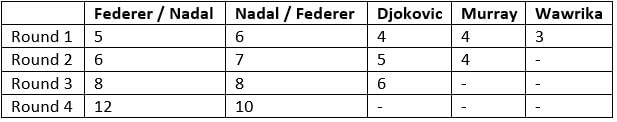
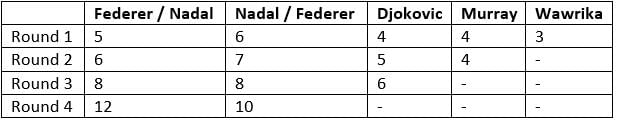
A twenty-two-member panel was constituted to select the best tennis player in the world from five players - Federer, Nadal, Djokovic, Murray and Wawrinka. This selection was done in four rounds, such that in each round, the player that received the minimum number of votes was eliminated. The player that received the maximum number of votes in the final round was declared the best tennis player in the world. All the twenty-two members voted in all the four rounds and in each round, one member can vote for exactly one player. The following table gives the total number of votes received by each player across all the rounds:
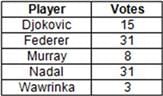
Further, it is also known that,
i. Murray was the only player who received the same number of votes in two or more rounds.
ii. all the members who voted for a specific player in a round voted for the same player in all the subsequent rounds unless he was eliminated.
iii. there were exactly two rounds in which the number of votes received by all the players were distinct, and exactly two rounds in which exactly two players received the same number of votes, while the others received a distinct number of votes each.
iv. in none of the rounds did more than one player receive the least number of votes.
Q. How many votes did Federer receive in the third round?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
A twenty-two-member panel was constituted to select the best tennis player in the world from five players - Federer, Nadal, Djokovic, Murray and Wawrinka. This selection was done in four rounds, such that in each round, the player that received the minimum number of votes was eliminated. The player that received the maximum number of votes in the final round was declared the best tennis player in the world. All the twenty-two members voted in all the four rounds and in each round, one member can vote for exactly one player. The following table gives the total number of votes received by each player across all the rounds:
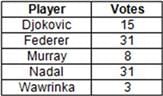
Further, it is also known that,
i. Murray was the only player who received the same number of votes in two or more rounds.
ii. all the members who voted for a specific player in a round voted for the same player in all the subsequent rounds unless he was eliminated.
iii. there were exactly two rounds in which the number of votes received by all the players were distinct, and exactly two rounds in which exactly two players received the same number of votes, while the others received a distinct number of votes each.
iv. in none of the rounds did more than one player receive the least number of votes.
Q. In which of the following rounds did at least two players receive the same number of votes?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
A twenty-two-member panel was constituted to select the best tennis player in the world from five players - Federer, Nadal, Djokovic, Murray and Wawrinka. This selection was done in four rounds, such that in each round, the player that received the minimum number of votes was eliminated. The player that received the maximum number of votes in the final round was declared the best tennis player in the world. All the twenty-two members voted in all the four rounds and in each round, one member can vote for exactly one player. The following table gives the total number of votes received by each player across all the rounds:
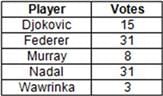
Further, it is also known that,
i. Murray was the only player who received the same number of votes in two or more rounds.
ii. all the members who voted for a specific player in a round voted for the same player in all the subsequent rounds unless he was eliminated.
iii. there were exactly two rounds in which the number of votes received by all the players were distinct, and exactly two rounds in which exactly two players received the same number of votes, while the others received a distinct number of votes each.
iv. in none of the rounds did more than one player receive the least number of votes.
Q. If Federer received more votes than exactly three other players in the first round, which player was declared the best tennis player in the world?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
A twenty-two-member panel was constituted to select the best tennis player in the world from five players - Federer, Nadal, Djokovic, Murray and Wawrinka. This selection was done in four rounds, such that in each round, the player that received the minimum number of votes was eliminated. The player that received the maximum number of votes in the final round was declared the best tennis player in the world. All the twenty-two members voted in all the four rounds and in each round, one member can vote for exactly one player. The following table gives the total number of votes received by each player across all the rounds:
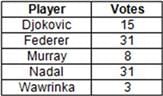
Further, it is also known that,
i. Murray was the only player who received the same number of votes in two or more rounds.
ii. all the members who voted for a specific player in a round voted for the same player in all the subsequent rounds unless he was eliminated.
iii. there were exactly two rounds in which the number of votes received by all the players were distinct, and exactly two rounds in which exactly two players received the same number of votes, while the others received a distinct number of votes each.
iv. in none of the rounds did more than one player receive the least number of votes.
Q. What is the total number of members in the panel who had to change the player that they voted for during any of the four rounds of voting?
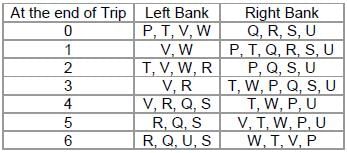
Four persons, Pavan, Tarun, Venky, and Waqar, are standing on the left bank of a river, whereas another four persons, Qureshi, Ramesh, Sanjay, and Uday, are standing on the right bank of the river. A ferry, which can be used to cross the river and go to the other bank, is present initially on the left bank. The ferry can carry a maximum of two people at a time. However, there must be at least one person in the ferry in order to guide it to the other bank. The travel of the ferry from one bank to the other is called a trip. The eight persons have certain preferences regarding how they would like to travel in the ferry. The following information is known about their preferences:
i. If Pavan is present in the ferry, Tarun must also be present in the ferry.
ii. If Venky travels to a bank on which Sanjay is already present, Sanjay will immediately travel to the opposite bank.
iii. Qureshi will travel in the ferry only when Uday is not present in the ferry.
iv. Venky and Waqar cannot travel together in the ferry.
Q. What is the minimum number of trips the ferry must make so that all the eight people are present on the same bank?
Four persons, Pavan, Tarun, Venky, and Waqar, are standing on the left bank of a river, whereas another four persons, Qureshi, Ramesh, Sanjay, and Uday, are standing on the right bank of the river. A ferry, which can be used to cross the river and go to the other bank, is present initially on the left bank. The ferry can carry a maximum of two people at a time. However, there must be at least one person in the ferry in order to guide it to the other bank. The travel of the ferry from one bank to the other is called a trip. The eight persons have certain preferences regarding how they would like to travel in the ferry. The following information is known about their preferences:
i. If Pavan is present in the ferry, Tarun must also be present in the ferry.
ii. If Venky travels to a bank on which Sanjay is already present, Sanjay will immediately travel to the opposite bank.
iii. Qureshi will travel in the ferry only when Uday is not present in the ferry.
iv. Venky and Waqar cannot travel together in the ferry.
Q. If Tarun and Ramesh are in the ferry during its second trip, what is the minimum number of trips in which all the people on the left bank could go to the right bank and vice versa?
Four persons, Pavan, Tarun, Venky, and Waqar, are standing on the left bank of a river, whereas another four persons, Qureshi, Ramesh, Sanjay, and Uday, are standing on the right bank of the river. A ferry, which can be used to cross the river and go to the other bank, is present initially on the left bank. The ferry can carry a maximum of two people at a time. However, there must be at least one person in the ferry in order to guide it to the other bank. The travel of the ferry from one bank to the other is called a trip. The eight persons have certain preferences regarding how they would like to travel in the ferry. The following information is known about their preferences:
i. If Pavan is present in the ferry, Tarun must also be present in the ferry.
ii. If Venky travels to a bank on which Sanjay is already present, Sanjay will immediately travel to the opposite bank.
iii. Qureshi will travel in the ferry only when Uday is not present in the ferry.
iv. Venky and Waqar cannot travel together in the ferry.
Q. If Ramesh and Uday are in the ferry during its second trip, who among the following could not have travelled in the ferry during its first trip?
Four persons, Pavan, Tarun, Venky, and Waqar, are standing on the left bank of a river, whereas another four persons, Qureshi, Ramesh, Sanjay, and Uday, are standing on the right bank of the river. A ferry, which can be used to cross the river and go to the other bank, is present initially on the left bank. The ferry can carry a maximum of two people at a time. However, there must be at least one person in the ferry in order to guide it to the other bank. The travel of the ferry from one bank to the other is called a trip. The eight persons have certain preferences regarding how they would like to travel in the ferry. The following information is known about their preferences:
i. If Pavan is present in the ferry, Tarun must also be present in the ferry.
ii. If Venky travels to a bank on which Sanjay is already present, Sanjay will immediately travel to the opposite bank.
iii. Qureshi will travel in the ferry only when Uday is not present in the ferry.
iv. Venky and Waqar cannot travel together in the ferry.
Q. After the ferry completed three trips, which of the following pairs of persons cannot be on the left bank?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
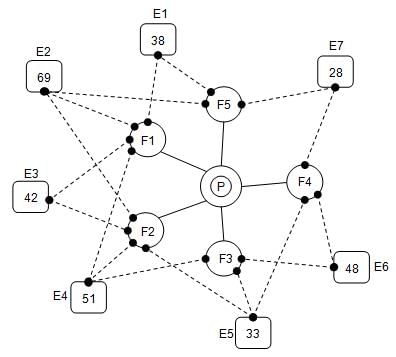
The capital city of the kingdom of Alami has the royal palace in the centre, with five fortifications, labelled F1 to F5, surrounding the palace. All the Alami soldiers are always present at the royal palace unless ordered to move to a fortification. An enemy kingdom Bagami planned to attack the royal palace and built camps around the fortifications. The Bagamis built seven camps - E1 to E7 - around the Alami fortifications and each Bagami camp had a certain number of soldiers. The soldiers from a given Bagami camp can attack only some of the fortifications, as indicated in the diagram below. However, all the soldiers from a single Bagami camp need not necessarily attack the same fortification. The Alami king came to know about this and was planning to send his soldiers to the fortifications.
The following diagram shows the royal palace (P), fortifications (F1 to F5) and enemy (Bagami) camps (E1 to E7). The solid lines in the figure represent the respective fortification to which the Alami soldiers can be deployed from the palace. The dotted lines from each enemy camp represent the fortifications that the soldiers from that enemy camp can attack. The numbers inside each enemy camp represent the number of soldiers stationed in that camp.
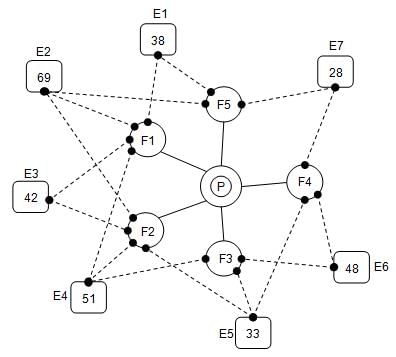
Assume that all the Bagami soldiers attack the fortifications that they are assigned to at the same time and each soldier will attack exactly one fortification. Also, a higher numerical strength in terms of soldiers will assure victory to any side in such a manner that, if, say, 10 Bagami soldiers attack a fortification with 11 Alami soldiers, all the Bagami soldiers will die and the fortification will remain safe, but with only 1 Alami soldier remaining alive. If the number of Bagami soldiers attacking a fortification is equal to the number of Alami soldiers at the fortification, all the soldiers belonging to both the sides will die but the fortification will remain safe. In a similar manner, if the number of Bagami soldiers attacking is in excess of the number of Alami soldiers, the fortification will be destroyed, but with only the excess number of Bagami soldiers remaining alive.
Q. What is the minimum number of soldiers the Alami king must deploy to all the fortifications put together in order to ensure that none of the fortifications is destroyed?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
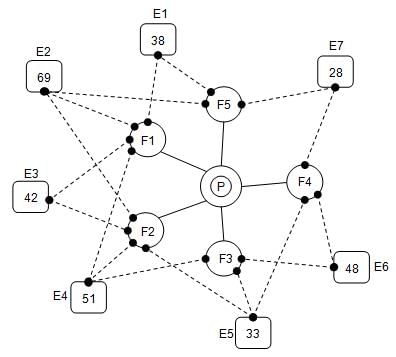
The capital city of the kingdom of Alami has the royal palace in the centre, with five fortifications, labelled F1 to F5, surrounding the palace. All the Alami soldiers are always present at the royal palace unless ordered to move to a fortification. An enemy kingdom Bagami planned to attack the royal palace and built camps around the fortifications. The Bagamis built seven camps - E1 to E7 - around the Alami fortifications and each Bagami camp had a certain number of soldiers. The soldiers from a given Bagami camp can attack only some of the fortifications, as indicated in the diagrambelow. However, all the soldiers from a single Bagami camp need not necessarily attack the same fortification. The Alami king came to know about this and was planning to send his soldiers to the fortifications.
The following diagram shows the royal palace (P), fortifications (F1 to F5) and enemy (Bagami) camps (E1 to E7). The solid lines in the figure represent the respective fortification to which the Alami soldiers can be deployed from the palace. The dotted lines from each enemy camp represent the fortifications that the soldiers from that enemy camp can attack. The numbers inside each enemy camp represent the number of soldiers stationed in that camp.
Assume that all the Bagami soldiers attack the fortifications that they are assigned to at the same time and each soldier will attack exactly one fortification. Also, a higher numerical strength in terms of soldiers will assure victory to any side in such a manner that, if, say, 10 Bagami soldiers attack a fortification with 11 Alami soldiers, all the Bagami soldiers will die and the fortification will remain safe, but with only 1 Alami soldier remaining alive. If the number of Bagami soldiers attacking a fortification is equal to the number of Alami soldiers at the fortification, all the soldiers belonging to both the sides will die but the fortification will remain safe. In a similar manner, if the number of Bagami soldiers attacking is in excess of the number of Alami soldiers, the fortification will be destroyed, but with only the excess number of Bagami soldiers remaining alive.
Q. If the Alami king wants to save at least three fortifications from being destroyed, what is the minimum number of soldiers that he must deploy?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
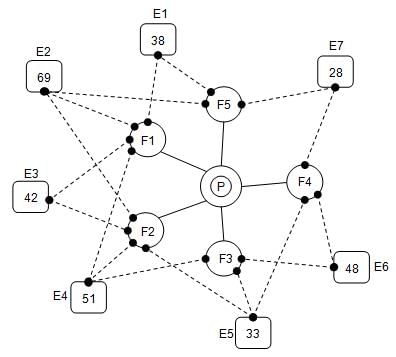
The capital city of the kingdom of Alami has the royal palace in the centre, with five fortifications, labelled F1 to F5, surrounding the palace. All the Alami soldiers are always present at the royal palace unless ordered to move to a fortification. An enemy kingdom Bagami planned to attack the royal palace and built camps around the fortifications. The Bagamis built seven camps - E1 to E7 - around the Alami fortifications and each Bagamicamp had a certain number of soldiers. The soldiers from a given Bagami camp can attack only some of the fortifications, as indicated in the diagrambelow. However, all the soldiers from a single Bagami camp need not necessarily attack the same fortification. The Alami king came to know about this and was planning to send his soldiers to the fortifications.
The following diagram shows the royal palace (P), fortifications (F1 to F5) and enemy (Bagami) camps (E1 to E7). The solid lines in the figure represent the respective fortification to which the Alami soldiers can be deployed from the palace. The dotted lines from each enemy camp represent the fortifications that the soldiers from that enemy camp can attack. The numbers inside each enemy camp represent the number of soldiers stationed in that camp.
Assume that all the Bagami soldiers attack the fortifications that they are assigned to at the same time and each soldier will attack exactly one fortification. Also, a higher numerical strength in terms of soldiers will assure victory to any side in such a manner that, if, say, 10 Bagami soldiers attack a fortification with 11 Alami soldiers, all the Bagami soldiers will die and the fortification will remain safe, but with only 1 Alami soldier remaining alive. If the number of Bagami soldiers attacking a fortification is equal to the number of Alami soldiers at the fortification, all the soldiers belonging to both the sides will die but the fortification will remain safe. In a similar manner, if the number of Bagami soldiers attacking is in excess of the number of Alami soldiers, the fortification will be destroyed, but with only the excess number of Bagami soldiers remaining alive.
Q. If it is known that the Bagami king is planning to send an equal number of soldiers from each enemy camp to all the possible fortifications from that enemy camp, what is the number of soldiers that will be killed during the attack, if no fortification
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
The capital city of the kingdom of Alami has the royal palace in the centre, with five fortifications, labelled F1 to F5, surrounding the palace. All the Alami soldiers are always present at the royal palace unless ordered to move to a fortification. An enemy kingdom Bagami planned to attack the royal palace and built camps around the fortifications. The Bagamis built seven camps - E1 to E7 - around the Alami fortifications and each Bagami camp had a certain number of soldiers. The soldiers from a given Bagami camp can attack only some of the fortifications, as indicated in the diagrambelow. However, all the soldiers from a single Bagami camp need not necessarily attack the same fortification. The Alami king came to know about this and was planning to send his soldiers to the fortifications.
The following diagram shows the royal palace (P), fortifications (F1 to F5) and enemy (Bagami) camps (E1 to E7). The solid lines in the figure represent the respective fortification to which the Alami soldiers can be deployed from the palace. The dotted lines from each enemy camp represent the fortifications that the soldiers from that enemy camp can attack. The numbers inside each enemy camp represent the number of soldiers stationed in that camp.
Assume that all the Bagami soldiers attack the fortifications that they are assigned to at the same time and each soldier will attack exactly one fortification. Also, a higher numerical strength in terms of soldiers will assure victory to any side in such a manner that, if, say, 10 Bagami soldiers attack a fortification with 11 Alami soldiers, all the Bagami soldiers will die and the fortification will remain safe, but with only 1 Alami soldier remaining alive. If the number of Bagami soldiers attacking a fortification is equal to the number of Alami soldiers at the fortification, all the soldiers belonging to both the sides will die but the fortification will remain safe. In a similar manner, if the number of Bagami soldiers attacking is in excess of the number of Alami soldiers, the fortification will be destroyed, but with only the excess number of Bagami soldiers remaining alive.
Q. During the attack, if a total of 612 soldiers died on both the sides put together, what is the minimum number of fortifications that would not have been destroyed?
DIRECTIONS for questions: Each question is followed by two statements, I and II, giving certain data. You have to decide whether the information provided in the statements is sufficient for answering the question and mark your answer as How many students from the top 20 rankers in the institute are Commerce graduates if it is known that none of the top five rankers are Commerce graduates?
I. None of the last four out of the top 20 rankers are Commerce graduates.
II. At least 8 of the top 15 rankers and not more than 8 of the last fifteen out of 20 top rankers are Commerce graduates.
DIRECTIONS for questions: Each question is followed by two statements, I and II, giving certain data. You have to decide whether the information provided in the statements is sufficient for answering the question and mark your answer as
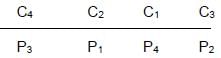
Four cities - C1, C2, C3 and C4 - lie in a straight line, not necessarily in the same order, with the distance between any two adjacent cities being equal. From each of these cities, exactly one person among P1, P2, P3 and P4 starts simultaneously towards one of the other cities. From which city did P4 start?
I. From the starting point of P1, he can reach either of the cities C1 and C4 in the same time.
II. Even if P3 is twice as fast as P2, he cannot reach city C3 by the time P2 reaches the city C1.
DIRECTIONS for questions: Each question is followed by two statements, I and II, giving certain data. You have to decide whether the information provided in the statements is sufficient for answering the question and mark your answer as At a primary school, girls and boys were going from their morning assembly to their classrooms in separate lines. They had to move through a narrow doorway, where the two lines had to merge into one. After the lines merged, no girl had overtaken any other girl and no boy had overtaken any other boy. Initially, Meena was in the 4th position from the front in the girls line and her brother was at the 6th position from the end in the boys line. If after the lines merged, there were eight students ahead of Meena, how many boys were behind Meena?
I. There were three boys between Meena and her brother.
II. After the merger, Meena was ahead of her brother.
DIRECTIONS for questions: Each question is followed by two statements, I and II, giving certain data. You have to decide whether the information provided in the statements is sufficient for answering the question and mark your answer as Vijay bought a new television costing at least Rs.10,000 after exchanging his old television with the new one. If exchanging the old television resulted in a 30% discount in the price of the new television, find the amount Vijay paid for purchasing the new television.
I. He borrowed an amount which was equal to 20% of the cost of the old television from his friend and raised the remaining amount from his personal savings.
II. His total personal savings were Rs.6400.
DIRECTIONS for questions: Each question is followed by two statements, I and II, giving certain data. You have to decide whether the information provided in the statements is sufficient for answering the question and mark your answer as How many among the four players - A, B, C and D scored a century?
I. Ram said A and B scored centuries while Mohan said at least two among A, C and D scored centuries.
II. Ram always tells the truth while Mohan always lies.
DIRECTIONS for questions: Each question is followed by two statements, I and II, giving certain data. You have to decide whether the information provided in the statements is sufficient for answering the question and mark your answer as I have a total of 125 fruits - apples and oranges put together - which are to be distributed to 12 boys and 9 girls of a class, such that each girl gets at least one apple but no orange but each boy gets at least one apple and at least one orange. Can apples be distributed equally to all the 21 students? Assume that the number of fruits of any variety, distributed for any person is always an integer.
I. The number of apples is a multiple of 7.
II. The oranges can be distributed equally to all the boys.
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
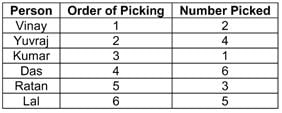
Six friends, Das, Kumar, Lal, Ratan, Vinay, and Yuvraj, wanted to play a game of Ludo and decided to draw lots to determine the order in which they play. For this purpose, they took six pieces of paper and wrote down a different number, from 1 to 6, on each piece of paper and then put them all in a bag. Each of them picked a piece of paper from the bag, without peeping into the bag, one after the other. They then saw the numbers that each of them picked and decided the order in which they will play. After they finished the game, they made the following statements, all of which are true:
Das : I picked a number which was greater than the number picked by Lal and the number four was already picked before I picked a number.
Kumar : I was the third person to pick a number and the number two was already picked by that time.
Lal : I picked a number after Ratan did and the number picked by Ratan was lower than mine.
Ratan : I picked a number lower than the number picked by Yuvraj and I picked the number after Das did.
Vinay : Ratan and I picked consecutive numbers and neither of us picked the lowest number.
Yuvraj : I did not pick the highest number and nor was I the first to pick a number.
Q. Which number did Yuvraj pick?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
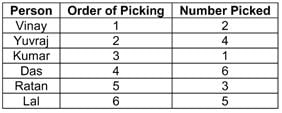
Six friends, Das, Kumar, Lal, Ratan, Vinay, and Yuvraj, wanted to play a game of Ludo and decided to draw lots to determine the order in which they play. For this purpose, they took six pieces of paper and wrote down a different number, from 1 to 6, on each piece of paper and then put them all in a bag. Each of them picked a piece of paper from the bag, without peeping into the bag, one after the other. They then saw the numbers that each of them picked and decided the order in which they will play. After they finished the game, they made the following statements, all of which are true:
Das : I picked a number which was greater than the number picked by Lal and the number four was already picked before I picked a number.
Kumar : I was the third person to pick a number and the number two was already picked by that time.
Lal : I picked a number after Ratan did and the number picked by Ratan was lower than mine.
Ratan : I picked a number lower than the number picked by Yuvraj and I picked the number after Das did.
Vinay : Ratan and I picked consecutive numbers and neither of us picked the lowest number.
Yuvraj : I did not pick the highest number and nor was I the first to pick a number.
Q. Who was the first person to pick a number?
DIRECTIONS for questions: Answer the questions on the basis of the information given below.
Six friends, Das, Kumar, Lal, Ratan, Vinay, and Yuvraj, wanted to play a game of Ludo and decided to draw lots to determine the order in which they play. For this purpose, they took six pieces of paper and wrote down a different number, from 1 to 6, on each piece of paper and then put them all in a bag. Each of them picked a piece of paper from the bag, without peeping into the bag, one after the other. They then saw the numbers that each of them picked and decided the order in which they will play. After they finished the game, they made the following statements, all of which are true:
Das : I picked a number which was greater than the number picked by Lal and the number four was already picked before I picked a number.
Kumar : I was the third person to pick a number and the number two was already picked by that time.
Lal : I picked a number after Ratan did and the number picked by Ratan was lower than mine.
Ratan : I picked a number lower than the number picked by Yuvraj and I picked the number after Das did.
Vinay : Ratan and I picked consecutive numbers and neither of us picked the lowest number.
Yuvraj : I did not pick the highest number and nor was I the first to pick a number.
Q. Which of the following statements is true?