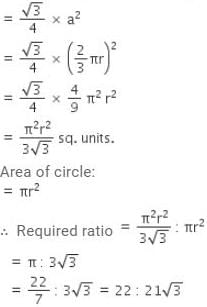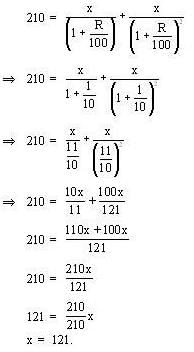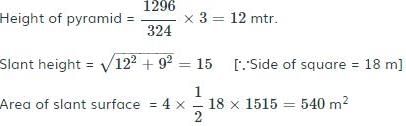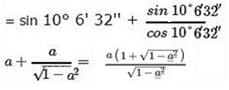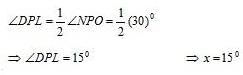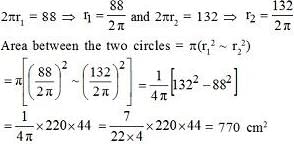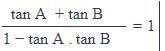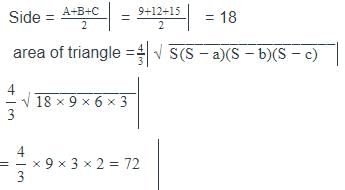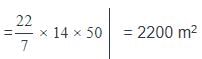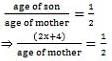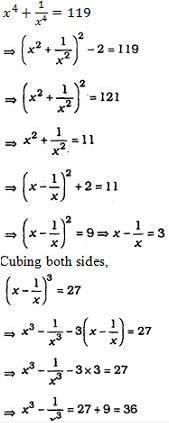All Courses
Get the App Signup / Login
Sign in Open App
Electronics and Communication Engineering (ECE) Exam  >  Electronics and Communication Engineering (ECE) Tests  >  RRB JE ECE (CBT I) Mock Test- 1 - Electronics and Communication Engineering (ECE) MCQ

RRB JE ECE (CBT I) Mock Test- 1 - Electronics and Communication Engineering (ECE) MCQ


Test Description

30 Questions MCQ Test - RRB JE ECE (CBT I) Mock Test- 1

RRB JE ECE (CBT I) Mock Test- 1 for Electronics and Communication Engineering (ECE) 2024 is part of Electronics and Communication Engineering (ECE) preparation. The RRB JE ECE (CBT I) Mock Test- 1 questions and answers have been prepared according to the Electronics and Communication Engineering (ECE) exam syllabus.The RRB JE ECE (CBT I) Mock Test- 1 MCQs are made for Electronics and Communication Engineering (ECE) 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for RRB JE ECE (CBT I) Mock Test- 1 below.
Solutions of RRB JE ECE (CBT I) Mock Test- 1 questions in English are available as part of our course for Electronics and Communication Engineering (ECE) & RRB JE ECE (CBT I) Mock Test- 1 solutions in Hindi for Electronics and Communication Engineering (ECE) course. Download more important topics, notes, lectures and mock test series for Electronics and Communication Engineering (ECE) Exam by signing up for free. Attempt RRB JE ECE (CBT I) Mock Test- 1 | 100 questions in 90 minutes | Mock test for Electronics and Communication Engineering (ECE) preparation | Free important questions MCQ to study for Electronics and Communication Engineering (ECE) Exam | Download free PDF with solutions
RRB JE ECE (CBT I) Mock Test- 1 - Question 1
Save

If P = sin θ (sin θ + sin 3θ), then

Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 1
We have

P = sin θ (sin θ + sin 3θ)

= sin θ (2sin 2θ. cos θ)

[∵ sin C + sin D = 2sin (C+D/2).cos (C−D/2)]

⇒ P = sin 2θ (2sin θ. cos θ)

= sin 2θ.sin 2θ = sin2 2θ

P = sin2 2θ ≥ 0

for all real θ

RRB JE ECE (CBT I) Mock Test- 1 - Question 2
Save

The value of the expression

Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 2

4(n+m-1+m-n-2m)x5(m-1+m+n-2-2m-n)x3(m-n+m+n-2-2m+2

= 4-1 x 5-3 x 30

= 1/4 x 1/125 x 1 = 1/500

1 Crore+ students have signed up on EduRev. Have you? Download the App
RRB JE ECE (CBT I) Mock Test- 1 - Question 3
Save

Perimeter of an equilateral triangle is equal to the circumference of a circle. The ratio of their areas is :

(Use π= 22/7)

Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 3
Accordingly to the question,

3a = 2πr

⇒ a = 2/3πr

Area of an equilateral triangle:

RRB JE ECE (CBT I) Mock Test- 1 - Question 4
Save

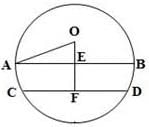
AB = 8 cm and CD = 6 cm are parallel chords on the same side of the centre of a circle. The distance between them is 1cm. The radius of the circle is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 4

Let OE= x

OF = x+1, OA=OC= r

AE= 4cm, CF= 3cm

From ∆ OAE

r2 = 16+x2

x2 = r2- 16 ------(1)

From ∆ OCF

(x+1)2 = r2 - 9 ------ (2)

By equation (2) - (1)

x = 3cm

r = 5cm

RRB JE ECE (CBT I) Mock Test- 1 - Question 5
Save

A tank that would normally be filled in 8 hours is now taking 2 hours more because of a leak. In how much time will the leak empty the full tank?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 5
Time taken by the inlet pipe to fill the tank = 8 h

Time taken to fill the tank due to leak = 8 + 2 = 10 h

Let the leak take x hours to empty the filled tank.

Now, according to the question:

Time taken by leak to empty the tank = X = 40 hours

RRB JE ECE (CBT I) Mock Test- 1 - Question 6
Save

A sum of Rs. 210 was taken as a loan. This is to be paid back in two equal installments. If the rate of interest be 10% compounded annually, then the value of each installment is
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 6

Let x be the value of installment.

Principal = Present value of x for 1 year + Present value of x for 2 years

RRB JE ECE (CBT I) Mock Test- 1 - Question 7
Save

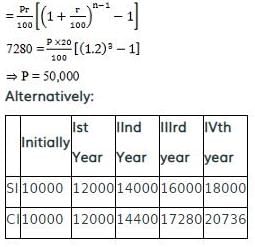
The difference between simple and compound interest for the fourth year is ₹ 7280 at 20% p.a. What is the sum?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 7

Difference between CI and SI for the nth year

CI for 4th year = 20736 - 17280 = 3456

SI for 4th year = 2000

Difference between CI and SI = 1456 for Rs10000

So, the difference of Rs7280 is for Rs50,000

RRB JE ECE (CBT I) Mock Test- 1 - Question 8
Save

At present, the sum of ages of R and K is 63 years. The ratio of their ages after 7 years will be 7:4; what is the present age of R?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 8
Let the present age of R is x

⇒ present age of K is 63 - x

7 years later

R's age = x+7

K's age = 63-x+7 = 70-x

⇒ (x+7)/(70-x) = 7/4

⇒ 4x+28 = 490-7x

⇒ 11x = 462

⇒ x = 42 years

therefore present age of R = 42 years

RRB JE ECE (CBT I) Mock Test- 1 - Question 9
Save

If cosec θ = x + 1/4x, then the value of cosec θ + cot θ is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 9
Let cosec θ + cot θ = k

⇒ cosec θ - cot θ = 1/k

On adding, we get

2 cosec θ = k + 1/k = 2(x+1/4x) = k+1/k

⇒ 2x+1/2x = k+1/k ⇒ k = 2x

RRB JE ECE (CBT I) Mock Test- 1 - Question 10
Save

Base of a right pyramid is a square of area 324 sqm. If the volume of the pyramid is 1296 cu.m., then the area (in m2) of the slant surface is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 10

RRB JE ECE (CBT I) Mock Test- 1 - Question 11
Save

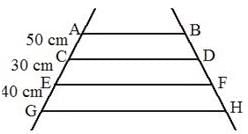
In the given figure AB || CD || EF || GH and BH = 100 cm. Find the value of DF.
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 11

AG/BH = CE/DF

120/100 = 30/DF ⇒ DF = 100×30/120 = 25

RRB JE ECE (CBT I) Mock Test- 1 - Question 12
Save

If sin (10° 6’ 32”) = a, then the value of cos (79° 53’ 28”) + tan (10° 6’ 32”) is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 12

cos 790 53’ 28’’ + tan 100 + 6’ 32’’

RRB JE ECE (CBT I) Mock Test- 1 - Question 13
Save

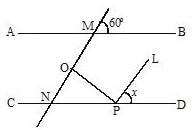
In the figure AB || CD, ∠DPL= 1/2∠NPO, OP is the perpendicular on MN, the value of xº is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 13

∠ONP = 60°

∠OPN = 90° - ∠ONP = 90° -60°

⇒ OPN = 30°

RRB JE ECE (CBT I) Mock Test- 1 - Question 14
Save

Find the area between two concentric circles of circumference 88 cm and 132 cm.
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 14
Let the radius of the inner circle be r1, and the radius of the outer circle be r2.

A.T.Q.,

RRB JE ECE (CBT I) Mock Test- 1 - Question 15
Save

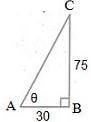
A man moving at a speed of 36 kmph covered the shadow of a tower of height 75 m in 3 seconds. If the height of the person is 1.75 m, the length of the shadow cast by the man in meters is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 15

Distance travelled in 3 seconds with a speed of 36 kmph is =

(5/18)×(3)×(36) = 30m.

From the figure tan θ = BC/AB = 75/30 = 5/2

The height of the person is 1.75 m

Let the length of the shadow be x m.

tan θ = 1.75/x ⇒ 52 = 1.75/x

⇒ x = 1.75/5(2) = 0.7

RRB JE ECE (CBT I) Mock Test- 1 - Question 16
Save

A dress is 25% off. If both the cost and selling prices are ₹ 100 less, the profit would be 35%. Find its cost price?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 16
C.p = (Initial profit + increase in profit %)/Increase in profit%×Amount

C.P = 25+10/10×100

= ₹ 350

RRB JE ECE (CBT I) Mock Test- 1 - Question 17
Save

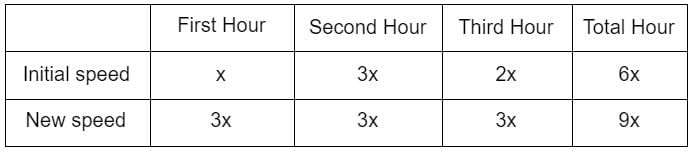
The speed of a car during the second hour of its journey is thrice that in the first hour. Also, its third-hour speed is the average speed of the first two hours. Had the car travelled at the second hour’s speed during all the first three hours, then it would have travelled 150 km more. Find the percentage reduction in time if the original distance covered by the second hour’s speed:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 17

∴ Percentage increase in speed = 3x/6x × 100 = 50%

Since speed is increased by (50%) ½

Therefore, time will reduce by (33.33%) 1/3

RRB JE ECE (CBT I) Mock Test- 1 - Question 18
Save

If A+B = 45°, then find the value of tan A + tan B + tan A tan B.
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 18

A+B = 45°

tan (A+B) = tan 45°

tan A + tanB + tanAtanB = 1

RRB JE ECE (CBT I) Mock Test- 1 - Question 19
Save

If a + b +c = 13, what is the maximum value of (a -3)(b -2) (c + 1) ?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 19
If x+y +z is constant, the product xyz takes maximum value when each of x, y, z takes equal value.

∵ a + b +c = 13

∴ (a -3) + (b -2) + (c + 1) = 13 – 3 – 2 + 1 = 9

For the maximum value of (a -3)(b -2) (c + 1)

= (a -3)= (b -2)= (c + 1)= 9/3 = 3

So, (a -3)(b -2) (c + 1) = 3 × 3 × 3 = 27

RRB JE ECE (CBT I) Mock Test- 1 - Question 20
Save

The length of three medians of a triangle are 9 cm, 12 cm and 15 cm. The area (in sq. cm) of the triangle is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 20

Area of triangle = Area of a triangle formed by taking median as a side of the triangle

A = 9, B = 12, C = 15

RRB JE ECE (CBT I) Mock Test- 1 - Question 21
Save

The floor of a room is of size 4 m x 3 m, and its height is 3 m. The walls and ceiling of the room require painting. The area to be painted is
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 21
Area of the four walls of the room

= 2 x height (length + breadth)

= 2 x 3(4+3) = 42 sq. metre

Area of ceiling = 4 x 3

= 12 sq. metre

Required area = 42 + 12

= 54 sq. metre

RRB JE ECE (CBT I) Mock Test- 1 - Question 22
Save

If 5 girls can embroider a dress in 9 days, then the number of days taken by 3 girls will be _____.
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 22
Days taken by 5 girls to embroider a dress = 9 days

Days taken by 1 girl to embroider a dress = 9 x 5 days

Days taken by 3 girls to embroider a dress = 9 × 5/3 days = 15 days

RRB JE ECE (CBT I) Mock Test- 1 - Question 23
Save

How many metres length of cloth 10 m wide will be required to make a conical tent with base radius of 14 m and height is 48 m?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 23
Slant height of a cone: ( l ) = √(r2 + h2)

= √(142 + 482)

= √196 + 2304

= √2500

l = 50 m

Lateral surface area of a cone = πrl

Hence length of cloth =

= 220 m

RRB JE ECE (CBT I) Mock Test- 1 - Question 24
Save

Two trains are travelling in the same direction at 40 kmph, and 22 kmph completely pass off another in 1 minute. If the length of the first train is 125 metres, what is the length of the second train?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 24
Distance traveled by the relative speed of (40-22) kmph i.e. 18 kmph

In 1 minute= 18×1000/60 = 300 m

∴ Length of second train = (300-125) = 175 m

RRB JE ECE (CBT I) Mock Test- 1 - Question 25
Save

The ratio between the ages of a father and a son at present is 5: 2, respectively. Four years hence the ratio between the ages of the son and his mother will be 1: 2 respectively. What is the ratio between the present ages of the father and the mother, respectively?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 25
Let the present ages of father and son be ‘5x’ and ‘2x’ years, respectively.

After 4 years, Son’s age = (2x + 4) years

According to question

After 4 years

The ratio between the present age of the father and mother = 5x: (4x + 4)

∴ We cannot find the exact value of the ratio.

Age of mother = 2 x (2x+ 4) = 4x+ 8 years

Present age of Mother = 4x + 8 - 4

= 4x +4

The ratio between the present age of the father and mother = 5x: (4x + 4)

∴ We cannot find the exact value of the ratio.

RRB JE ECE (CBT I) Mock Test- 1 - Question 26
Save

If x4 + 1/x4 =119 and x > 1, then the value of x3 − 1/x3 is:
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 26

RRB JE ECE (CBT I) Mock Test- 1 - Question 27
Save

The total annual Co2 emissions from various sectors are 5 MMT. In the pie chart given below, the percentage distribution to Co2 emission from various sectors are indicated.

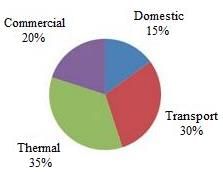
Q. Emission of Domestic sector is how much % of transport and commercial sector combined?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 27

15/50 × 100 = 30%

RRB JE ECE (CBT I) Mock Test- 1 - Question 28
Save

The total annual Co2 emissions from various sectors are 5 MMT. In the pie chart given below, the percentage distribution to Co2 emission from various sectors are indicated.

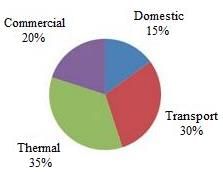
Q. Which of the following sectors have an emission difference of 1 MMT between them?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 28

1 mmt = 20% = Thermal – Domestic

RRB JE ECE (CBT I) Mock Test- 1 - Question 29
Save

Twenty percent of Anuj’s annual salary is equal to seventy-five per cent of Raj’s annual salary. Raj’s monthly salary is 60% of Ravi’s monthly salary. If Ravi’s annual salary is Rs. 1.44 lacs, what is Anuj’s monthly salary?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 29

Given:

20% of Anuj's annual salary = 75% of Raj's annual salary

Raj's annual salary = 60% of Ravi's monthly salary

Ravi's annual salary = Rs. 1.44 lakhs = Rs. 144000

Concept:

20% = 1/5, 75% = 3/4, 60% = 3/5

Calculation:

Ravi's monthly salary = 144000/12 = 12000

60% of Ravi's monthly salary = Raj's annual salary

⇒ (3/5) × 12000 = Rs. 7200 = Raj's annual salary

Now, 1/5 of Anuj's annual salary = 3/4 of 7200 = 5400

∴ Anuj's annual salary = 5 × 5400 = Rs. 27000

RRB JE ECE (CBT I) Mock Test- 1 - Question 30
Save

The product of two successive numbers is 4032. Which is the greater of the two numbers?
Detailed Solution for RRB JE ECE (CBT I) Mock Test- 1 - Question 30

63 x 64 = 4032

View more questions
Information about RRB JE ECE (CBT I) Mock Test- 1 Page
In this test you can find the Exam questions for RRB JE ECE (CBT I) Mock Test- 1 solved & explained in the simplest way possible. Besides giving Questions and answers for RRB JE ECE (CBT I) Mock Test- 1, EduRev gives you an ample number of Online tests for practice

Top Courses for Electronics and Communication Engineering (ECE)

Download as PDF

Top Courses for Electronics and Communication Engineering (ECE)

Important Questions for RRB JE ECE (CBT I) Mock Test- 1

Find all the important questions for RRB JE ECE (CBT I) Mock Test- 1 at EduRev.Get fully prepared for RRB JE ECE (CBT I) Mock Test- 1 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the RRB JE ECE (CBT I) Mock Test- 1 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of RRB JE ECE (CBT I) Mock Test- 1 resources.

RRB JE ECE (CBT I) Mock Test- 1 MCQs with Answers

Prepare for the RRB JE ECE (CBT I) Mock Test- 1 within the Electronics and Communication Engineering (ECE) exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for RRB JE ECE (CBT I) Mock Test- 1

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace RRB JE ECE (CBT I) Mock Test- 1 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup