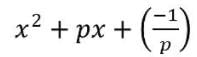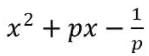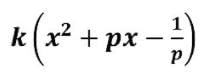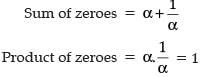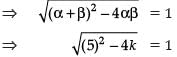Case Based Questions Test: Polynomials - 1 - Class 9 MCQ
15 Questions MCQ Test - Case Based Questions Test: Polynomials - 1
Direction: Read the following text and answer the following questions on the basis of the same:
The below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.
If the sum of the roots is –p and product of the roots is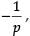 
then the quadratic polynomial is
then the quadratic polynomial is
Direction: Read the following text and answer the following questions on the basis of the same:
The below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.
If the roots of the quadratic polynomial are equal, where the discriminant D = b2 – 4ac, then
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Direction: Read the following text and answer the following questions on the basis of the same:
The below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.
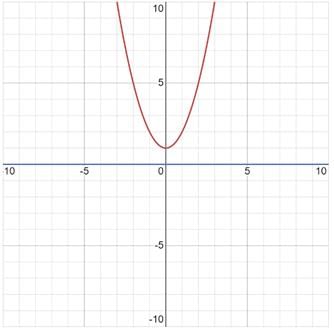
The graph of x2 + 1 = 0
Direction: Read the following text and answer the following questions on the basis of the same:
The below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.
In the standard form of quadratic polynomial, ax2 + bx, c, a, b and c are
Direction: Read the following text and answer the following questions on the basis of the same:
The below picture are few natural examples of parabolic shape which is represented by a quadratic polynomial. A parabolic arch is an arch in the shape of a parabola. In structures, their curve represents an efficient method of load, and so can be found in bridges and in architecture in a variety of forms.
If α are 1/α the zeroes of the quadratic polynomial 2x2 – x + 8k, then k is
Direction: Read the following text and answer the following questions on the basis of the same:
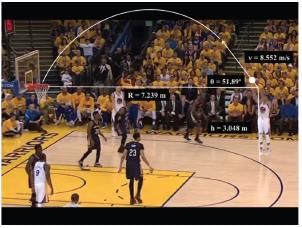
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.
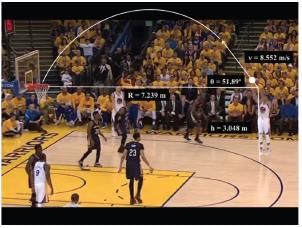
What will be the expression of the polynomial?
Direction: Read the following text and answer the following questions on the basis of the same:
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.
The graph of parabola opens upwards, if _______
Direction: Read the following text and answer the following questions on the basis of the same:
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.
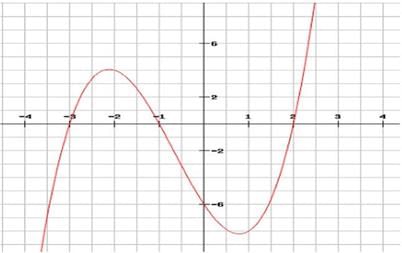
The three zeroes in the above shown graph are
Direction: Read the following text and answer the following questions on the basis of the same:
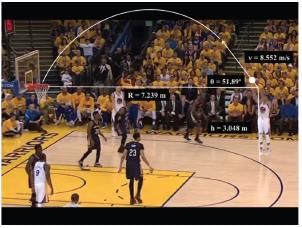
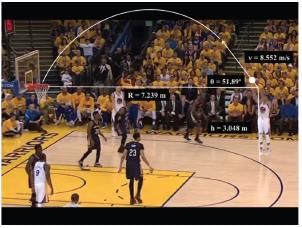
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.
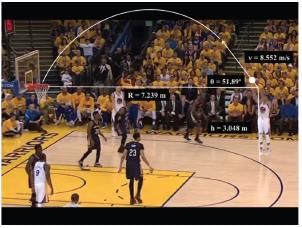
The shape of the path traced shown is
Direction: Read the following text and answer the following questions on the basis of the same:
Basketball and soccer are played with a spherical ball. Even though an athlete dribbles the ball in both sports, a basketball player uses his hands and a soccer player uses his feet. Usually, soccer is played outdoors on a large field and basketball is played indoor on a court made out of wood. The projectile (path traced) of soccer ball and basketball are in the form of parabola representing quadratic polynomial.
Observe the following graph and answer
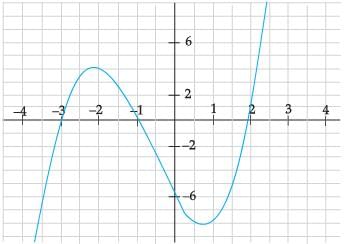
In the above graph, how many zeroes are there for the polynomial?
Direction: Read the following text and answer the following questions on the basis of the same:
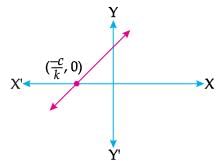
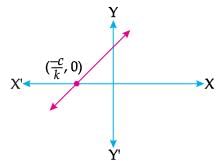
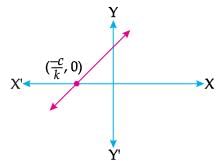
For a linear polynomial kx + c, k ≠ 0, the graph of y = kx + c is a straight line which intersects the X-axis at exactly one point, namely, 
Therefore, the linear polynomial kx + c, k ≠ 0, has exactly one zero, namely, the X-coordinate of the point where the graph of y = kx + c intersects the X-axis.
If the product of the zeroes of the quadratic polynomial p(x) = ax2 – 6x – 6 is 4, then the value of a is:
Direction: Read the following text and answer the following questions on the basis of the same:
For a linear polynomial kx + c, k ≠ 0, the graph of y = kx + c is a straight line which intersects the X-axis at exactly one point, namely,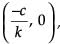 
Therefore, the linear polynomial kx + c, k ≠ 0, has exactly one zero, namely, the X-coordinate of the point where the graph of y = kx + c intersects the X-axis.
The graph of y = p(x) is given in figure below for some polynomial p(x). The number of zero/zeroes of p(x) is/are:
Direction: Read the following text and answer the following questions on the basis of the same:
For a linear polynomial kx + c, k ≠ 0, the graph of y = kx + c is a straight line which intersects the X-axis at exactly one point, namely, 
Therefore, the linear polynomial kx + c, k ≠ 0, has exactly one zero, namely, the X-coordinate of the point where the graph of y = kx + c intersects the X-axis.
If a and b are the zeroes of the quadratic polynomial p(x) = 4x2 + 5x + 1, then the product of zeroes is:
Direction: Read the following text and answer the following questions on the basis of the same:
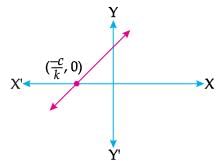
For a linear polynomial kx + c, k ≠ 0, the graph of y = kx + c is a straight line which intersects the X-axis at exactly one point, namely, 
Therefore, the linear polynomial kx + c, k ≠ 0, has exactly one zero, namely, the X-coordinate of the point where the graph of y = kx + c intersects the X-axis.
If a linear polynomial is 2x + 3, then the zero of 2x + 3 is:
Direction: Read the following text and answer the following questions on the basis of the same:
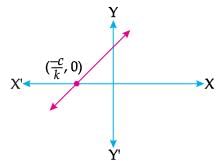
For a linear polynomial kx + c, k ≠ 0, the graph of y = kx + c is a straight line which intersects the X-axis at exactly one point, namely, 
Therefore, the linear polynomial kx + c, k ≠ 0, has exactly one zero, namely, the X-coordinate of the point where the graph of y = kx + c intersects the X-axis.
If α and β are the zeroes of the quadratic polynomial x2 – 5x + k such that α – β = 1, then the value of k is:
Top Courses for Class 9
Important Questions for Case Based Questions Test: Polynomials - 1
Case Based Questions Test: Polynomials - 1 MCQs with Answers
Online Tests for Case Based Questions Test: Polynomials - 1
|
© EduRev
|
Education Revolution
|
|
