Practice Test: Mechanical Engineering (ME)- 11 - Mechanical Engineering MCQ
30 Questions MCQ Test - Practice Test: Mechanical Engineering (ME)- 11
A man covered certain distance on foot at the rate of 5km/h and rest by bicycle at the rate of 12km/h. If he took 15hrs to cover a distance of 110km. Find the distance covered by him on foot.
The compound interest compounded half yearly on a certain sum of money at 10% per annum for 1 year is Rs. 246.The simple interest on the same sum for 2.5 years at 7% per annum is:
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If the world ‘MAJORITY’ is encoded as ‘PKBNXSHQ’, how will the word ‘DAUGHTER’ be encoded?
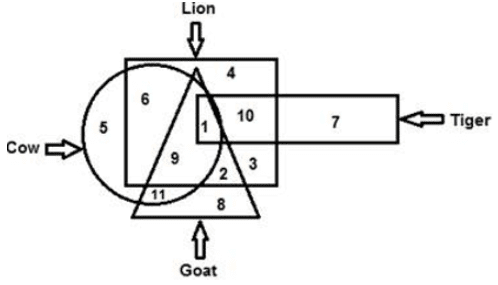
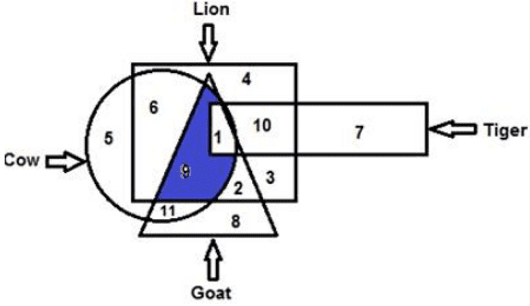
In the given figure, which number represents cow, lion and goat but not tiger.
In a car showroom, the average value of three cars is 15 Lakh, where the average price of two costliest cars is double the price of the cheapest car. Find the cost of the cheapest car.
In the following question, four words are given out of which one word is correctly spelt. Select the correctly spelt word.
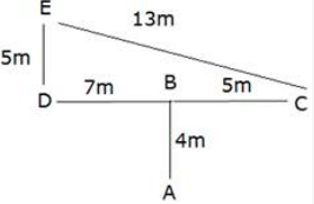
A is in the south of B at a distance of 4m. C is in the east of B at a distance of 5m. D is in the west of C at a distance of 12m. E is in the north of D at a distance of 5m. How far is C from E and E is in which direction from C?
In the sentence, identify the segment which contains the grammatical error.
Every student has to face a lot of pain to get selected in this competitive exam.
Arrange the given words in the sequence in which they occur in the dictionary.
1. Culmination, 2. Conclusion, 3. Climax, 4. Cultivation
Gustavo, Pablo and Gaviria are working together on a project. They can finish it together in a day. Gustavo alone can finish it in 2 days and Pablo in 3 days. How much time will Gaviria take to finish the project alone?
Let A ϵ M3 (R) be such that det (A-I) = 0, where I denotes the 3 x 3 identity matrix. If the trace of A = 13 and det A = 32, then the sum of squares of the eigen values of A is _________.
The value of surface integral 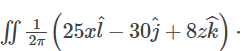 ndS over the surface of the sphere x2 + y2 + z2 = 16 is
ndS over the surface of the sphere x2 + y2 + z2 = 16 is
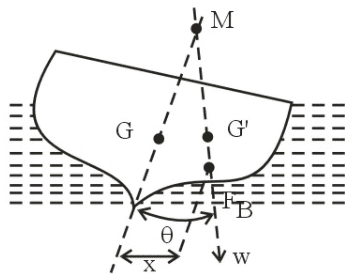
A cargo ship weighs 50 MN and the ships is tilted through an angle of 5 due to water of weighing 500 KN is being filled on ship’s boats. The mean distance of water (in mm) from the centre of the ships is 12 m. The metacentric height is
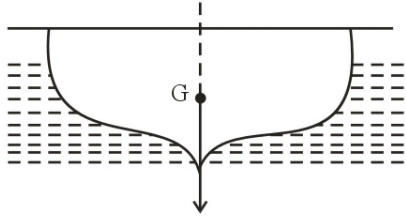
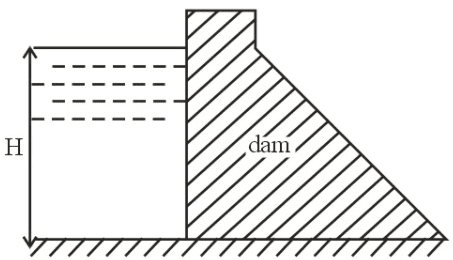
A dam is constructed across river for the purpose of irrigation. The depth of water (H) stored on the upstream side of dam is 5 m. The total pressure exerted on the upstream face for 1 m length of dam (in KN) is (assume g = 10 m/s2)
Three kg of oxygen gas is heated from 15oC to 90oC. , the change in enthalpy( in KJ) is
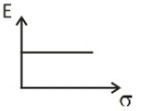
Which of the following curve represent a non-strain hardening material
A thin cylindrical pressure vessel of internal diameter 800 mm and wall thickness 10 mm is given a coating which cracks when the strain reaches to 0.0002. The internal pressure (in MPa) at which cracking start in coating is (Take E = 210 GPa, Poisson ratio = 0.25)
The effect of forced convection can be neglected if
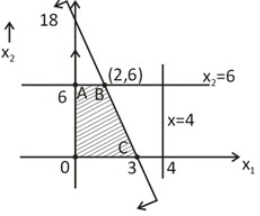
Consider the following linear programming problem
Maximize X= 3y1 + 2y2
Subject toy1 ≤ 4
y2 ≤ 6
6y1 + y2 ≤ 18
y1 ≥ 0, y2 ≥ 0
The optimal value of the objective function for the above problem is
In a single-channel queuing model, the customer arrival rate is 12 per hour and the waiting time in the queue is 2.5 minute. The proportion of time that a server actually spends with customers is
A rotating bar made of forged steel 50 mm in diameter (Sut = 600 N/m2), is subjected to completely reverse bending stress. The corrected endurance limit (in MPa) of the bar is 100 N/mm2. The fatigue strength of the bar for the life of 25000 cycles is
Which of the following is a multi point cutting tool?
Mechanism of material removal for ECM machining
A 6 mm thick sheet is rolled to 3 mm with a pair of rollers having diameter 300mm. The friction coefficient at the work roll interface is 0.1, the maximum number of passes required ____
A 140 mm diameter billet is extruded to 70 mm, at the working temperature of 800ºC. The average flow stress of material is 350 MPa, the force required for extrusion (in MN) is _____
The steam is expanded isentropically in steam turbine from 20 bars, 350∘C to 0.08 bars and then it condensed to saturated liquid water in the condenser. The pump feeds back the water into the boiler. Neglect losses in the processes, the cycle efficiency (in percentage) are
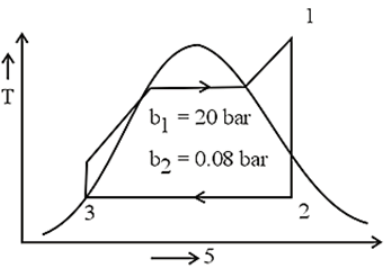
Given data: At p = 0.08 bar, hfg = 2403.1 kJ/kg, sfg = 7.6361 kJ/kg K, vf = 0.001008 m3/kg
h1 =3159.3 kJ/kg, s1 = 6.9917 kJ/kg K, h3 = 173.88 kJ/kg, s3 = 0.5926 kJ/kgK,
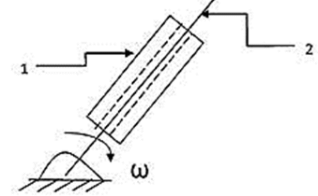
In the figure shown below, the relative velocity of link 1 with respect to link 2 is 15 m/s. The link 2 rotates at a constant speed of 150 rpm. The magnitude of the coriolis component of acceleration of link 1 is .....
The annual demand of the company is 10000 units per year. The ordering cost per order is Rs 100 per year and the carrying cost is Rs10 per unit per year and the shortage is allowed. The shortage cost is Rs 5 per unit per year. Maximum inventory is_______.
A cantilever beam of 5m length supports a triangularly distributed load over its entire length, the maximum of which is at the free end. The total load is 21 N. What is the bending moment at fixed end?


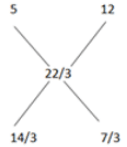
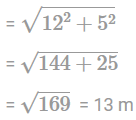
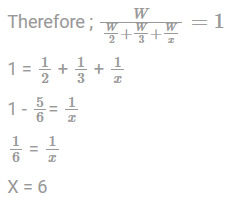
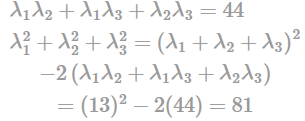
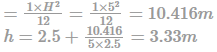
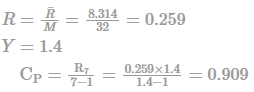
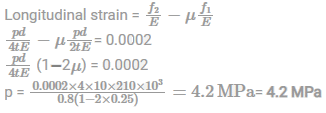
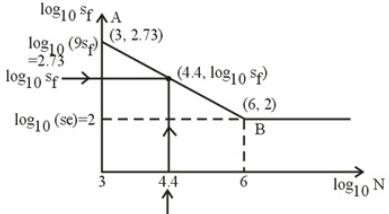
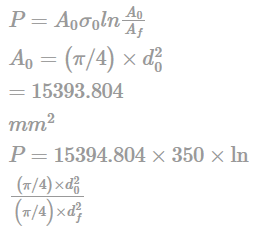
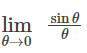 equal to?
equal to?