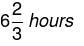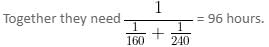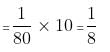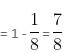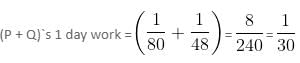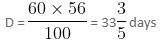Test Level 1: Time & Work - 1 - CAT MCQ
10 Questions MCQ Test - Test Level 1: Time & Work - 1
A and B can do a piece of work in 10 days and A alone can do it in 15 days. In how many days can B alone do it?
20 men can complete a work in 50 days, working for 5 hours a day. After 15 days from the start of work, 10 men quit. How many hours per day do the remaining men have to work so as to complete the work on time?
A contractor employs 200 men to build a dam. They finish 5/6 part of the work in 10 weeks. Due to rain, the work remains suspended for 4 weeks and also half of the work already done is washed away. When the work is resumed, only 140 men turn up. The total time in which the contractor is able to complete the work is (Assuming there are no further disruptions)
A contractor was asked to build a garage. He employed a number of men of equal efficiency. They could finish the job in 6 hours if they worked simultaneously, but he told them to begin one after the other, such that the intervals between their starting times should be equal. After the last worker had begun working, a time interval of the same length elapsed and the job was finished. Each one of the workers worked till the completion of the job. If the first man to begin worked 5 times as long as the last one to begin, in how many hours was the garage built?
An inlet pipe A lets water in a tank at 2 litres per minute and another pipe B lets water in at 3 litres per minute. There is an outlet pipe at the bottom, which can empty the tank in 10 hours. If all the three pipes were kept on, then the tank would be filled in 8 hours. What is the capacity of the tank?
Tap 'x' can fill the tank in 8 hours. Tap 'y' can empty it in 12 hours. Both the taps were opened for 4 hours and then, tap 'y' was closed. How much more time will it take to fill the tank completely?
Water flows from a pipe of sectional area 500 cm2 at the rate of 8 m/min. How long would it take to fill a tank whose dimensions are 6 m × 4 m × 4 m?
A and B, working 8 hours a day, can do a piece of work in 20 days and 30 days, respectively. On weekends, they work for only 1 hour and on weekdays, they work for 4 hours. If they start working together on Sunday, then on which day will they finish the task? (Assume that weekend includes Saturday and Sunday)
P can do a piece of work in 80 days. He works at it for 10 days and then Q alone finishes the remaining work in 42 days. The two together would have completed the work in
A group of 60 men can complete a piece of work in a certain number of days. If 10 more men join them, then the work can be completed in 8 days less. How many days will be required to complete the same work by 100 men?


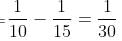

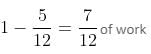
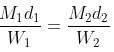
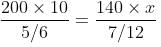
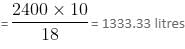
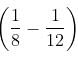 part = 1/24 part of the tank.
part = 1/24 part of the tank.