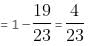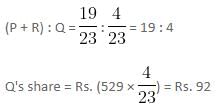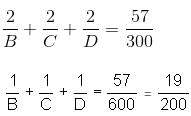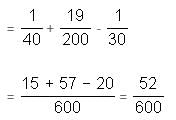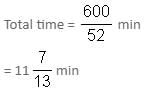Test Level 1: Time & Work - 2 - CAT MCQ
20 Questions MCQ Test - Test Level 1: Time & Work - 2
P, Q and R are employed to do a piece of work for Rs. 529. P and R are supposed to finish 19/23 of the work together. How much shall be paid to Q?
It was expected that 10 men together would complete a piece of work in 40 days. But after a few days, they increased their efficiency by 25% and completed the work six days earlier. How many days after the start of the work did they increase their efficiency?
A swimming pool is filled with water through four pipes, and there is an outlet pipe which can empty the pool in 30 minutes. The first pipe can fill the pool in 40 minutes; the second and third pipes can fill it in 15 minutes; the second and fourth pipes can fill it in 25 minutes; and the third and fourth pipes can fill it in 12 minutes.
In what time will the pool be filled if all the five pipes are working simultaneously?
Raju can do 25% of a piece of work in 5 days. How many days will he take to complete the work ten times?
A can do a piece of work in 20 days and B can do it in 15 days. How long will they take if both work together?
Nishu and Archana can do a piece of work in 10 days and Nishu alone can do it in 12 days. In how many days can Archana do it alone?
4 men and 3 women finish a job in 6 days, and 5 men and 7 women can do the same job in 4 days. How long will 1 man and 1 woman take to do the work?
A can do a piece of work in 10 days and B can do the same work in 20 days. With the help of C, they finish the work in 5 days. How long will it take for C alone to finish the work?
A and B undertake to do a piece of work for ₹ 100. A can do it in 5 days and B can do it in 10 days. With the help of C, they finish it in 2 days. How much should C be paid for his contribution?
Arun and Vinay together can do a piece of work in 7 days. If Arun does twice as much work as Vinay in a given time, how long will Arun alone take to do the work.
X number of men can finish a piece of work in 30 days. If there were 6 men more, the work could be finished in 10 days less. What is the original number of men?
Raju can do a piece of work in 10 days, Vicky in 12 days and Tinku in 15 days. They all start the work together, but Raju leaves after 2 days and Vicky leaves 3 days before the work is completed. In how many days is the work completed?
Manoj takes twice as much time as Anjay and thrice as much as Vijay to finish a piece of work. Together they finish the work in 1 day. What is the time taken by Manoj to finish the work?
Apurva can do a piece of work in 12 days. Apurva and Amit complete the work together and were paid ₹ 54 and ₹ 81 respectively. How many days must they have taken to complete the work together?
In a company XYZ Ltd. a certain number of engineers can develop a design in 40 days. If there were 5 more engineers, it could be finished in 10 days less. How many engineers were there in the beginning?
A can do a work in 10 days and B can do the same work in 20 days. They work together for 5 days and then A goes away. In how many more days will B finish the work?
Ajay and Vijay undertake to do a piece of work for ₹ 200. Ajay alone can do it in 24 days while Vijay alone can do it in 30 days. With the help of Pradeep, they finish the work in 12 days. How much should Pradeep get for his work?
Ajay, Vijay and Sanjay are employed to do a piece of work for ₹ 529. Ajay and Vijay together are supposed to do 19/23 of the work and Vijay and Sanjay together 8/23 of the work. How much should Ajay be paid?
In a fort there was sufficient food for 200 soldiers for 31 days. After 27 days 120 soldiers left the fort. For how many extra days will the rest of the food last for the remaining soldiers?
Ajay and Vijay can do a piece of work in 28 days. With the help of Manoj, they can finish it in 21 days. How long will Manoj take to finish the work all alone?