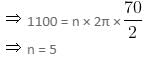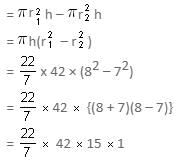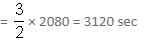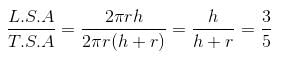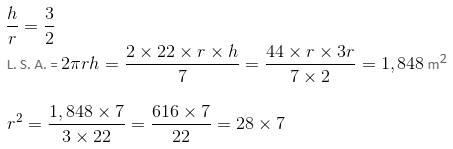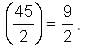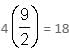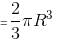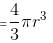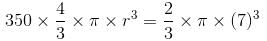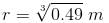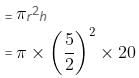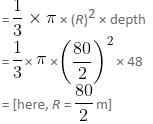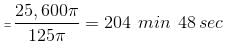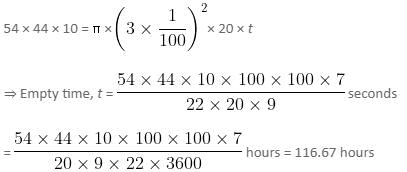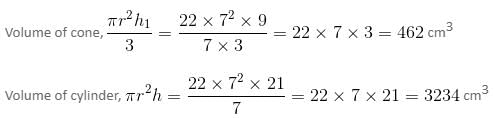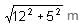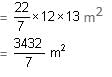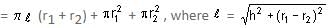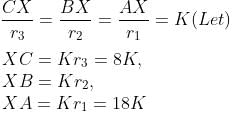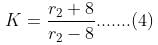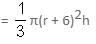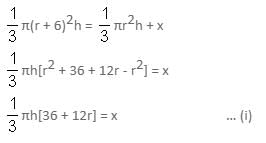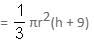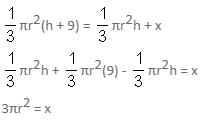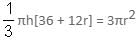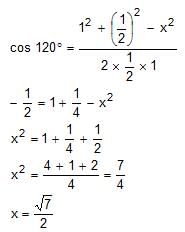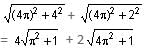Test Level 2: Mensuration - 2 - CAT MCQ
20 Questions MCQ Test - Test Level 2: Mensuration - 2
In the given diagram, a rope is wound around a circular drum whose diameter is 70 cm and a bucket is tied to the other end of the rope. Find the number of revolutions made by the drum if the bucket is to be raised by 11 m.
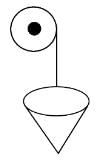

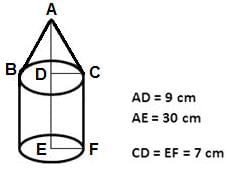
One of the base edges of a metallic plate, which is in the form of a right-angled triangle, is soldered to a wire. The wire is rotated around its own axis. What is the maximum volume of the shape obtained by rotating the metallic plate, given that the smallest side of the triangular plate is 8 cm in length and the largest side is 2 cm greater than the second largest side?
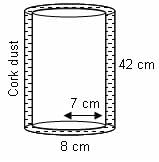
A cylindrical vessel of diameter 14 cm and height 42 cm is fixed symmetrically inside a similar vessel of diameter 16 cm and height 42 cm. If the total space between the two vessels is filled with cork dust for heat insulation purposes, then how many cubic centimetres of cork dust will be required?

A tap drips at an average rate of 2 drops in 3 seconds. It takes 2080 drops of water to completely fill a hemispherical vessel of radius 8.3 cm. How many times can the vessel be filled in this manner in 13 hours?
Ratio between the lateral surface area and the total surface area of a right circular cylinder is 3 : 5. If the lateral surface area is 1848 sq. m, then volume of the cylinder is (in cubic m).
The given rectangle ABCD has length 9 and width 5. Diagonal AC is divided into 5 equal parts at W, X, Y and Z. Find the area of the shaded region.

If 350 stones of spherical shape are dropped into a hemispherical cup containing some water, they get fully submerged. Diameter of the cup is 14 m. What will be the radius of the stone, if the water just spills off from the hemispherical cup?
Find the approximate time taken to fill a chemical conical vessel, if the flow rate is 20 meters per minute from a cylindrical pipe whose diameter is 5 meters. Take diameter and depth of the conical vessel as 80 meters and 48 meters, respectively.
A rectangular reservoir has dimensions 54 meters × 44 meters × 10 meters. An outlet pipe of circular cross-section has a radius of 3 centimeters and the water runs through the pipe at a rate of 20 meters/second. What is the time taken by the outlet pipe to empty the reservoir full of water?
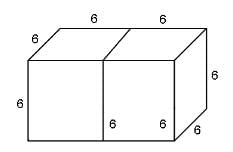
Two cubes, each of edge length 6 cm, are joined end to end. The surface area of the resulting cuboid is
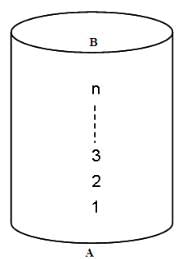
Consider a cylinder of height h cm and radius r = 2/π cm, as shown in the figure (not drawn to scale). A string of a certain length, when bound on its cylindrical surface starting at point A and ending at point B, gives a maximum of n turns (in other words, the string's length is the minimum length required to wind n turns). What is the vertical spacing in cm between the two consecutive turns?
A solid is in the form of a cylinder with hemispherical ends. The total height of the solid is 19 cm and the diameter of the cylinder is 7 cm. The surface area of the solid is
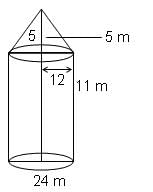
A tent is in the form of a right circular cylinder surmounted by a cone. The diameter of the cylinder is 24 m. The height of the cylindrical portion is 11 m, while the vertex of the cone is 16 m above the ground. What is the area of the curved surface for the conical portion?
Let ℓ be the slant height of a frustum. If a and b represent the two base radii, which of the following expressions represents the ratio of the total surface area to the curved surface area of the frustum?
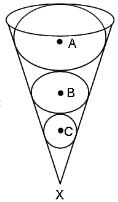
Three spherical marbles are placed in a hollow inverted cone, one above the other. Each marble is in contact with the adjacent marble(s). Also, each marble is in contact with the wall of the cone. The smallest marble has a radius of 8 cm. The largest marble has a radius of 18 cm.
The radius of the middle marble is
Increasing the height of a cone by 9 units increases its volume by x cubic units. Increasing its radius by 6 units also increases its volume by x cubic units. If the original height is 3 units, then the original radius is _____.
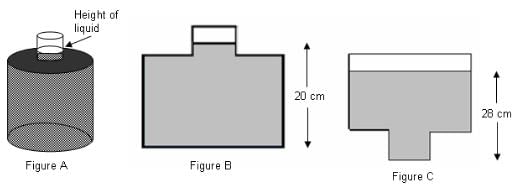
A sealed bottle, which contains water, has been constructed by attaching a cylinder of radius 1 cm to a cylinder of radius 3 cm, as shown in Figure A. When the bottle is upright, the height of the water is 20 cm, as shown in the cross section of the bottle in Figure B. When the bottle is upside down, the height of the liquid is 28 cm, as shown in Figure C. What is the total height (in cm) of the bottle?
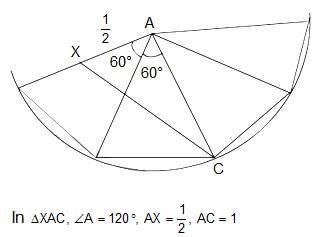
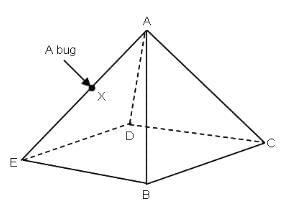
A certain pyramid has a square base with side 1 cm and four lateral faces, each of which is an equilateral triangle. A bug sitting at point X wishes to crawl over the faces of the pyramid to reach point C. Assuming that point X is half way up the pyramid, what is the length of the shortest trip he can take?
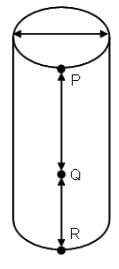
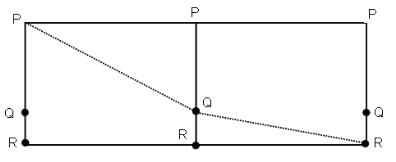
A cylindrical tank is 6 feet tall and its base is 4 feet in diameter. A bug crawls from a point P on the upper rim of the tank to a point Q which is directly 4 feet below P, going around the tank once in doing so. Then, the bug crawls from point Q to a point R, directly below P, on the bottom rim of the tank (the vertical distance from Q to R is 2 feet), again going around the can once in doing so. What is the length (in inches) of the shortest path that the bug could have taken from P to R?