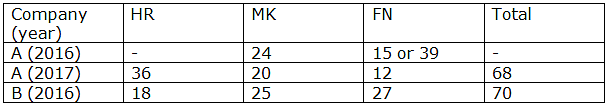All Courses
Get the App Signup / Login
Sign in Open App
Bank Exams Exam  >  Bank Exams Tests  >  Test: Quantitative Aptitude - 1 - Bank Exams MCQ

Test: Quantitative Aptitude - 1 - Bank Exams MCQ


Test Description

20 Questions MCQ Test - Test: Quantitative Aptitude - 1

Test: Quantitative Aptitude - 1 for Bank Exams 2024 is part of Bank Exams preparation. The Test: Quantitative Aptitude - 1 questions and answers have been prepared according to the Bank Exams exam syllabus.The Test: Quantitative Aptitude - 1 MCQs are made for Bank Exams 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Quantitative Aptitude - 1 below.
Solutions of Test: Quantitative Aptitude - 1 questions in English are available as part of our course for Bank Exams & Test: Quantitative Aptitude - 1 solutions in Hindi for Bank Exams course. Download more important topics, notes, lectures and mock test series for Bank Exams Exam by signing up for free. Attempt Test: Quantitative Aptitude - 1 | 20 questions in 10 minutes | Mock test for Bank Exams preparation | Free important questions MCQ to study for Bank Exams Exam | Download free PDF with solutions
Test: Quantitative Aptitude - 1 - Question 1
Save

Directioons: In each of the following question three or more statements is given. You are expected to decide which of the following statement or pair of statement is sufficient to answer the question.

Q. 6 years ago, age of father of Roma 14 years more than her only one brother and only one sister. Find the age of Sister of Roma after 12 years?

Statement I. Roma is 20 years younger than her father and her present age of 116.66% of her brother.

Statement II. 4 years ago, age of brother is 166.66% of Sister

Statement III.  8 years hence, Age of Father is same as sum of ages of her brother and Sister.

Detailed Solution for Test: Quantitative Aptitude - 1 - Question 1

Father – 6 = Sister – 6 + Brother – 6 + 14

Father = sister + brother + 8…. (1)

Statement I.

Farther = Roma + 20

Roma = 7/6 x brother

This statement alone is not sufficient to answer

Statement II.

Brother – 4 = 5/3 x (Sister - 4)

3 x brother – 12 = 5 x sister – 20

3 x brother – 5 x sister = - 8……….. (2)

This statement alone is not sufficient to answer the question

Statement III.

Father – 8 = Sister – 8 + Brother – 8

Father = brother + Sister – 8….. (3)

On combining I and II

Father = sister + brother + 8 ……. (1)

Also, Father = Roma + 20

Roma = 7/6 x brother

So, 7/6 x brother + 20= brother + sister + 8

6x sister – Brother = 72…………… (4)

Also, 3 x brother – 5 x sister = - 8 …..(2)

On solving 4 and 2, we get

Sister = 16 years

Age of sister after 12 years = 16 + 12 = 28 years

This combination is sufficient to answer the question.

Statement III have same information what we have in question initially.
So, combination of statement III with any other statement is not sufficient.

So only (I and II) is sufficient to answer the question.

Hence answer is option A

Test: Quantitative Aptitude - 1 - Question 2
Save

Read the following information carefully and answer the questions based on it.

Article P sold at 35% profit and Q at 20% profit, MRP of P is 92% more that of Q. Selling price of Q is 58.33% less than that of P. Article P sold after discount of (M – 15) % and Q at discount of (N +20) %. N is half of M.

Q. A retailer cheats whole seller, buys M% extra quantity and, cheats customer by giving N% less quantity. He claims no profit no loss to customer, who don’t know about cheating, fin d his actual profit %.

Detailed Solution for Test: Quantitative Aptitude - 1 - Question 2

Let MRP of Q = 100a

So MRP of P = 192% x 100a = 192a

Now,

192a x [ 100 – (M – 15)] % / 100a x [100 – (N + 20)] % = 12/5

Also, M = 2N

460 – 8N = 400 – 5N

3N = 60

Value of N = 20

Value of M = 2 x 20 = 40

Now,

CP of P X 1.35 / CP of Q x 1.2 = 12/5

CP of P / CP of Q = 32/15

Value of (2M + 3N) = 2 x 40 + 3 x 20 = 140

Let actual CP of 1 unit = Rs. 100

Cost price for retailor per unit = 500/7

Selling price for retailer per unit = 100/0.8 = Rs. 125

Ratio of CP and SP for retailor = 500/7: 125 = 4:7

Actual profit % = (7 – 4)/4 x 100 = 75%

Hence answer is option D

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Quantitative Aptitude - 1 - Question 3
Save

Quantity I. A and B entered into a partnership with initial investments of Rs. 3200 and Rs. 5000 respectively. After 6 months A increased his investment by 60% while B increased his investment by 40%. At the end of 1.5 years, difference in profit of A and B is Rs. 3336 then find the total profit earned by A and B together.

Quantity II. If difference between total surface area and curved surface area of cylinder is 7776 cm2. Find 30% of volume of cylinder, if height is 20 cm less than radius. Only numerical value.

Detailed Solution for Test: Quantitative Aptitude - 1 - Question 3

Quantity I.

According to given statement

Ratio of profit share of A and B = (3200 x 6 + 3200 x 1.6 x 12): (5000 x 6 + 5000 x 1.4 x 12) = 336:475

Required total profit = [3336/ (475 – 336)] x [475 + 336] = Rs. 19464

Quantity II.

According to question,

(2Πr2 + 2πrh – 2πrh) = 7776

So, radius of cylinder = [7776/ (2 x 3)] 1/2 = 36 cm

Height of cylinder = 36 – 20 = 16 cm

Required value = 30% x (3 x 36 x 36 x 16) = 18662.4 cm3

Quantity I > Quantity II

Hence answer is option A

Test: Quantitative Aptitude - 1 - Question 4
Save

Directions: Read the following information carefully and answer the questions based on it.

Article P sold at 35% profit and Q at 20% profit, MRP of P is 92% more that of Q. Selling price of Q is 58.33% less than that of P. Article P sold after discount of (M – 15) % and Q at discount of (N +20) %. N is half of M.

Q. If cost price of both articles together is Rs. 3760, then which of the following can be the possible value of (2M + 3N).

I. 87.5% of difference between cost price of P and twice of cost price of Q

II. 148 less than HCF of (Selling price of P, selling price of Q)

III. Rs. 20 more than profit earned on article R, whose cost price is same as that of Q, and having same markup %
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 4

Let MRP of Q = 100a

So MRP of P = 192% x 100a = 192a

Now,

192a x [ 100 – (M – 15)] % / 100a x [100 – (N + 20)] % = 12/5

Also, M = 2N

460 – 8N = 400 – 5N

3N = 60

Value of N = 20

Value of M = 2 x 20 = 40

Now,

CP of P X 1.35 / CP of Q x 1.2 = 12/5

CP of P / CP of Q = 32/15

Value of (2M + 3N) = 2 x 40 + 3 x 20 = 140

According to question,

So, CP of P = 32/47 X 3760 = 2560

CP of Q = 3760 – 2560 = 1200

SP of P = 2560 x 1.35 = Rs. 3456

SP of Q = 1200 x 1.2 = Rs. 1440

I. 87.5% of difference between cost price of P and twice of cost price of Q

7/8 x (2560 – 2 x 1200) = Rs. 140

This statement is true.

II. 44 more than HCF of (Selling price of P, selling price of Q)

SP of P = 3456 = 27 x 33

SP of Q = 1440 = 25 x 32 x 5

So HCF = 32 x 9 = 288

Required value = 288 – 148 = 140

This statement is true.

III. Rs. 20 more than profit earned on article R, sold at 10% profit whose cost price is same as that of Q, and having same markup %.

Profit earned on R = 1200 x 10% = 120

Required value = 120 + 20 = 140

All statements are true,

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 5
Save

P, Q, and invested in a business. Initial investment of P is 66.66% as that of Q, while initial investment of R is 275% more than that of P. P and Q started together and after 8 months P invested 50% more to his investment, Q invested 66.66% more to his investment and R joined them. If initial investment of Q is Rs. 12000, and at the end of year total profit is 1.7 lakhs. They donated 25% of profit for education of children.

M = Difference between profit share of P and Q

N = (2500 + difference between profit share of Q and R)

Q. Find which of the following option is true.
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 5

Ratio of initial investment of P and Q = 2:3

Ratio of initial investment of P and R = 4:15

Ratio of initial investment of P, Q, and R = 4:6:15

Ratio of profit share = (4 x 2 + 1.5 x 4 x 1): (6 x 2 + 5/3 x 6 x 1): (15 x 1)

= 14:22:15

Profit share of P = [14/ (14 + 22 + 15)] x 75% x 1.7 lakhs = Rs. 35000

Profit share of Q = 22/14 x 35000 = Rs. 55000

Profit share of R = 15/14 x 35000 = Rs. 37500

Value of M = 55000 – 35000 = Rs. 20000

Value of N = 2500 + 55000 – 37500 = Rs. 20000

Hence answer is option C

Test: Quantitative Aptitude - 1 - Question 6
Save

Directions: Read the following information carefully and answer the questions based on it.

There are two companies A and B. Each company have three departments – HR, Marketing (MK) and Finance (FN).

  • Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).
  • Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year. Employees in MK of company A (2017) were (3c – a/5), while employees in FN of company A (2017) were 2(a + 1).
  • Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year, while difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company A (2017)
  • a, b, c are positive integers. Where ‘a’ is prime number more than 3, while ‘b’ is an odd composite number more than 4. Sum of a and b is less than 20, and c is 50% of sum of a and b.
  • In company A (2016), number of employees is finance is less than employees in other two department in same year and same company.

Q. Find Minimum possible number of employees in company A in 2016?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 6

First, we need to find the value of a, b, c

Value of a is prime number more than 3. Value of b is odd composite number more than 4.

(a + b) < 20, that means both are less than 20

Value of b = 9, 15

Value of a = 5, 7, 11

If we add, 15 to any value of a we get sum more than 20 or 20. So this is for sure value of b = 9

Value of a = 5 or 7

Also,

Employees in MK of company A (2017) were (3c – a/5).

Value of a, must be divisible by 5.

So, value of a = 5

Value of c = (9 + 5)/2 = 7

Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).

Employees in MK of company A (2016) = 2 x (5 + 7) = 24

Employees in HR of company B (2016) = 24 – 6 = 18

Employees in MK of company B (2016) = 18/72 x 100 = 25

Employees in FN of company A (2017) = 2(a + 1) = 2 x (5 + 1) = 12

Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year.

Employees in HR of company A (2017) = 24 + 12 = 36

Employees in MK of company A (2017) = (3c – a/5) = (3 x 7 –5/5) = 20

Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year.

Employees in FN of company B (2016) = 18 x 1.5 = 27

Difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company (A).

So, number of employees in FN of company A (2016) = (27 – 12) or 27 + 12 = 39 or 15

According to question,

We need to find minimum possible number of employees of company A in 2016.

Number of employees in MK of company A in 2016 = 24

Minimum number of employees in FN of company A in 2016 = 15

Minimum number of employees in HR of company A in 2016 = 15 + 1 = 16

Required number of employees = 15 + 16 + 24 = 55

Hence answer is option C

Test: Quantitative Aptitude - 1 - Question 7
Save

Directions: Read the following information carefully and answer the questions based on it.

There are two companies A and B. Each company have three departments – HR, Marketing (MK) and Finance (FN).

  • Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).
  • Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year. Employees in MK of company A (2017) were (3c – a/5), while employees in FN of company A (2017) were 2(a + 1).
  • Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year, while difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company A (2017)
  • a, b, c are positive integers. Where ‘a’ is prime number more than 3, while ‘b’ is an odd composite number more than 4. Sum of a and b is less than 20, and c is 50% of sum of a and b.
  • In company A (2016), number of employees is finance is less than employees in other two department in same year and same company.

Q. If number of employees in FN of company A in 2016 is more than 15, then find minimum possible difference between number of employees in company A in 2016 and in 2017?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 7

First, we need to find the value of a, b, c

Value of a is prime number more than 3. Value of b is odd composite number more than 4.

(a + b) < 20, that means both are less than 20

Value of b = 9, 15

Value of a = 5, 7, 11

If we add, 15 to any value of a we get sum more than 20 or 20. So this is for sure value of b = 9

Value of a = 5 or 7

Also,

Employees in MK of company A (2017) were (3c – a/5).

Value of a, must be divisible by 5.

So, value of a = 5

Value of c = (9 + 5)/2 = 7

Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).

Employees in MK of company A (2016) = 2 x (5 + 7) = 24

Employees in HR of company B (2016) = 24 – 6 = 18

Employees in MK of company B (2016) = 18/72 x 100 = 25

Employees in FN of company A (2017) = 2(a + 1) = 2 x (5 + 1) = 12

Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year.

Employees in HR of company A (2017) = 24 + 12 = 36

Employees in MK of company A (2017) = (3c – a/5) = (3 x 7 –5/5) = 20

Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year.

Employees in FN of company B (2016) = 18 x 1.5 = 27

Difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company (A).

So, number of employees in FN of company A (2016) = (27 – 12) or 27 + 12 = 39 or 15

Number of employees in FN of company A in 2016 is more than 15

So, Number of employees in FN of company A in 2016 = 39

We need minimum possible difference.

So, employees in HR of company A in 2016 = 39 + 1 = 40

Required difference = (40 + 24 + 39) – 68 = 35

Hence answer is option A

Test: Quantitative Aptitude - 1 - Question 8
Save

Directions: Read the following information carefully and answer the questions based on it.

There are two companies A and B. Each company have three departments – HR, Marketing (MK) and Finance (FN).

  • Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).
  • Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year. Employees in MK of company A (2017) were (3c – a/5), while employees in FN of company A (2017) were 2(a + 1).
  • Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year, while difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company A (2017)
  • a, b, c are positive integers. Where ‘a’ is prime number more than 3, while ‘b’ is an odd composite number more than 4. Sum of a and b is less than 20, and c is 50% of sum of a and b.
  • In company A (2016), number of employees is finance is less than employees in other two department in same year and same company.

Q. Find minimum possible difference between number of employees in FN of company A and B in 2016?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 8

First, we need to find the value of a, b, c

Value of a is prime number more than 3. Value of b is odd composite number more than 4.

(a + b) < 20, that means both are less than 20

Value of b = 9, 15

Value of a = 5, 7, 11

If we add, 15 to any value of a we get sum more than 20 or 20. So this is for sure value of b = 9

Value of a = 5 or 7

Also,

Employees in MK of company A (2017) were (3c – a/5).

Value of a, must be divisible by 5.

So, value of a = 5

Value of c = (9 + 5)/2 = 7

Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).

Employees in MK of company A (2016) = 2 x (5 + 7) = 24

Employees in HR of company B (2016) = 24 – 6 = 18

Employees in MK of company B (2016) = 18/72 x 100 = 25

Employees in FN of company A (2017) = 2(a + 1) = 2 x (5 + 1) = 12

Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year.

Employees in HR of company A (2017) = 24 + 12 = 36

Employees in MK of company A (2017) = (3c – a/5) = (3 x 7 –5/5) = 20

Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year.

Employees in FN of company B (2016) = 18 x 1.5 = 27

Difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company (A).

So, number of employees in FN of company A (2016) = (27 – 12) or 27 + 12 = 39 or 15

Number of employees in FN of company A in 2016= 39 or 15

Number of employees in FN of company B in 2016 = 27

Required minimum difference = 27 – 15 = 39 – 27 = 12

Hence answer is option B

Test: Quantitative Aptitude - 1 - Question 9
Save

Directions: Read the following information carefully and answer the questions based on it.

There are two companies A and B. Each company have three departments – HR, Marketing (MK) and Finance (FN).

  • Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).
  • Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year. Employees in MK of company A (2017) were (3c – a/5), while employees in FN of company A (2017) were 2(a + 1).
  • Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year, while difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company A (2017)
  • a, b, c are positive integers. Where ‘a’ is prime number more than 3, while ‘b’ is an odd composite number more than 4. Sum of a and b is less than 20, and c is 50% of sum of a and b.
  • In company A (2016), number of employees is finance is less than employees in other two department in same year and same company.

Q. Find total number of employees in company B in 2016?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 9

First, we need to find the value of a, b, c

Value of a is prime number more than 3. Value of b is odd composite number more than 4.

(a + b) < 20, that means both are less than 20

Value of b = 9, 15

Value of a = 5, 7, 11

If we add, 15 to any value of a we get sum more than 20 or 20. So this is for sure value of b = 9

Value of a = 5 or 7

Also,

Employees in MK of company A (2017) were (3c – a/5).

Value of a, must be divisible by 5.

So, value of a = 5

Value of c = (9 + 5)/2 = 7

Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).

Employees in MK of company A (2016) = 2 x (5 + 7) = 24

Employees in HR of company B (2016) = 24 – 6 = 18

Employees in MK of company B (2016) = 18/72 x 100 = 25

Employees in FN of company A (2017) = 2(a + 1) = 2 x (5 + 1) = 12

Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year.

Employees in HR of company A (2017) = 24 + 12 = 36

Employees in MK of company A (2017) = (3c – a/5) = (3 x 7 –5/5) = 20

Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year.

Employees in FN of company B (2016) = 18 x 1.5 = 27

Difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company (A).

So, number of employees in FN of company A (2016) = (27 – 12) or 27 + 12 = 39 or 15

Required number of employees = 70

Hence answer is option D

Test: Quantitative Aptitude - 1 - Question 10
Save

Directions: Read the following information carefully and answer the questions based on it.

There are two companies A and B. Each company have three departments – HR, Marketing (MK) and Finance (FN).

  • Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).
  • Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year. Employees in MK of company A (2017) were (3c – a/5), while employees in FN of company A (2017) were 2(a + 1).
  • Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year, while difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company A (2017)
  • a, b, c are positive integers. Where ‘a’ is prime number more than 3, while ‘b’ is an odd composite number more than 4. Sum of a and b is less than 20, and c is 50% of sum of a and b.
  • In company A (2016), number of employees is finance is less than employees in other two department in same year and same company.

Q. Which of the following can be the maximum possible sum of number of employees in 2016 in both companies together?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 10

First, we need to find the value of a, b, c

Value of a is prime number more than 3. Value of b is odd composite number more than 4.

(a + b) < 20, that means both are less than 20

Value of b = 9, 15

Value of a = 5, 7, 11

If we add, 15 to any value of a we get sum more than 20 or 20. So this is for sure value of b = 9

Value of a = 5 or 7

Also,

Employees in MK of company A (2017) were (3c – a/5).

Value of a, must be divisible by 5.

So, value of a = 5

Value of c = (9 + 5)/2 = 7

Employees in MK of company A (2016) were 2(a + c), which is 6 more than employees in HR of company B (2016), which in turn 28% less than employees in MK of company B (2016).

Employees in MK of company A (2016) = 2 x (5 + 7) = 24

Employees in HR of company B (2016) = 24 – 6 = 18

Employees in MK of company B (2016) = 18/72 x 100 = 25

Employees in FN of company A (2017) = 2(a + 1) = 2 x (5 + 1) = 12

Employees in HR of company A (2017) is 24 more than that of employees in FN of same company in same year.

Employees in HR of company A (2017) = 24 + 12 = 36

Employees in MK of company A (2017) = (3c – a/5) = (3 x 7 –5/5) = 20

Employees in FN of company B (2016) is 50% more than employees in HR of same company in same year.

Employees in FN of company B (2016) = 18 x 1.5 = 27

Difference between employees in FN of company A (2016) and B (2016) is same as number of employees in FN of company (A).

So, number of employees in FN of company A (2016) = (27 – 12) or 27 + 12 = 39 or 15

Number of employees in company B in 2016 = 70

Number of employees in company A in (MK + FN) in 2016 = 24 + 39 = 63

Minimum number of employees in company A in HR = 40

So, sum ≥ 70 + 63 + 40 ≥ 173

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 11
Save

Directions: Read the following information carefully and answer the questions based on it.

Equation 1. 6P2 – 19P – (M2 – 4) = 0, M is positive integer

Equation 2. 4(Z – 4Y)2 + 4Y2 + K2 – 4YK = 0

(-7/3) and K is the root of equation 1.

Q. Find M is approx. how much % more or less than K?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 11

6P2 – 19P – (M2 – 4) = 0

6 x (-7/3)2 – 19 x (-7/3) = (M2 – 4)

M2 = 77 + 4

Value of M = 9

So,

(-7/3) + K = 19/6

Value of K = 11/2

Equation 2.

4(Z – 4Y)2 + 4Y2 + K2 – 4YK = 0

(Z – 4Y)2 + Y2 + K2/4 – YK = 0

(Z – 4Y)2 + (Y – K/2)2 = 0

Z = 4Y, Y = K/2

Value of Y = 11/4

Z = 4 x 11/4 = 11

Value of M = 9

Value of K = 11/2

M: K = 9:11/2 = 18:11

Required % change = [(18 – 11)/11] x 100 = 63%

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 12
Save

Directions: In each of the following question three or more statements is given. You are expected to decide which of the following statement or pair of statement is sufficient to answer the question. Find the Value of D.

Q. A boatman covers a total distance of ‘2D + 160’ km daily. He started from his home daily at fixed time and go to his work place and after that come to home by same route. Speed of river is 4 km/h, and he used different boats on each day. Flow of river is from home to workplace.

Statement I. Speed of boat in still water on Monday is 50% more than that of Wednesday, which is 55.55% less than that on Tuesday.

Statement II. Total traveling time on Wednesday is 128/3 hours.

Statement III. Time taken for return journey on Tuesday is 12.5% less than forward journey on Monday.
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 12

On combining (I + III).

Ratio of speed of boat in still water on Monday, Tuesday and Wednesday together = 6:9:4

Now,

(9a – 4) / (6a + 4) = 8/7

63a – 28 = 48a + 32

Value of a = 4

We are able to find speeds but we don’t know about the time. We need time to calculate distance.

This combination is not sufficient to answer the question.

On combining (I and II)

Ratio of speed o boat in still water on Monday, Tuesday and Wednesday together = 6:9:4

Now,

(9a – 4) / (6a + 4) = 8/7

63a – 28 = 48a + 32

Value of a = 4

Now,

(D + 80) / (4a – 4) + (D + 80)/ (4a + 4) = 128/3

We have two variables, so can’t calculate distance

This combination is also not sufficient to answer the questions.

So, also on combining (II + III), we are not able to find the data.

On combing all three statements (I + II + III)

Ratio of speed of boat in still water on Monday, Tuesday and Wednesday together = 6:9:4

Now,

(9a – 4) / (6a + 4) = 8/7

63a – 28 = 48a + 32

Value of a = 4

So, (D + 80) / (4a – 4) + (D + 80)/ (4a + 4) = 128/3

(D + 80)/12 + (D + 80)/20 = 128/3

So, value of D = 240 km

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 13
Save

Directions: In each of the following question three or more statements is given. You are expected to decide which of the following statement or pair of statement is sufficient to answer the question.

Q. The LCM of two positive integers P and Q is 165. What will be the 50% of HCF of P and Q?

Statement I. 50% of (P – Q) is a multiple 3 less than 12

Statement II. 75% of (P + Q) = 36

Statement III. P2 – 55P + 726 = 0
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 13

On combining (I + II)

50% of (P – Q) = 3 or 6 or 9

(P – Q) = 6 or 12 or 18…………….. (1)

Also, 75% of (P + Q) = 36

(P + Q) = 48…………… (2)

On solving both equations we get

P = 27, Q = 21

P = 30, Q = 18

P = 33, Q = 15

LCM of (33, 15) is 165

33 = 11 x 3

15 = 5 x 3

So, HCF of (33, 15) = 3

Required value = 50% x 3 = 1.5

This combination of statement is sufficient to answer the question

On combining (II + III)

75% of (P + Q) = 36

(P + Q) = 48

Also,

P2 – 55P + 726 = 0

(P – 33)(P – 22) = 0

P = 33, 22

On putting these values in above equation

Q = 15, 26

LCM of (33, 15) = 165

33 = 11 x 3

15 = 5 x 3

So, HCF of (33, 15) = 3

Required value = 50% x 3 = 1.5

This combination is sufficient to answer the question.

On combining (III + I)

50% of (P – Q) = 3 or 6 or 9

(P – Q) = 6 or 12 or 18

Also,

P2 – 55P + 726 = 0

(P – 33)(P – 22) = 0

P = 33, 22

On putting these values in above equation

Q = 27, 16, 21, 9, 15, 4

Only (33, 15) gives LCM 165

This combination of statement is sufficient to answer the question.

So, combination of any two statement is sufficient to answer the question.

Hence answer is option D

Test: Quantitative Aptitude - 1 - Question 14
Save

24, 38, (2P), 98, 148, 214

(P – 11) is the first term of another sequence have same logic as the given sequence find 4th element of later sequence.
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 14

24 + (22 + 10) = 38

38 + (32 + 15) = 62

62 + (42 + 20) = 98

98 + (52 + 25) = 148

148 + (62 + 30) = 214

Value of P = 62/2 = 31

Fist term of other sequence = (31 – 11) = 20

20 + (22 + 10) = 34

34 + (32 + 15) = 58

58 + (42 + 20) = 94

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 15
Save

Directions: In each of the following question three or more statements is given. You are expected to decide which of the following statement or pair of statement is sufficient to answer the question.

Q. A water tank is cuboidal in shape having dimension (48 cm x 32 cm x 40cm). if there is a leakage in tank, so this leakage emptied the water at the rate of 64 cm3/ second. Two taps T1 and T2 together can fill the tank in 7.5 minutes. Find the time taken by tap T1 to fill the tank.

I. Time taken by tap T3 and T2 together to fill the tank is 10 minutes.

II. time taken by T2 to fill the tank with leakage is 48 minutes.

III. Time taken by T3 to fill the tank which is hemispherical in shape is 2 hours. Volume of hemispherical tank is twice as that of cuboidal tank
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 15

Total volume of cuboidal tank = 48 x 32 x 40 = 61440 cm3

Time taken by leakage to empty the tank alone = 61440 / (64 x 60) = 16 minutes.

We need to find connection between T1 and leakage.

From statement I, we have connection of T3 and T2 together. And in main statement we have connection of T1 and T2 together, so we are not able to find the alone time of T1.

From statement II,

Part of tank filled by T2 with leakage in 1 minute = 1/48

Part of tank filled by leakage to fill the tank alone = 1/16

So, time taken by T2 alone to fill the tank is = 1/ (1/16 +1/48) = 12 minutes

Time taken by T1 alone to fill the tank = 1/ (2/15 – 1/12) = 20 minutes

This statement alone is sufficient to answer the question.

From statement III, we don’t have any connection of T3 with the given taps of leakage in given statement. This statement alone is not sufficient.

On combining (I and III)

Time taken by T3 to fill the cuboidal tank = 120/2 = 60 minutes

Time taken by T2 alone to fill the tank = 1/ (1/10 – 1/60) = 12 minutes

So, time taken by T1 alone to fill the tank = 1/ (2/15 – 1/12) = 20 minutes

Either II alone or I and III together is sufficient to answer the question.

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 16
Save

Directions: Read the following information carefully and answer the questions based on it.

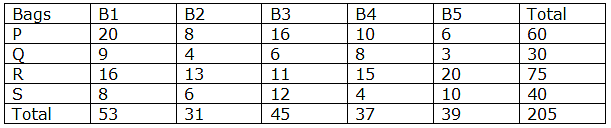
There are four bags – P, Q, R, and S. Each bag contains five type of balls – B1, B2, B3, B4, and B5.

Bag P: Number of B4 balls is 10, which is 2 more than B2. Number of B1 balls in bag P is 5 times of B2 balls in bag Q. Respective ratio of number of B3 and B5 balls is 8:3, and probability of picking a B2 ball from bag is 2/15.

Bag Q: number of B4 and B2 balls respectively is 8 and 4. Number of B1 balls is thrice of B5 balls and total balls in bag is 5 times of B5 balls in bag P. Difference between probability of picking a B1 balls and probability of picking a B5 ball is 1/5.

Bag R: Number of B3 balls is 11, which is 55% of number of B5 balls. Total balls in bag are35 more than bag S, and probability of picking two B4 balls from bag is 7/185. Number of B1 balls is 4 times of number of B4 balls in bag S.

Bag S: Number of B3 balls is 50% more than that of B1, while number of B5 balls are 10. Probability of picking a B2 ball from bag is 3/20, while number of B2 balls is 50% more than B4. Number of B3 balls are 12.

Q. Find probability of picking two B4 balls from bag S?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 16

For Bag P

Number of B4 balls = 10

Number of B2 balls = 10 – 2 = 8

Number of B1 balls = 5 x 4 = 20

probability of picking a B2 ball from bag is 2/15.

So, total balls in Bag = 15/2 x 8 = 60

Number of B3 and B5 balls = 60 – 10 – 8 – 20 = 22

Number of B3 balls = 8/11 x 22 = 16

Number of B5 balls = 22 – 16 = 6

For bag Q

Number of B4 balls = 8

Number of B2 balls = 4

Total balls in bag = 5 x 6 = 30

Let number of B5 balls = a

So, number of B1 balls = 3 x a = 3a

Now,

3a/30 – a/30 = 1/5

Value of a = 3

Number of B1 balls = 3 x 3 = 9

Number of B5 balls = 3

Number of B3 balls = 30 – 4 – 8 – 9 – 3 = 6

For Bag S

Number of B3 balls = 12

Number of B1 balls = 12/150 x 100 = 8

Let number of B4 balls = 2a

Number of B2 balls = 150% x 2a = 3a

Number of B5 balls = 10

Probability of picking a B2 ball from bag is 3/20

3a / (3a + 2a + 12 + 8 + 10) = 3/20

20a = 5a + 30

Value of a = 2

Total balls in bag = 30 + 6 + 4 = 40

For bag R

Total balls in bag = 40 + 35 = 75

Number of B1 balls = 4 x 4 = 16

Number of B3 balls = 11

Number of B5 balls = 11/55 x 100 = 20

probability of picking two B4 balls from bag is 7/185

let number of B4 balls = a

so,

a x (a – 1) / (75 x 74) = 7 / 185

a x (a – 1) = 210

so, value of a = 15

number of B4 balls in bag = 15

number of B2 balls in bag = 75 – 15 – 16 – 11 – 20 = 13

According to question,

Number of B4 balls in Bag S = 4

Required probability = (4 x 3) / (40 x 39) = 1/130

Hence answer is option D

Test: Quantitative Aptitude - 1 - Question 17
Save

Directions: Read the following information carefully and answer the questions based on it.

There are four bags – P, Q, R, and S. Each bag contains five type of balls – B1, B2, B3, B4, and B5.

Bag P: Number of B4 balls is 10, which is 2 more than B2. Number of B1 balls in bag P is 5 times of B2 balls in bag Q. Respective ratio of number of B3 and B5 balls is 8:3, and probability of picking a B2 ball from bag is 2/15.

Bag Q: number of B4 and B2 balls respectively is 8 and 4. Number of B1 balls is thrice of B5 balls and total balls in bag is 5 times of B5 balls in bag P. Difference between probability of picking a B1 balls and probability of picking a B5 ball is 1/5.

Bag R: Number of B3 balls is 11, which is 55% of number of B5 balls. Total balls in bag are35 more than bag S, and probability of picking two B4 balls from bag is 7/185. Number of B1 balls is 4 times of number of B4 balls in bag S.

Bag S: Number of B3 balls is 50% more than that of B1, while number of B5 balls are 10. Probability of picking a B2 ball from bag is 3/20, while number of B2 balls is 50% more than B4. Number of B3 balls are 12.

Q. Find the difference between probability of picking a B3 ball and a B5 ball from bag P?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 17

For Bag P

Number of B4 balls = 10

Number of B2 balls = 10 – 2 = 8

Number of B1 balls = 5 x 4 = 20

probability of picking a B2 ball from bag is 2/15.

So, total balls in Bag = 15/2 x 8 = 60

Number of B3 and B5 balls = 60 – 10 – 8 – 20 = 22

Number of B3 balls = 8/11 x 22 = 16

Number of B5 balls = 22 – 16 = 6

For bag Q

Number of B4 balls = 8

Number of B2 balls = 4

Total balls in bag = 5 x 6 = 30

Let number of B5 balls = a

So, number of B1 balls = 3 x a = 3a

Now,

3a/30 – a/30 = 1/5

Value of a = 3

Number of B1 balls = 3 x 3 = 9

Number of B5 balls = 3

Number of B3 balls = 30 – 4 – 8 – 9 – 3 = 6

For Bag S

Number of B3 balls = 12

Number of B1 balls = 12/150 x 100 = 8

Let number of B4 balls = 2a

Number of B2 balls = 150% x 2a = 3a

Number of B5 balls = 10

Probability of picking a B2 ball from bag is 3/20

3a / (3a + 2a + 12 + 8 + 10) = 3/20

20a = 5a + 30

Value of a = 2

Total balls in bag = 30 + 6 + 4 = 40

For bag R

Total balls in bag = 40 + 35 = 75

Number of B1 balls = 4 x 4 = 16

Number of B3 balls = 11

Number of B5 balls = 11/55 x 100 = 20

probability of picking two B4 balls from bag is 7/185

let number of B4 balls = a

so,

a x (a – 1) / (75 x 74) = 7 / 185

a x (a – 1) = 210

so, value of a = 15

number of B4 balls in bag = 15

number of B2 balls in bag = 75 – 15 – 16 – 11 – 20 = 13

Required probability

16/60 – 6/60 = 1/6

Hence answer is option B

Test: Quantitative Aptitude - 1 - Question 18
Save

Directions: Read the following information carefully and answer the questions based on it.

There are four bags – P, Q, R, and S. Each bag contains five type of balls – B1, B2, B3, B4, and B5.

Bag P: Number of B4 balls is 10, which is 2 more than B2. Number of B1 balls in bag P is 5 times of B2 balls in bag Q. Respective ratio of number of B3 and B5 balls is 8:3, and probability of picking a B2 ball from bag is 2/15.

Bag Q: number of B4 and B2 balls respectively is 8 and 4. Number of B1 balls is thrice of B5 balls and total balls in bag is 5 times of B5 balls in bag P. Difference between probability of picking a B1 balls and probability of picking a B5 ball is 1/5.

Bag R: Number of B3 balls is 11, which is 55% of number of B5 balls. Total balls in bag are35 more than bag S, and probability of picking two B4 balls from bag is 7/185. Number of B1 balls is 4 times of number of B4 balls in bag S.

Bag S: Number of B3 balls is 50% more than that of B1, while number of B5 balls are 10. Probability of picking a B2 ball from bag is 3/20, while number of B2 balls is 50% more than B4. Number of B3 balls are 12.

Q. Find total number of balls in all four bags together?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 18

For Bag P

Number of B4 balls = 10

Number of B2 balls = 10 – 2 = 8

Number of B1 balls = 5 x 4 = 20

probability of picking a B2 ball from bag is 2/15.

So, total balls in Bag = 15/2 x 8 = 60

Number of B3 and B5 balls = 60 – 10 – 8 – 20 = 22

Number of B3 balls = 8/11 x 22 = 16

Number of B5 balls = 22 – 16 = 6

For bag Q

Number of B4 balls = 8

Number of B2 balls = 4

Total balls in bag = 5 x 6 = 30

Let number of B5 balls = a

So, number of B1 balls = 3 x a = 3a

Now,

3a/30 – a/30 = 1/5

Value of a = 3

Number of B1 balls = 3 x 3 = 9

Number of B5 balls = 3

Number of B3 balls = 30 – 4 – 8 – 9 – 3 = 6

For Bag S

Number of B3 balls = 12

Number of B1 balls = 12/150 x 100 = 8

Let number of B4 balls = 2a

Number of B2 balls = 150% x 2a = 3a

Number of B5 balls = 10

Probability of picking a B2 ball from bag is 3/20

3a / (3a + 2a + 12 + 8 + 10) = 3/20

20a = 5a + 30

Value of a = 2

Total balls in bag = 30 + 6 + 4 = 40

For bag R

Total balls in bag = 40 + 35 = 75

Number of B1 balls = 4 x 4 = 16

Number of B3 balls = 11

Number of B5 balls = 11/55 x 100 = 20

probability of picking two B4 balls from bag is 7/185

let number of B4 balls = a

so,

a x (a – 1) / (75 x 74) = 7 / 185

a x (a – 1) = 210

so, value of a = 15

number of B4 balls in bag = 15

number of B2 balls in bag = 75 – 15 – 16 – 11 – 20 = 13

Required number of balls = 205

Hence answer is option E

Test: Quantitative Aptitude - 1 - Question 19
Save

Directions: Read the following information carefully and answer the questions based on it.

There are four bags – P, Q, R, and S. Each bag contains five type of balls – B1, B2, B3, B4, and B5.

Bag P: Number of B4 balls is 10, which is 2 more than B2. Number of B1 balls in bag P is 5 times of B2 balls in bag Q. Respective ratio of number of B3 and B5 balls is 8:3, and probability of picking a B2 ball from bag is 2/15.

Bag Q: number of B4 and B2 balls respectively is 8 and 4. Number of B1 balls is thrice of B5 balls and total balls in bag is 5 times of B5 balls in bag P. Difference between probability of picking a B1 balls and probability of picking a B5 ball is 1/5.

Bag R: Number of B3 balls is 11, which is 55% of number of B5 balls. Total balls in bag are35 more than bag S, and probability of picking two B4 balls from bag is 7/185. Number of B1 balls is 4 times of number of B4 balls in bag S.

Bag S: Number of B3 balls is 50% more than that of B1, while number of B5 balls are 10. Probability of picking a B2 ball from bag is 3/20, while number of B2 balls is 50% more than B4. Number of B3 balls are 12.

Q. Find number of B2 balls in bag R?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 19

For Bag P

Number of B4 balls = 10

Number of B2 balls = 10 – 2 = 8

Number of B1 balls = 5 x 4 = 20

probability of picking a B2 ball from bag is 2/15.

So, total balls in Bag = 15/2 x 8 = 60

Number of B3 and B5 balls = 60 – 10 – 8 – 20 = 22

Number of B3 balls = 8/11 x 22 = 16

Number of B5 balls = 22 – 16 = 6

For bag Q

Number of B4 balls = 8

Number of B2 balls = 4

Total balls in bag = 5 x 6 = 30

Let number of B5 balls = a

So, number of B1 balls = 3 x a = 3a

Now,

3a/30 – a/30 = 1/5

Value of a = 3

Number of B1 balls = 3 x 3 = 9

Number of B5 balls = 3

Number of B3 balls = 30 – 4 – 8 – 9 – 3 = 6

For Bag S

Number of B3 balls = 12

Number of B1 balls = 12/150 x 100 = 8

Let number of B4 balls = 2a

Number of B2 balls = 150% x 2a = 3a

Number of B5 balls = 10

Probability of picking a B2 ball from bag is 3/20

3a / (3a + 2a + 12 + 8 + 10) = 3/20

20a = 5a + 30

Value of a = 2

Total balls in bag = 30 + 6 + 4 = 40

For bag R

Total balls in bag = 40 + 35 = 75

Number of B1 balls = 4 x 4 = 16

Number of B3 balls = 11

Number of B5 balls = 11/55 x 100 = 20

probability of picking two B4 balls from bag is 7/185

let number of B4 balls = a

so,

a x (a – 1) / (75 x 74) = 7 / 185

a x (a – 1) = 210

so, value of a = 15

number of B4 balls in bag = 15

number of B2 balls in bag = 75 – 15 – 16 – 11 – 20 = 13

Number of B2 balls in bag R = 13

Hence answer is option C

Test: Quantitative Aptitude - 1 - Question 20
Save

Directions: Read the following information carefully and answer the questions based on it.

There are four bags – P, Q, R, and S. Each bag contains five type of balls – B1, B2, B3, B4, and B5.

Bag P: Number of B4 balls is 10, which is 2 more than B2. Number of B1 balls in bag P is 5 times of B2 balls in bag Q. Respective ratio of number of B3 and B5 balls is 8:3, and probability of picking a B2 ball from bag is 2/15.

Bag Q: number of B4 and B2 balls respectively is 8 and 4. Number of B1 balls is thrice of B5 balls and total balls in bag is 5 times of B5 balls in bag P. Difference between probability of picking a B1 balls and probability of picking a B5 ball is 1/5.

Bag R: Number of B3 balls is 11, which is 55% of number of B5 balls. Total balls in bag are35 more than bag S, and probability of picking two B4 balls from bag is 7/185. Number of B1 balls is 4 times of number of B4 balls in bag S.

Bag S: Number of B3 balls is 50% more than that of B1, while number of B5 balls are 10. Probability of picking a B2 ball from bag is 3/20, while number of B2 balls is 50% more than B4. Number of B3 balls are 12.

Q. Find the probability of picking two B3 balls from bag Q?
Detailed Solution for Test: Quantitative Aptitude - 1 - Question 20

For Bag P

Number of B4 balls = 10

Number of B2 balls = 10 – 2 = 8

Number of B1 balls = 5 x 4 = 20

probability of picking a B2 ball from bag is 2/15.

So, total balls in Bag = 15/2 x 8 = 60

Number of B3 and B5 balls = 60 – 10 – 8 – 20 = 22

Number of B3 balls = 8/11 x 22 = 16

Number of B5 balls = 22 – 16 = 6

For bag Q

Number of B4 balls = 8

Number of B2 balls = 4

Total balls in bag = 5 x 6 = 30

Let number of B5 balls = a

So, number of B1 balls = 3 x a = 3a

Now,

3a/30 – a/30 = 1/5

Value of a = 3

Number of B1 balls = 3 x 3 = 9

Number of B5 balls = 3

Number of B3 balls = 30 – 4 – 8 – 9 – 3 = 6

For Bag S

Number of B3 balls = 12

Number of B1 balls = 12/150 x 100 = 8

Let number of B4 balls = 2a

Number of B2 balls = 150% x 2a = 3a

Number of B5 balls = 10

Probability of picking a B2 ball from bag is 3/20

3a / (3a + 2a + 12 + 8 + 10) = 3/20

20a = 5a + 30

Value of a = 2

Total balls in bag = 30 + 6 + 4 = 40

For bag R

Total balls in bag = 40 + 35 = 75

Number of B1 balls = 4 x 4 = 16

Number of B3 balls = 11

Number of B5 balls = 11/55 x 100 = 20

probability of picking two B4 balls from bag is 7/185

let number of B4 balls = a

so,

a x (a – 1) / (75 x 74) = 7 / 185

a x (a – 1) = 210

so, value of a = 15

number of B4 balls in bag = 15

number of B2 balls in bag = 75 – 15 – 16 – 11 – 20 = 13

For Bag Q

Number of B3 balls = 6

Total number of balls = 30

Required probability = (6 x 5) / (30 x 29) = 1/29

Hence answer is option A

Information about Test: Quantitative Aptitude - 1 Page
In this test you can find the Exam questions for Test: Quantitative Aptitude - 1 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Quantitative Aptitude - 1, EduRev gives you an ample number of Online tests for practice

Top Courses for Bank Exams

Download as PDF

Top Courses for Bank Exams

Important Questions for Quantitative Aptitude - 1

Find all the important questions for Quantitative Aptitude - 1 at EduRev.Get fully prepared for Quantitative Aptitude - 1 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Quantitative Aptitude - 1 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Quantitative Aptitude - 1 resources.

Quantitative Aptitude - 1 MCQs with Answers

Prepare for the Quantitative Aptitude - 1 within the Bank Exams exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Quantitative Aptitude - 1

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Quantitative Aptitude - 1 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup