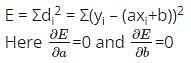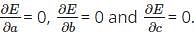Civil Engineering (CE) Exam > Civil Engineering (CE) Tests > Test: Approximation of Functions using Least Square Method - Civil Engineering (CE) MCQ
Test: Approximation of Functions using Least Square Method - Civil Engineering (CE) MCQ
Test Description
10 Questions MCQ Test - Test: Approximation of Functions using Least Square Method
Test: Approximation of Functions using Least Square Method for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Test: Approximation of Functions using Least Square Method questions and answers have been prepared
according to the Civil Engineering (CE) exam syllabus.The Test: Approximation of Functions using Least Square Method MCQs are made for Civil Engineering (CE) 2024 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Approximation of Functions using Least Square Method below.
Solutions of Test: Approximation of Functions using Least Square Method questions in English are available as part of our course for Civil Engineering (CE) & Test: Approximation of Functions using Least Square Method solutions in
Hindi for Civil Engineering (CE) course.
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free. Attempt Test: Approximation of Functions using Least Square Method | 10 questions in 30 minutes | Mock test for Civil Engineering (CE) preparation | Free important questions MCQ to study for Civil Engineering (CE) Exam | Download free PDF with solutions
Test: Approximation of Functions using Least Square Method - Question 1
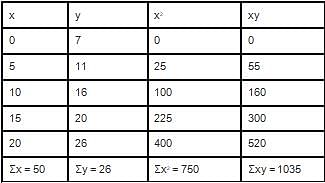
Fit the straight line to the following data.
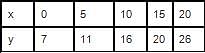

Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 1
Test: Approximation of Functions using Least Square Method - Question 2
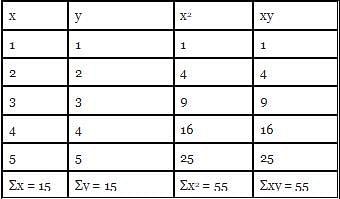
Fit the straight line to the following data.
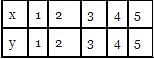

Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Test: Approximation of Functions using Least Square Method - Question 3
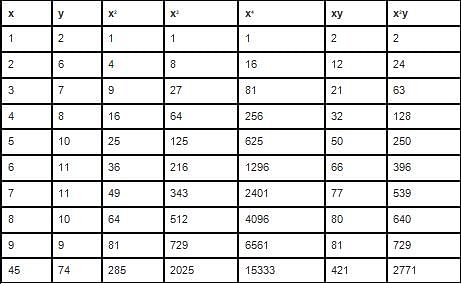
Fit a second degree parabola to the following data.


Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 3
Test: Approximation of Functions using Least Square Method - Question 4
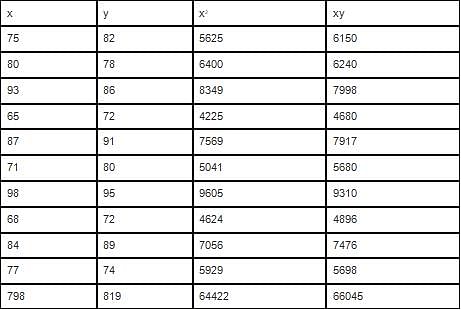
Fit the straight line curve to the following data.
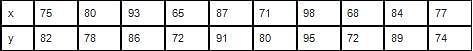
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 4
Test: Approximation of Functions using Least Square Method - Question 5
If the equation y = aebx can be written in linear form Y = A + BX, what are Y, X, A, B?
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 5
Test: Approximation of Functions using Least Square Method - Question 6
The normal equations for a straight line y = ax + b are:
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 6
Test: Approximation of Functions using Least Square Method - Question 7
If the equation y=axb can be written in the linear form Y=A+BX, what are Y, X, A, B?
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 7
Test: Approximation of Functions using Least Square Method - Question 8
If the equation y = abx can be written in linear form Y = A+BX, what are Y, X, A, B?
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 8
Test: Approximation of Functions using Least Square Method - Question 9
The normal equations for a second degree parabola y = ax2 + bx + c are Σy = aΣx2 + bΣx + nc, Σxy = aΣx3 + bΣx2 + cΣx and Σx2y = aΣx4 + bΣx3 + cΣx2.. Is it true or false?
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 9
Test: Approximation of Functions using Least Square Method - Question 10
The parameter E which we use for least square method is called as ______
Detailed Solution for Test: Approximation of Functions using Least Square Method - Question 10
Information about Test: Approximation of Functions using Least Square Method Page
In this test you can find the Exam questions for Test: Approximation of Functions using Least Square Method solved & explained in the simplest way possible.
Besides giving Questions and answers for Test: Approximation of Functions using Least Square Method, EduRev gives you an ample number of Online tests for practice
Download as PDF