BITSAT Mathematics Test - 1 - JEE MCQ
30 Questions MCQ Test - BITSAT Mathematics Test - 1
If P(at2, 2at) be one end of a focal chord of the parabola y2 = 4ax, then the length of the chord is
Two tangents are drawn from a point (-2, -1) to the curve y2 = 4x. If α is the angle between them, then |tanα| is equal to
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If 2x+3y=α, x−y=β and kx+15y=r are 3 concurrent normals of parabola y2=λx, then the value of k is
If three parabolas touch all the lines x=0, y=0 and x+y=2, then the maximum area of the triangle formed by joining their foci is
An equilateral triangle is inscribed in the parabola y2=4 ax, where one vertex is at the vertex of the parabola. Find the length of the side of the triangle.
The length of the latus rectum and equation of the directrix of the parabola y2=−8x
The mirror image of the directrix of the parabola y2=4(x+1) in the line mirror x+2y=3, is
The shortest distance (in units) between the parabolas y2 = 4x and y2=2x−6 is
The sum of 40 terms of an A.P. whose first term is 2 and common difference 4, will be
99th term of the series 2 + 7 + 14 + 23 + 34 +_______ is
If the sum of first n natural numbers is one-fifth of the sum of their squares, then n is
If a polygon has 44 diagonals, then the number of its sides are
If 7 points out of 12 are in same striaght line, then the number of triangles formed is
Sum of coefficients in the expansion of (x + 2y + z)10 is
The probability of getting heads in both trials when a balanced coin is tossed twice, will be
A and B throw 2 dices. If A throws sum of 9, then B's chance of throwing higher sum number is
Two cards are drawn at random from a pack to 52 cards. The probability of these two being aces is
The curve described parametrically by x = t2 + 2t − 1, y = 3t + 5 represents
There were two women participating in a chess tournament. Every participant played two games with the other participants. The number of games that the men played between themselves proved to exceed by 66 the number of games that the men played with the women. The number of participants is
Out of 6 boys and 4 girls, a group of 7 is to be formed. In how many ways can this be done, if the group is to have a majority of boys?
If all the words formed from the letters of the word "HORROR" are arranged in the opposite order as they are in a dictionary, then the rank of the word "HORROR" is
In a football championship, there were played 153 matches. Every team played one match with each other. The number of teams participating in the championship is
Find the maximum number of points of intersection of 8 circles.
Four normal dice are rolled once. The number of possible outcomes in which at least one die shows up 2 is -
How many 5 digit telephone numbers can be constructed using the digits 0 to 9, if each number starts with 67 and no digit appears more than once?
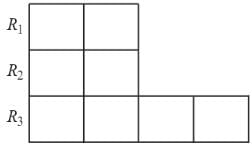
The number of ways in which we can put letters of the word PERSON in the squares of the Fig so that no row remains empty is
The orthocentre of the triangle with vertices (−2,−6), (−2,4) and (1,3) is


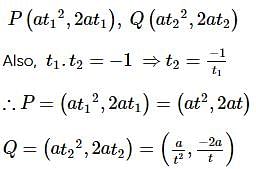
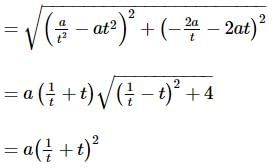
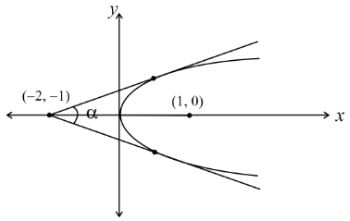
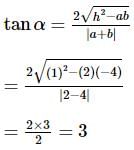
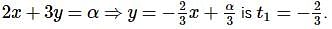
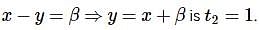
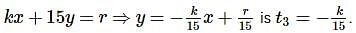
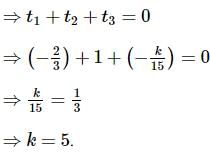
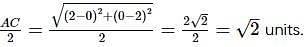
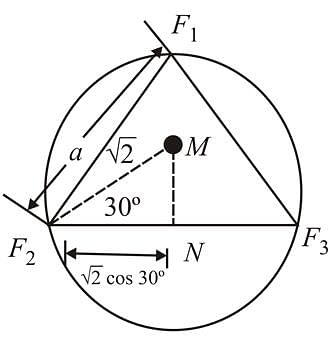
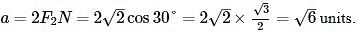
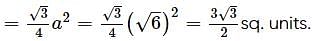
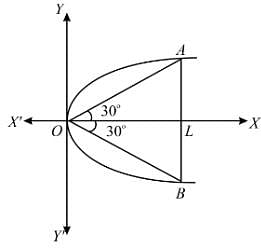
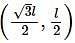
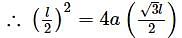
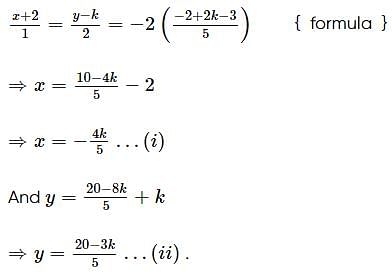
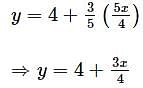
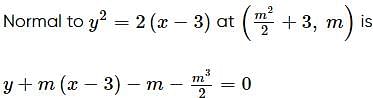
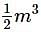
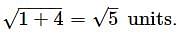
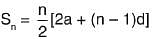
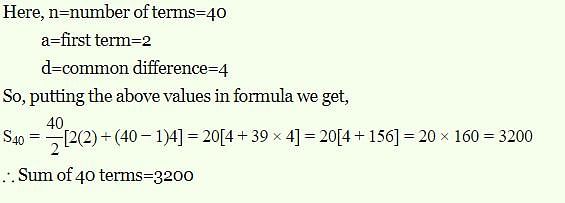

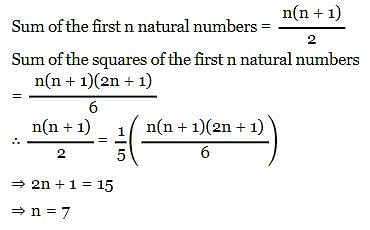
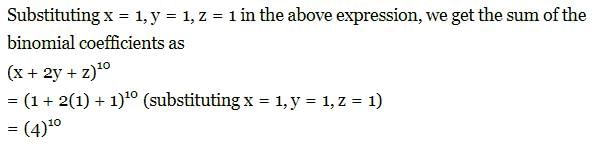
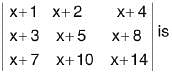
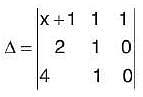
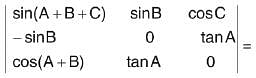
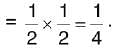
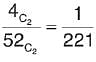
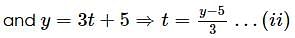
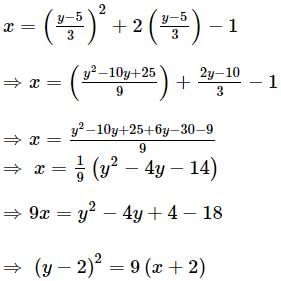
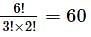
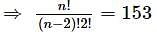
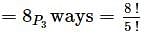
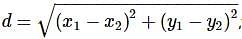 we get
we get