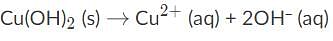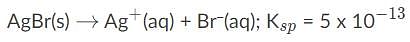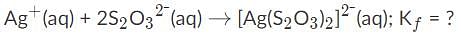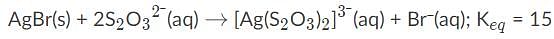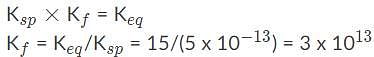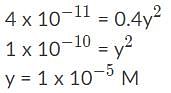Test: Solubility Equilibria - MCAT MCQ
10 Questions MCQ Test - Test: Solubility Equilibria
Which of the following Lewis dot structures of polyatomic ions has or have an overall charge of -2?
I. 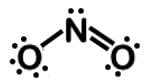
II. 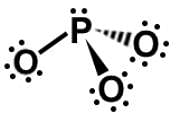
III. 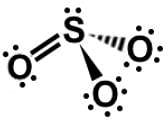
IV. 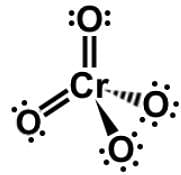
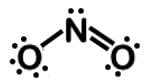
II.

III.

IV.

Based on general solubility rules, which of the following compounds is considered soluble?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Which of the following compounds will not create a precipitate upon addition into a saturated solution of potassium nitrate?
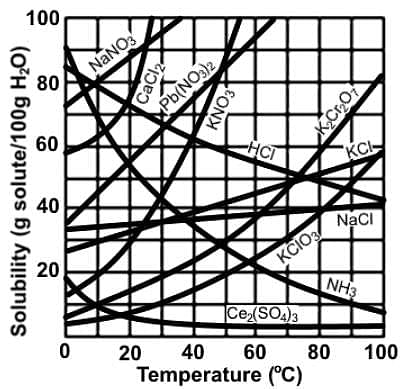
Which of the following statements most accurately describes the data in the following chart depicting solubility of various compounds in water at varying temperatures?
Which of the following conditions will allow cupric hydroxide to dissolve in a saturated solution of Cu(OH)2?
Which of the following statements most accurately describes the solubility product constant?
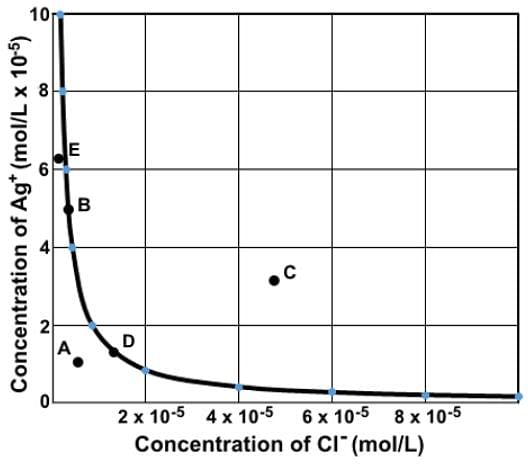
Which of the following statements most accurately describes the data depicted in the graph depicting the concentration of silver ion at varying concentrations of chloride ion in a saturated solution of AgCl? (Ksp of Agcl = 1.8 x 10-10)
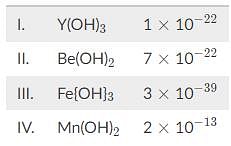
Based on the solubility product constants below, what is the correct order of the following hydroxide solutions in increasing pH?
AgBr is a sparingly soluble salt and has a Ksp value of 5 x 10−13 By adding thiosulfate, we are able to dramatically increase the solubility of AgBr. Given the equation and equilibrium constant below, what is the formation constant (Kf) of the complex ion [Ag(S2O3)2]2-?,
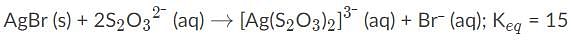
Calcium fluoride (CaF2) has a Ksp value of 4.0 x 10−11. Which of the following statements correctly describes the solubility of CaF2 in a solution containing 0.10 M Ca(NO3)2 versus a solution containing 0.10 M NaF?