Test: Rectangles And Squares- 1 - GRE MCQ
15 Questions MCQ Test - Test: Rectangles And Squares- 1
Rectangular gardens A and B have equal perimeters. If garden A is 10 feet by 14 feet and garden B is 16 feet long, then what is the area (in ft2) of garden B?
Is parallelogram ABCD a square?
(1) All sides of ABCD are equal
(2) Angle ABC = 900
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Area of square ABCD is equal to the area of rectangle PQRS. If the length of rectangle PQRS is 21% more than its breadth, then what is the ratio of perimeter of ABCD to perimeter of PQRS?
What is the area of rectangle ABCD?
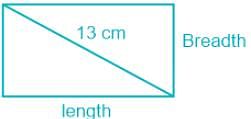
- Length of each diagonal of rectangle ABCD = 13cm
- Perimeter of rectangle ABCD = 34cm
A border of uniform width of ‘w’ inches is placed around a rectangular photograph. What is the area of the border, in square inches?
- w = 2 inches
- Perimeter of rectangular photograph = 20 inches
In the given trapezium, what is the length of the diagonal shown by the dashed line?
The length of a rectangle is 9 cm more than its width, and the area of the rectangle is 486 cm2. What is the length of the diagonal (in cm) of the rectangle?
A jogger runs along the track PABCQRCDASP. How many times will he need to run around this track to cover 5 miles? Note that ABCD is a square.
(1) ∠PSD = ∠QRD = 90° and PA = AB = CQ
(2) PS = QR = BD = 0.35 miles (rounded to nearest tenth)
If the shaded area represents a kids’ playground in a rectangular park, what is the perimeter of the rectangular park?
(1) At a rate of $80 per meter, it costs $1920 to fence the two smaller sides of the kids’ playground.
(2) The area of the kids’ playground is 64 m2.
What is the cost to fence a rectangular plot at the rate of $20/meter?
(1) If length of the plot is increased by 10 meters, the area of the plot increases by 100 sq. m.
(2) If length of the plot is increased by 10% and width of the plot is decreased by 10%, the perimeter decreases by 5%.
ABCD is a rectangle whose longer side is AB. E, F, G and H are the mid-points of the sides AB, BC, CD and DA respectively. The line segment joining E and G intersects with the line segment joining F and H at point P. The perimeter of the rectangle EBFP is 28 units and the area of the triangle AEP is 24 square units. What is the length of each of the smaller sides, AD and BC, of the rectangle ABCD?
A rectangular photograph shown by the white region in the figure is enclosed by a frame of uniform width. The shaded region in the figure represents the frame. What is the ratio of AB to BC?
(1) The ratio of the longer dimension to the smaller dimension of the photograph is 3:2.
(2) If a frame of twice the width was used then the ratio of AB to BC would have been 5:4.
A triangle ABC is placed inside a rectangle PQRS such that the minimum distance of any vertex of the triangle from the rectangle is 2 inches. What is the area of the rectangle PQRS?
(1) Triangle ABC is an equilateral triangle
(2) The area of Triangle ABC is 4√3 square inches and the length of the side AB of the triangle is 50% of the length of side PQ of the rectangle.
The floor of a company's storage room has an area, of 20,000 square feet. If the floor is in the shape of a square, approximately how many feet long is each side?

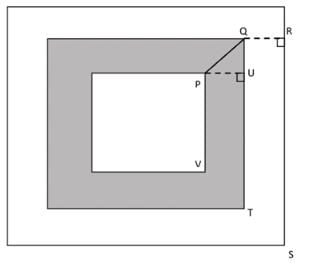
squares are placed one inside another, leaving a strip of uniform width around each square. If
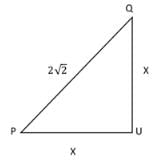
PQ = 2√2 units, QR = 2 units and RS = 10 units, what is the area of the shaded region?