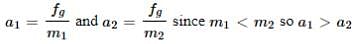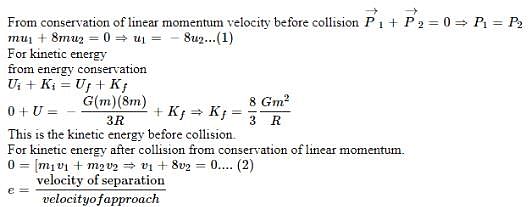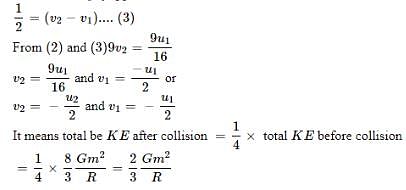Test: Gravitation (October 4) - NEET MCQ
15 Questions MCQ Test - Test: Gravitation (October 4)
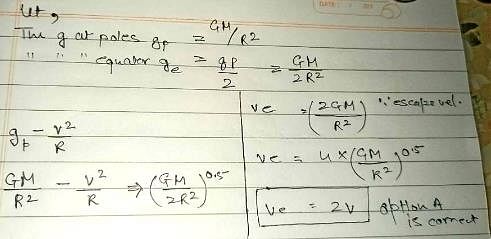
A spherical uniform planet is rotating about its axis. The velocity of a point on its equator is V. Due to the rotation of planet about its axis the acceleration due to gravity g at equator is 1/2 of g at poles. The escape velocity of a particle on the pole of planet in terms of V.
An earth satellite is moved from one stable circular orbit to another larger and stable circular orbit. The following quantities increase for the satellite as a result of this change
For a satellite to orbit around the earth, which of the following must be true ?
The orbit of a planet P round the sun S. AB and CD are the minor and major axes of the ellipse.
If U is the potential energy and K kinetic energy then |U| > |K| at
Two masses m1 and m2 (m1 < m2) are released from rest from a finite distance. They start under their mutual gravitational attraction
The orbit of a planet P round the sun S. AB and CD are the minor and major axes of the ellipse.
If t1 is the time taken by the planet to travel along ACB and t2 the time along BDA, then
When a satellite in a circular orbit around the earth enters the atmospheric region, it encounters small air resistance to its motion. Then
A satellite S is moving in an elliptical orbit around the earth. The mass of the satellite is very small compared to the mass of the earth
Two masses m1 & m2 are initially at rest and are separated by a very large distance. If the masses approach each other subsequently, due to gravitational attraction between them, their relative velocity of approach at a separation distance of d is
A satellite of mass m, initially at rest on the earth, is launched into a circular orbit at a height equal to the radius of the earth. The minimum energy required is
Figure shows the variation of energy with the orbit radius r of a satellite in a circular motion. Select the correct statement.
Two uniform spherical stars made of same material have radii R and 2R. Mass of the smaller planet is m. They start moving from rest towards each other from a large distance under mutual force of gravity. The collision between the stars is inelastic with coefficient of restitution 1/2.
Kinetic energy of the system just after the collision is
A mass is at the center of a square, with four masses at the corners as shown.
(A) (B)
(C) (D)
Rank the choices according to the magnitude of the gravitational force on the center mass.
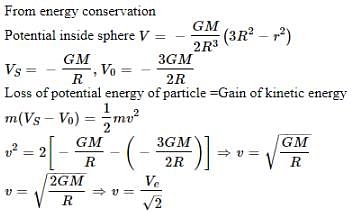
The escape velocity for a planet is ve. A tunnel is dug along a diameter of the planet and a small body is dropped into it at the surface. When the body reaches the centre of the planet, its speed will be