All Courses
Get the App Signup / Login
Sign in Open App
Grade 9 Exam  >  Grade 9 Tests  >  Test: Congruence Criteria- SAS And ASA - Grade 9 MCQ

Test: Congruence Criteria- SAS And ASA - Grade 9 MCQ


Test Description

15 Questions MCQ Test - Test: Congruence Criteria- SAS And ASA

Test: Congruence Criteria- SAS And ASA for Grade 9 2024 is part of Grade 9 preparation. The Test: Congruence Criteria- SAS And ASA questions and answers have been prepared according to the Grade 9 exam syllabus.The Test: Congruence Criteria- SAS And ASA MCQs are made for Grade 9 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Congruence Criteria- SAS And ASA below.
Solutions of Test: Congruence Criteria- SAS And ASA questions in English are available as part of our course for Grade 9 & Test: Congruence Criteria- SAS And ASA solutions in Hindi for Grade 9 course. Download more important topics, notes, lectures and mock test series for Grade 9 Exam by signing up for free. Attempt Test: Congruence Criteria- SAS And ASA | 15 questions in 15 minutes | Mock test for Grade 9 preparation | Free important questions MCQ to study for Grade 9 Exam | Download free PDF with solutions
Test: Congruence Criteria- SAS And ASA - Question 1
Save

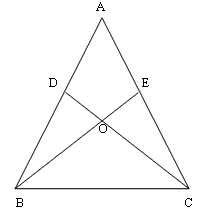
In the given figure, AB = AC, AD = AE = 5 cm and DC = 8 cm. Length of EB is______.

Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 1

8 cm is the answer because DC = EB = 8 cm.

Test: Congruence Criteria- SAS And ASA - Question 2
Save

Triangle ABC is congruent to triangle DEF. Which side is congruent to side BC?

Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 2

The side BC is congruent to side EF.

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Congruence Criteria- SAS And ASA - Question 3
Save

Which of the following statements is incorrect ?

Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 3

2 rectangles having same area may differ in their lengths. So they will not be congruent

Test: Congruence Criteria- SAS And ASA - Question 4
Save

In the following figure, PQ = PR and SQ = SR, then
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 4

In △PQR and △SRQ,

► PQ = SR(Given)
► QR = QR(Common)
► PR = SQ(Given)

By SSS property:
△PQR≅△SRQ
Therefore, ∠PQS =∠PRS

Test: Congruence Criteria- SAS And ASA - Question 5
Save

If two triangles ABC and PQR are congruent under the correspondence A ↔ P, B ↔ Q and C ↔ R, then symbolically, it is expressed as
Test: Congruence Criteria- SAS And ASA - Question 6
Save

If ΔABC ≌ ΔPQR then, which of the following is true?
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 6

Corresponding sides in congruent triangles are equal.

So AC = PR ,AB = PQ ,BC = QR

Test: Congruence Criteria- SAS And ASA - Question 7
Save

If two sides of a triangle are equal, the angles opposite to these sides are______.
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 7

Theorem: Angles opposite to equal sides of an isosceles triangle are equal.

Test: Congruence Criteria- SAS And ASA - Question 8
Save

In case of two equilateral triangles, PQR and STU which of the following correspondence is not correct?
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 8

The correct option is Option A.

All equilateral triangles have the same angles by the congruence rule (SSS)

So, TTS <—> PQR

Test: Congruence Criteria- SAS And ASA - Question 9
Save

In quadrilateral ADBC, AB bisects ∠A. Which of the following criterion will prove ΔABC ≅ ΔABD?
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 9

In quadrilateral ADBC we have:
► AC = AD
and AB being the bisector of ∠A.

Now, in ΔABC and ΔABD:
► AC = AD     [Given]
► AB = AB     [Common]
► ∠CAB = ∠DAB [∴ AB bisects ∠CAD]

∴ Using SAS criteria, we have
ΔABC ≌ ΔABD.

∴ Corresponding parts of congruent triangles (c.p.c.t) are equal.
∴ BC = BD

Test: Congruence Criteria- SAS And ASA - Question 10
Save

In an isosceles triangle ABC with AB = AC, if BD and CE are the altitudes, then BD and CE are______.
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 10

Given: △ABC, AB=AC, BD⊥AC and CE⊥AB
Area of triangle = 1/2 × base × height
Area of △ABC = 1/2 × ​AB × CE = 1/2 × ​BD × AC
CE = BD (Since, AB=AC)

Test: Congruence Criteria- SAS And ASA - Question 11
Save

In fig., if AB = AC and PB = QC, then by which congruence criterion PBC ≅ QCB
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 11

As AB = AC so angle ACB = angle(ABC) as angles opposite to equal sides r equal.

In triangle PBC and Triangle QCB we see that:
i) PB = QC (given)
ii) angle(PBC) = (angle)QCB (proved earliar)
iii) BC = BC (common)

So, triangle PBC is congruent to triangle QCB by SAS axiom of congruency.

Test: Congruence Criteria- SAS And ASA - Question 12
Save

The diagonal PR of a quadrilateral PQRS bisects the angles P and R, then
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 12

In Δ PSR and ΔPQR

► PR = PR
► ∠ 1 = ∠ 2 
► ∠ 3 = ∠ 4

Δ PSR ≅ Δ PQR  [ASA]

► PS = PQ   [CPCT]
► QR = RS  [CPCT]

The correct option is Option A

Test: Congruence Criteria- SAS And ASA - Question 13
Save

In the given figure, AB = EF, BC = DE, AB ⊥ BD and EF ⊥ CE. Which of the following criterion is true for ΔABD ≅ ΔEFC?
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 13

In triangle ABD and FEC:
► AB = FE ( given )
► ∠FEC = ∠ABD ( 90degree)
► BC = DE
CD is common part coming in both triangles.
► BC + CD = CD + DE
► BD = CE
Therefore, triangle ABD is congruent to triangle FEC by SAS rule of congruence.

Test: Congruence Criteria- SAS And ASA - Question 14
Save

Two figures are congruent if they have______.
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 14

In geometry, two figures or objects are congruent if they have the same shape and size, or if one has the same shape and size as the mirror image of the other.

Test: Congruence Criteria- SAS And ASA - Question 15
Save

A triangle can have______.
Detailed Solution for Test: Congruence Criteria- SAS And ASA - Question 15

The least number of acute angles that a triangle can have is 2.

As we cannot have more than one right angle or obtuse angle, we have only two or three acute angles in a triangle.

Further, if one angle is acute, sum of other two angles is more than 900 and we cannot have two right angles or obtuse angles.

Information about Test: Congruence Criteria- SAS And ASA Page
In this test you can find the Exam questions for Test: Congruence Criteria- SAS And ASA solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Congruence Criteria- SAS And ASA, EduRev gives you an ample number of Online tests for practice

Top Courses for Grade 9

Download as PDF

Top Courses for Grade 9

Important Questions for Congruence Criteria- SAS And ASA

Find all the important questions for Congruence Criteria- SAS And ASA at EduRev.Get fully prepared for Congruence Criteria- SAS And ASA with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Congruence Criteria- SAS And ASA syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Congruence Criteria- SAS And ASA resources.

Congruence Criteria- SAS And ASA MCQs with Answers

Prepare for the Congruence Criteria- SAS And ASA within the Grade 9 exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Congruence Criteria- SAS And ASA

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Congruence Criteria- SAS And ASA with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup