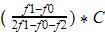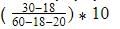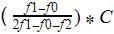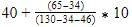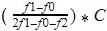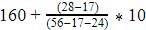Test: Relation between Central Tendencies - Class 10 MCQ
10 Questions MCQ Test - Test: Relation between Central Tendencies
The mean and median of same data are 24 and 26 respectively. The value of mode is :
The mode, median and mean of the following data are:
If median of 20 observations is 50 and mode is also 50, then the mean is
For a symmetrical distribution, which is correct
The median of the given data is 46 and the total number of items is 230.
Using the formula Mode = 3 Median – 2 Mean, the mean will be
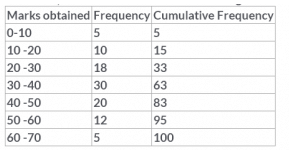
For the following frequency distribution:
If the mode and the median are 12.9 and 14.44 respectively, then the mean is
A student noted the number of cars passing through a spot on a road for 100 periods each of 3 minutes and summarized it in the table given below
The mode, median and mean of the data is
If the median of the following data is 166.79, then the mean and mode are
The mean and the median of a distribution are 45.9 and 46 respectively. The mode will be
Which of the following is not a measure of central tendency?