CSIR NET Physical Science Mock Test - 5 - UGC NET MCQ
30 Questions MCQ Test - CSIR NET Physical Science Mock Test - 5
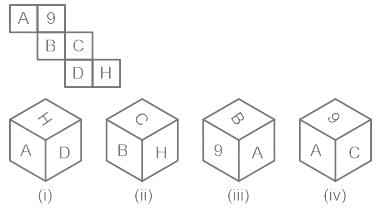
By folding the given paper, which of the following cube cannot be made?

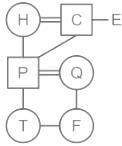
T is the daughter of P, who is the son of H. H is married to C. F is the daughter of Q, who is married to P. C is the brother of E. How is C related to T?
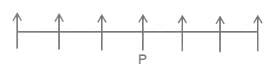
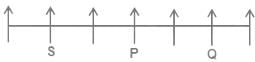
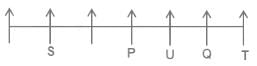
Seven persons – P, Q, R, S, T, U and V are sitting in a straight line facing north, but not necessarily in the same order. P is sitting at the centre of the row. Between P and Q only one person sits. S sits second from the left corner. Between U and T only one person sits and T at the right corner. R does not sit in any corner. Who is sitting between P and Q?
A house has a number which needs to be identified. The following three statements are given that can help in identifying the house number.
- If the house number is a multiple of 3, then it is a number from 50 to 59.
- If the house number is NOT a multiple of 4, then it is a number from 60 to 69.
- If the house number is NOT a multiple of 6, then it is a number from 70 to 79.
What is the house number?
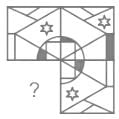
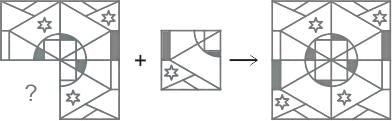
Select the figure from the options that can replace the question mark(?) and complete the given pattern.
The police arrested four criminals - P, Q, R and S. The criminals knew each other. They made the following statements:
P says “Q committed the crime.”
Q says “S committed the crime.
R says “I did not do it.”
S says “What Q said about me is false.”
Assume only one of the arrested four committed the crime and only one of the statements made above is true. Who committed the crime?
The probability of having 53 Tuesdays in an ordinary year is:
Amulya and Amar visited two places A and B respectively in Kashmir and recorded the minimum temperatures on a particular day as –4°C at A and –1°C at B. Which of the following statement is true?
If the compound interest on a certain sum of money for 2 years at 4% per annum is Rs. 3264, then what would be the simple interest on the same sum for 2 years at the same rate?
Pipe A and pipe B can fill the tank completely in 20 hours and 30 hours respectively. Pipe C can empty the completely filled tank in 24 hours. If pipe A and pipe B are opened together and after 10 hours pipe B is closed and pipe C is opened. Find the total time required to fill the tank completely. (in hours)
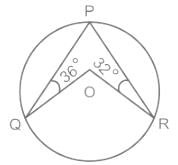
In the given figure O is the center of the circle.∠PQO = 36° and ∠PRO = 32°
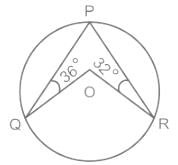
Find the value of ∠QOR.
If x is real then find the minimum value of (x + 4)(x + 3)
The following line graph shows the number of bikes sold by Bikash Motors in the last month.
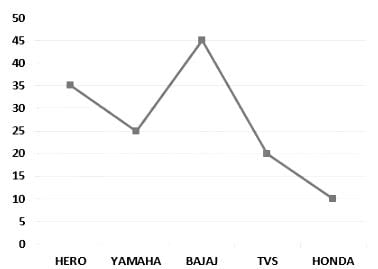
The number of Yamaha bikes sold is what percent of the total sales?
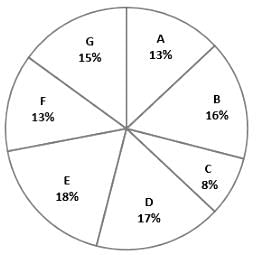
The population of 7 villages is shown in the following pie chart.
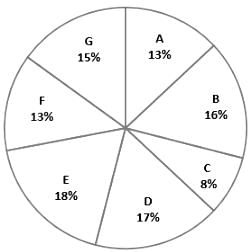
If the total population of all the villages is 60000, what is the population of village G?
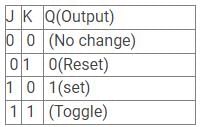
A Counter Consists of Four Flip-Flops
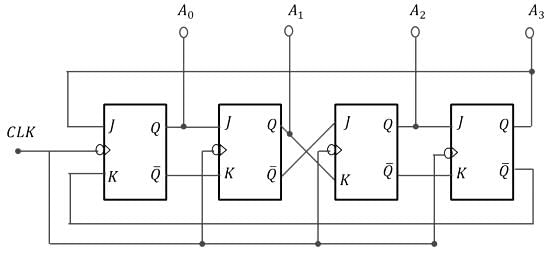
It the counter is initialized as A0A1A2A3=0110 , the state after the next clock pulse is
The Van der Waals' equation of state for a gas is given by,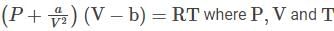 represent the pressure, volume V and temperature respectively, and a and b are constant parameters. At the critical point, where all the roots of the above cubic equation are degenerate, the volume is given by-
represent the pressure, volume V and temperature respectively, and a and b are constant parameters. At the critical point, where all the roots of the above cubic equation are degenerate, the volume is given by-
The equation of motion of a system described by the time dependent Lagrangian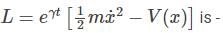
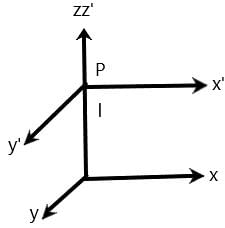
In the rest frame S1 of a point particle with electric charge q1, another point particle with electric
charge q2 moves with a speed v parallel to the x -axis at a perpendicular distance l. The magnitude of
the electromagnetic force felt by q1 due to q2 when the distance between them is minimum, is
[In the following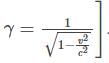
The Hamiltonian of a two-level quantum system is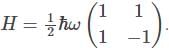 A possible initial state in which the probability of the system being in that quantum state does not change with time, is
A possible initial state in which the probability of the system being in that quantum state does not change with time, is
The magnetization M of a ferro-magnet, as a function of the temperature T and the magnetic field H, is described by the equation 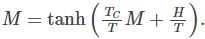 In these units, the zero − field magnetic susceptibility in terms of
In these units, the zero − field magnetic susceptibility in terms of 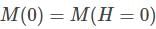
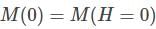 is given by,
is given by,
Consider black body radiation contained in a cavity whose walls are at temperature T. The radiation is in equilibrium with the walls of the cavity. If the temperature of the walls is increased to 2T and the radiation is allowed to come to equilibrium at the new temperature, the entropy of the radiation increases by a factor of:
A matter wave is represented by the wave function: 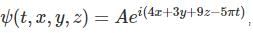 where A is a constant. The direction of wave propagation is given by?
where A is a constant. The direction of wave propagation is given by?
A one-dimensional simple harmonic oscillator with Hamiltonian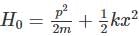 is subjected to small perturbation
is subjected to small perturbation  The first order correction to the ground state energy is dependent on
The first order correction to the ground state energy is dependent on
A walker travels along a one dimensional discrete lattice, labeled by points –N, –N + 1, ...0, ..., N – 1, N, by putting random left and/or right steps of length l with equal probability for every step. Suppose the random walker starts from the lattice position 0 and is found at the same lattice position after (i) 10 and (ii) 7 step walks. The corresponding probabilities are respectively given by
Consider the following probability density function :
P(x) = 0 x < –2 and x > 3
P(x) = a –2 < x < 2
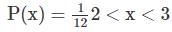
The value of a is :
The mean kinetic energy per atom in a sodium vapor lamp is 0.8 eV. Given that the ratio of the Doppler width of an optical line to its central frequency is 4 X 10-8. Find the approximate mass of the sodium atom?
The electrical conductivity of cooper is approximately 90 % of the electrical conductivity of silver, while the electron density in the silver is approximately 50 % of the electron density in cooper. In the Drude's model, the approximate ratio τCu/τAg of the mean collision time in cooper to mean collision time in silver is?
A particle in 1-D moves under the influence of a potential of V(x) a x4, where a is a real constant. For large n the quantized energy En depends on n as:
Diffuse hydrogen gas within a galaxy may be assumed to follow a Maxwell distribution at temperature 106 K, while the temperature appropriate for the H gas in the inter-galactic space, following the same distribution, may be taken to be 104 K. The ratio of thermal broadening ΔvG / ΔvIG of the Lyman-α line from the H- atoms within the galaxy to that from the inter-galactic space is closest to


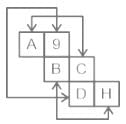
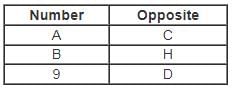
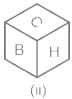 has opposite pair B and H together → This cube can not be made.
has opposite pair B and H together → This cube can not be made. has opposite pair A and C together → This cube can not be made.
has opposite pair A and C together → This cube can not be made.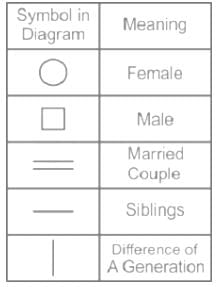



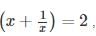 then
then 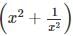 is equal to-
is equal to-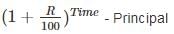
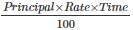
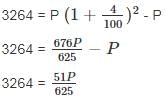
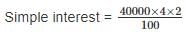
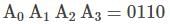
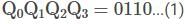
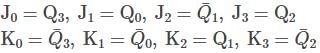
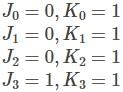
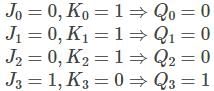
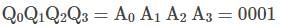
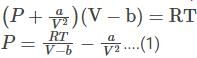
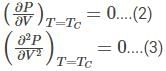
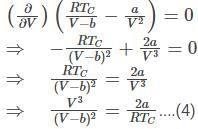
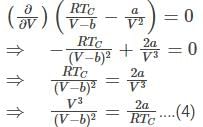
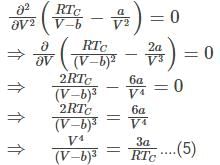
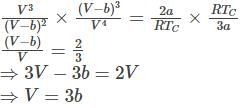
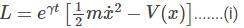
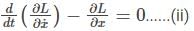
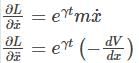
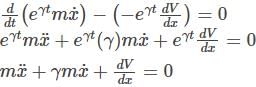
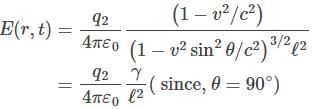
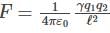
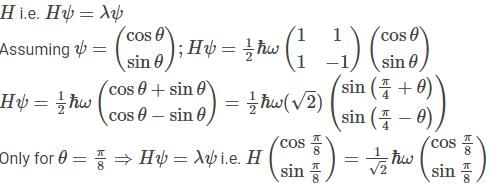
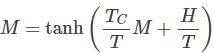
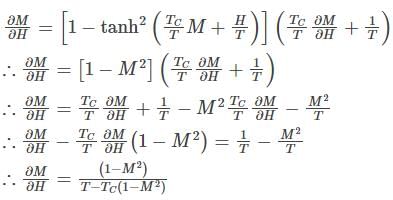
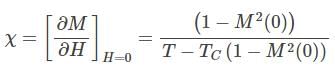
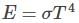
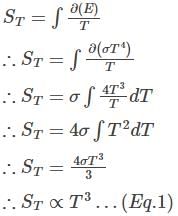
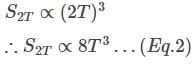
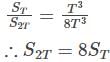
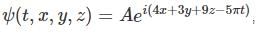 we have:
we have: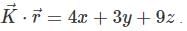

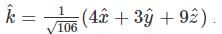
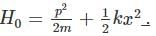
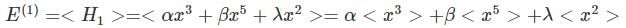
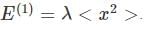
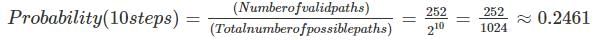
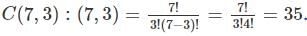
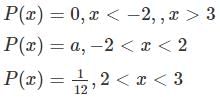
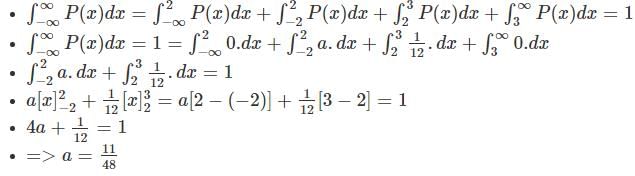
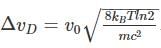
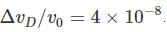
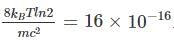
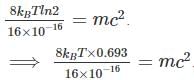
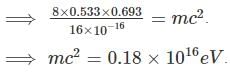
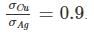
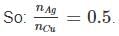
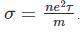
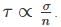
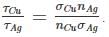
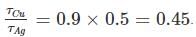
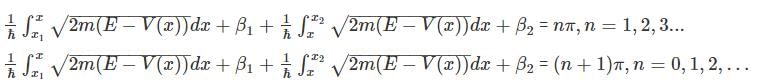
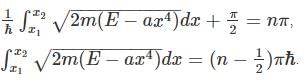
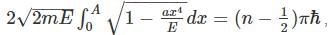 where A is some constant
where A is some constant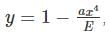 we get:
we get: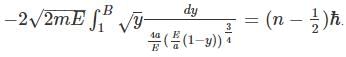
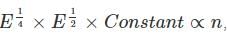 This constant carries a constant after doing the integration.
This constant carries a constant after doing the integration.











