KEAM Paper 2 Mock Test - 5 - JEE MCQ
Test Description
13 Questions MCQ Test - KEAM Paper 2 Mock Test - 5
KEAM Paper 2 Mock Test - 5 for JEE 2024 is part of JEE preparation. The KEAM Paper 2 Mock Test - 5 questions and answers have been prepared
according to the JEE exam syllabus.The KEAM Paper 2 Mock Test - 5 MCQs are made for JEE 2024 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for KEAM Paper 2 Mock Test - 5 below.
Solutions of KEAM Paper 2 Mock Test - 5 questions in English are available as part of our course for JEE & KEAM Paper 2 Mock Test - 5 solutions in
Hindi for JEE course.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt KEAM Paper 2 Mock Test - 5 | 13 questions in 180 minutes | Mock test for JEE preparation | Free important questions MCQ to study for JEE Exam | Download free PDF with solutions
KEAM Paper 2 Mock Test - 5 - Question 1
If one root of the equation ax2 + bx + c = 0 be n times the other root, then
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 1
KEAM Paper 2 Mock Test - 5 - Question 2
Let n(U) = 700, n(A) = 200, n(B) = 300 and n(A ∩ B) = 100,
Then n(Ac∩Bc) =
Then n(Ac∩Bc) =
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 3
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 4
KEAM Paper 2 Mock Test - 5 - Question 5
In a ΔABC, a = 13 cm, b = 12 cm and c = 5 cm. The distance of A from BC is
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 5
KEAM Paper 2 Mock Test - 5 - Question 6
The lengths of sides of a triangle are in the ratio 5 : 12 : 13 and its area is 270 cm2. The respective lengths of sides of the triangle (in cm) are
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 6
KEAM Paper 2 Mock Test - 5 - Question 7
If the mean of a binomial distribution is 25, then its standard deviation lies in the interval
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 7
KEAM Paper 2 Mock Test - 5 - Question 8
The solution of the equation x2 dy/dx = x2 + xy + y2 is
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 8
KEAM Paper 2 Mock Test - 5 - Question 9
Assuming that f is continuous everywhere, (1/c)  is equal to
is equal to
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 9
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 10
KEAM Paper 2 Mock Test - 5 - Question 11
The equation of the normal to the ellipse x2/a2 + y2/b2 = 1 at the positive end of the latus rectum is
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 11
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 12
Detailed Solution for KEAM Paper 2 Mock Test - 5 - Question 13
Information about KEAM Paper 2 Mock Test - 5 Page
In this test you can find the Exam questions for KEAM Paper 2 Mock Test - 5 solved & explained in the simplest way possible.
Besides giving Questions and answers for KEAM Paper 2 Mock Test - 5, EduRev gives you an ample number of Online tests for practice
Download as PDF














 is equal to
is equal to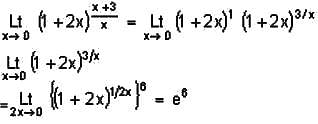
 then f(2x) − f(x) is not divisible by
then f(2x) − f(x) is not divisible by















